Counting Atoms In One Gram Answer Key
Muz Play
Apr 03, 2025 · 6 min read
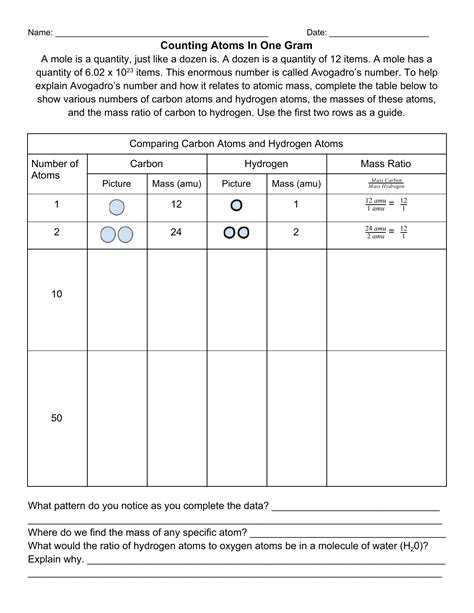
Table of Contents
Counting Atoms in One Gram: A Comprehensive Guide
Determining the number of atoms in a single gram of any element is a fundamental concept in chemistry, bridging the macroscopic world of grams and moles with the microscopic world of individual atoms. This process involves understanding several key concepts, including molar mass, Avogadro's number, and the relationship between mass and the number of particles. This article provides a detailed explanation, working through example problems, and exploring the broader implications of this calculation.
Understanding the Fundamentals: Moles, Molar Mass, and Avogadro's Number
Before we delve into calculating the number of atoms in a gram, let's solidify our understanding of the foundational concepts:
1. The Mole (mol):
The mole is the cornerstone of chemical calculations. It's defined as the amount of a substance containing the same number of elementary entities (atoms, molecules, ions, etc.) as there are atoms in 12 grams of carbon-12. This number is incredibly large: approximately 6.022 x 10<sup>23</sup>. This monumental number is known as Avogadro's number (N<sub>A</sub>).
2. Molar Mass (g/mol):
The molar mass of an element is the mass of one mole of that element, expressed in grams per mole (g/mol). It's numerically equal to the atomic weight of the element found on the periodic table. For example, the atomic weight of carbon (C) is approximately 12.01, so its molar mass is 12.01 g/mol. This means that one mole of carbon atoms has a mass of 12.01 grams.
3. Avogadro's Number (N<sub>A</sub>):
As mentioned earlier, Avogadro's number (N<sub>A</sub>) is approximately 6.022 x 10<sup>23</sup>. This represents the number of atoms, molecules, or ions present in one mole of any substance. It's a crucial conversion factor that allows us to move between the macroscopic world (grams) and the microscopic world (number of atoms).
Calculating the Number of Atoms in One Gram: A Step-by-Step Approach
Let's illustrate the process with a few examples. We'll systematically break down the calculation, highlighting the crucial steps and underlying principles.
Example 1: Calculating the Number of Atoms in 1 gram of Carbon (C)
-
Find the Molar Mass: The molar mass of carbon (C) is approximately 12.01 g/mol (from the periodic table).
-
Convert Grams to Moles: We have 1 gram of carbon. To convert this to moles, we use the molar mass as a conversion factor:
(1 g C) * (1 mol C / 12.01 g C) = 0.0833 mol C
-
Convert Moles to Atoms: Now, we use Avogadro's number to convert moles to the number of atoms:
(0.0833 mol C) * (6.022 x 10<sup>23</sup> atoms C / 1 mol C) ≈ 5.01 x 10<sup>22</sup> atoms C
Therefore, there are approximately 5.01 x 10<sup>22</sup> atoms in 1 gram of carbon.
Example 2: Calculating the Number of Atoms in 1 gram of Gold (Au)
-
Find the Molar Mass: The molar mass of gold (Au) is approximately 196.97 g/mol.
-
Convert Grams to Moles:
(1 g Au) * (1 mol Au / 196.97 g Au) ≈ 0.00507 mol Au
-
Convert Moles to Atoms:
(0.00507 mol Au) * (6.022 x 10<sup>23</sup> atoms Au / 1 mol Au) ≈ 3.05 x 10<sup>21</sup> atoms Au
Therefore, there are approximately 3.05 x 10<sup>21</sup> atoms in 1 gram of gold.
Example 3: A More Complex Example – Considering Isotopes
Most elements exist as a mixture of isotopes, each with a slightly different mass. The atomic weight on the periodic table is a weighted average of the masses of these isotopes. Let's consider chlorine (Cl), which has two major isotopes: <sup>35</sup>Cl and <sup>37</sup>Cl. While the calculation will be more complex, the underlying principle remains the same. We will need the natural abundance of each isotope to obtain a precise answer. Let's assume the following abundances:
- <sup>35</sup>Cl: 75.77%
- <sup>37</sup>Cl: 24.23%
The weighted average molar mass of Chlorine will be:
(0.7577 * 35 g/mol) + (0.2423 * 37 g/mol) ≈ 35.45 g/mol
Now we can proceed as before:
-
Convert Grams to Moles:
(1 g Cl) * (1 mol Cl / 35.45 g Cl) ≈ 0.0282 mol Cl
-
Convert Moles to Atoms:
(0.0282 mol Cl) * (6.022 x 10<sup>23</sup> atoms Cl / 1 mol Cl) ≈ 1.70 x 10<sup>22</sup> atoms Cl
Therefore, considering the isotopic abundances, there are approximately 1.70 x 10<sup>22</sup> atoms in 1 gram of chlorine.
Implications and Applications
The ability to count atoms in a given mass is not just a theoretical exercise; it has significant implications across various scientific fields:
- Chemistry: It's fundamental to stoichiometry, allowing us to calculate reactant and product amounts in chemical reactions.
- Material Science: Understanding the number of atoms allows for precise control over material properties at the nanoscale.
- Nuclear Physics: Calculations involving radioactive decay and nuclear reactions rely heavily on knowing the number of atoms.
- Pharmaceutical Science: Accurate dosages in drug formulation are based on the number of molecules (and therefore atoms) involved.
- Environmental Science: Monitoring pollutant concentrations and their impact often involves quantifying the number of atoms or molecules present.
Beyond Atoms: Extending the Calculation to Molecules
The principles outlined above can be extended to calculate the number of molecules in a given mass of a compound. The key difference is that we use the molar mass of the compound instead of the atomic mass of the element.
For example, let's calculate the number of water (H<sub>2</sub>O) molecules in 1 gram of water.
-
Calculate the Molar Mass of H<sub>2</sub>O: The molar mass of water is approximately 18.02 g/mol (2 x 1.01 g/mol for hydrogen + 16.00 g/mol for oxygen).
-
Convert Grams to Moles:
(1 g H<sub>2</sub>O) * (1 mol H<sub>2</sub>O / 18.02 g H<sub>2</sub>O) ≈ 0.0555 mol H<sub>2</sub>O
-
Convert Moles to Molecules:
(0.0555 mol H<sub>2</sub>O) * (6.022 x 10<sup>23</sup> molecules H<sub>2</sub>O / 1 mol H<sub>2</sub>O) ≈ 3.34 x 10<sup>22</sup> molecules H<sub>2</sub>O
Therefore, there are approximately 3.34 x 10<sup>22</sup> molecules of water in 1 gram of water.
Conclusion
Counting atoms in a gram of a substance is a powerful technique that connects the macroscopic world of observable quantities with the microscopic world of atoms and molecules. By understanding molar mass, Avogadro's number, and the relationship between mass and the number of particles, we can perform these calculations accurately and apply them to a wide range of scientific problems. This fundamental concept is critical for understanding chemical reactions, material properties, and numerous other aspects of the physical world. The examples and explanations provided in this article offer a comprehensive guide to mastering this essential skill in chemistry and related fields. Remember to always refer to the most up-to-date periodic table for the most accurate atomic weights.
Latest Posts
Latest Posts
-
The Spleen Is Located In Which Of The Abdominopelvic Quadrants
Apr 04, 2025
-
Physical And Chemical Properties And Changes Answers
Apr 04, 2025
-
Which Process Of Cellular Respiration Produces The Most Atp
Apr 04, 2025
-
How To Fill In An Anova Table
Apr 04, 2025
-
What Are The Basic Building Blocks Of Nucleic Acids
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Counting Atoms In One Gram Answer Key . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
