Differential Rate Law Vs Integrated Rate Law
Muz Play
Apr 06, 2025 · 6 min read
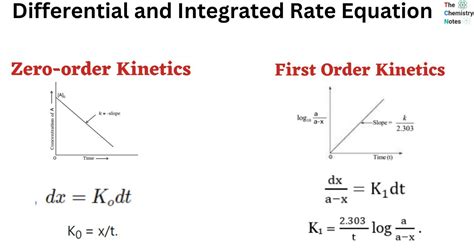
Table of Contents
Differential Rate Law vs. Integrated Rate Law: A Comprehensive Guide
Understanding reaction rates is fundamental in chemistry. This understanding hinges on grasping the difference between two crucial concepts: the differential rate law and the integrated rate law. While both describe the speed of a reaction, they do so in distinct ways, providing complementary insights into reaction kinetics. This comprehensive guide will delve into the intricacies of each, highlighting their differences, applications, and the essential connections between them.
What is a Rate Law?
Before diving into the specifics of differential and integrated rate laws, let's establish a common foundation. A rate law, also known as a rate equation, is a mathematical expression that describes the relationship between the rate of a chemical reaction and the concentrations of the reactants. It's an empirical equation determined experimentally, not derived theoretically from the stoichiometry of the reaction. The rate law generally takes the form:
Rate = k[A]<sup>m</sup>[B]<sup>n</sup>...
Where:
- Rate: Represents the speed of the reaction (often expressed as the change in concentration per unit time).
- k: Is the rate constant, a proportionality constant specific to the reaction and temperature. A larger k indicates a faster reaction.
- [A], [B], ...: Represent the concentrations of reactants A, B, and so on.
- m, n, ...: Are the orders of the reaction with respect to each reactant. These are experimentally determined exponents and are not necessarily equal to the stoichiometric coefficients in the balanced chemical equation.
The Differential Rate Law: A Snapshot in Time
The differential rate law, also called the instantaneous rate law, expresses the rate of reaction at a specific instant in time. It's a differential equation because it describes the rate of change of reactant or product concentrations. The equation is directly derived from the rate law mentioned above:
Rate = -d[A]/dt = k[A]<sup>m</sup>[B]<sup>n</sup>...
Where:
- -d[A]/dt: Represents the instantaneous rate of change in the concentration of reactant A with respect to time. The negative sign indicates that the concentration of reactant A decreases over time.
Key Characteristics of the Differential Rate Law:
- Instantaneous Rate: Provides the reaction rate at a specific point in time.
- Differential Equation: Expresses the rate as a derivative, showing the relationship between the rate and concentration changes.
- Useful for: Determining the reaction order with respect to each reactant through experimental data analysis (e.g., method of initial rates).
- Difficult to integrate directly: Determining the concentration of reactants as a function of time requires integration, leading to the integrated rate law.
Determining Reaction Order using the Differential Rate Law: The Method of Initial Rates
The method of initial rates is a common experimental technique used to determine the reaction order from the differential rate law. By measuring the initial rates of the reaction under varying initial concentrations of reactants, we can deduce the exponents (m, n, etc.) in the rate law.
Let's consider a hypothetical reaction: A + B → Products.
If we observe that doubling the concentration of A doubles the initial rate while keeping the concentration of B constant, this indicates that the reaction is first order with respect to A (m=1). Similarly, if tripling the concentration of B triples the rate while keeping the concentration of A constant, the reaction is first order with respect to B (n=1). This allows us to construct the complete differential rate law:
Rate = k[A][B]
The Integrated Rate Law: Concentration as a Function of Time
The integrated rate law provides a direct relationship between the concentration of a reactant (or product) and time. It's obtained by integrating the differential rate law. The specific form of the integrated rate law depends on the order of the reaction. This makes it incredibly valuable for predicting reactant concentrations at any given time or determining the time required for a certain extent of reaction.
Here's how the integrated rate laws look for different reaction orders:
1. Zero-Order Reactions:
- Differential Rate Law: Rate = k
- Integrated Rate Law: [A]<sub>t</sub> = [A]<sub>0</sub> - kt
- Linear Plot: [A]<sub>t</sub> vs. t (slope = -k, y-intercept = [A]<sub>0</sub>)
2. First-Order Reactions:
- Differential Rate Law: Rate = k[A]
- Integrated Rate Law: ln[A]<sub>t</sub> = ln[A]<sub>0</sub> - kt or [A]<sub>t</sub> = [A]<sub>0</sub>e<sup>-kt</sup>
- Linear Plot: ln[A]<sub>t</sub> vs. t (slope = -k, y-intercept = ln[A]<sub>0</sub>)
- Half-life: t<sub>1/2</sub> = 0.693/k (independent of initial concentration)
3. Second-Order Reactions (with respect to a single reactant):
- Differential Rate Law: Rate = k[A]²
- Integrated Rate Law: 1/[A]<sub>t</sub> = 1/[A]<sub>0</sub> + kt
- Linear Plot: 1/[A]<sub>t</sub> vs. t (slope = k, y-intercept = 1/[A]<sub>0</sub>)
- Half-life: t<sub>1/2</sub> = 1/(k[A]<sub>0</sub>) (dependent on initial concentration)
Key Characteristics of the Integrated Rate Law:
- Concentration vs. Time: Shows the direct relationship between reactant concentration and time.
- Predictive Power: Allows for prediction of concentrations at various times or time required to reach specific concentrations.
- Graphical Analysis: Linear plots of the integrated rate law help determine the reaction order and rate constant.
- Half-life Calculations: Provides a convenient way to calculate the half-life of the reaction (time required for half of the reactant to be consumed).
Comparing Differential and Integrated Rate Laws
| Feature | Differential Rate Law | Integrated Rate Law |
|---|---|---|
| Definition | Rate of reaction at a specific instant | Concentration as a function of time |
| Expression | Rate = -d[A]/dt = k[A]<sup>m</sup>[B]<sup>n</sup>... | Varies with reaction order; see examples above |
| Mathematical Form | Differential equation | Algebraic equation |
| Application | Determining reaction order, initial rate studies | Predicting concentrations, determining half-life |
| Graphical Analysis | Not directly used for determining reaction order | Linear plots reveal reaction order and rate constant |
| Direct Measurement | Measures instantaneous rates | Measures concentration changes over time |
Beyond Simple Reactions: Complex Kinetics
The examples above focus on simple reactions with single reactants or reactions where the rate law conveniently matches the stoichiometry. However, many real-world reactions are much more complex:
- Multiple Reactants: Integrated rate laws become more intricate, especially if the reaction order with respect to different reactants is not equal to 1. Numerical methods may be necessary.
- Consecutive Reactions: These involve multiple steps, and understanding the overall rate requires examining individual steps and their respective rate laws.
- Parallel Reactions: The reactant can follow multiple pathways. Analyzing these situations demands advanced kinetic treatments.
- Reversible Reactions: Reactions that proceed in both the forward and reverse directions need consideration of equilibrium conditions and equilibrium constants alongside rate laws.
- Catalysis: The presence of a catalyst dramatically alters the rate law, creating different rate-determining steps and rate constants.
Conclusion: A Powerful Partnership
The differential and integrated rate laws are complementary tools for studying chemical reaction kinetics. The differential rate law offers a snapshot of the reaction's speed at a particular moment, crucial for determining the reaction order. The integrated rate law provides a complete picture of how reactant concentrations change over time, enabling predictions and half-life calculations. While the simple cases are easily solvable, many complex scenarios necessitate more sophisticated techniques, but the fundamental understanding of both approaches is indispensable for anyone working with reaction kinetics. Mastering both allows for a comprehensive and accurate analysis of reaction behavior, a cornerstone of chemical understanding.
Latest Posts
Latest Posts
-
What Is The Law Of Cross Cutting Relationships
Apr 08, 2025
-
What Is The Unit Of Solubility
Apr 08, 2025
-
What Is The Purpose Of The Contractile Vacuole
Apr 08, 2025
-
Chemical Bonding And Molecular Structure Lab
Apr 08, 2025
-
What Is The Cortex In Plants
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Differential Rate Law Vs Integrated Rate Law . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.