Electric Field Due To A Disk Of Charge
Muz Play
Apr 04, 2025 · 6 min read
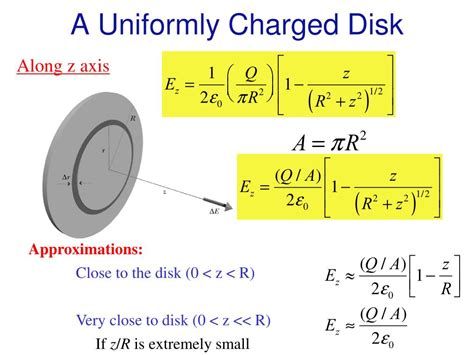
Table of Contents
Electric Field Due to a Disk of Charge: A Comprehensive Guide
The electric field, a fundamental concept in electromagnetism, describes the force exerted on a charged particle at any point in space. Calculating the electric field for various charge distributions is crucial in understanding and applying electromagnetism. One such important distribution is a disk of charge, a continuous distribution of charge spread uniformly over a circular surface. This article provides a comprehensive guide to calculating the electric field due to a disk of charge, exploring various approaches and their implications.
Understanding the Problem: Setting the Stage
Before delving into the calculations, let's clearly define the problem. We're considering a uniformly charged disk with a radius R and a surface charge density σ (sigma), which represents the charge per unit area (Coulombs per square meter). Our goal is to determine the electric field E at a point P located on the axis of the disk, at a distance z from its center. This setup offers a valuable case study for understanding how to integrate over continuous charge distributions.
We'll approach this problem using two primary methods:
- Direct Integration: This involves integrating the contribution of infinitesimally small charge elements across the entire disk. This approach builds an intuitive understanding of the problem.
- Using Gauss's Law: While less intuitive for this specific geometry, Gauss's Law provides a powerful alternative, particularly useful for symmetric charge distributions. However, it's crucial to understand the limitations and why it's not the most straightforward method in this case.
Method 1: Direct Integration - A Step-by-Step Approach
This method involves breaking down the disk into infinitesimal charge elements, calculating the electric field contribution from each element, and then integrating over the entire disk.
1. Defining the Charge Element
Consider a small ring of radius r and width dr on the disk. The area of this ring is dA = 2πr dr. Since the surface charge density is σ, the charge dq on this ring is:
dq = σ dA = σ (2πr dr)
2. Calculating the Electric Field due to the Ring
The electric field dE at point P due to this infinitesimal ring is directed along the axis of the disk. The components perpendicular to the axis cancel out due to symmetry. Using Coulomb's Law, the magnitude of dE is:
dE = (k dq z) / (z² + r²)^(3/2)
where k is Coulomb's constant (8.98755 × 10⁹ N⋅m²/C²).
Substituting the expression for dq:
dE = [k σ (2πr dr) z] / (z² + r²)^(3/2)
3. Integrating over the Entire Disk
To find the total electric field E, we integrate dE over the entire disk, from r = 0 to r = R:
E = ∫ dE = ∫₀ᴿ [k σ (2πr dr) z] / (z² + r²)^(3/2)
This integral can be solved using a simple substitution, u = z² + r², du = 2r dr:
E = k σ π z ∫ (du/u^(3/2))
The limits of integration change accordingly: when r = 0, u = z²; when r = R, u = z² + R².
Solving the integral:
E = k σ π z [-2u^(-1/2)] | (from z² to z²+R²)
E = 2πkσ [1 - z / √(z² + R²)]
This is the final expression for the electric field along the axis of a uniformly charged disk.
4. Analyzing the Result
This equation reveals important insights:
- At the Center of the Disk (z=0): The electric field is zero. This is expected due to symmetry.
- Far from the Disk (z >> R): The term z / √(z² + R²) approaches 1, and the electric field approaches:
E ≈ 2πkσ (1 - 1) = 0
This is because at large distances, the disk appears as a point charge, with a total charge Q = πR²σ.
- Close to the Disk (z << R): The term z / √(z² + R²) approaches 0, and the electric field approaches:
E ≈ 2πkσ
This is the electric field near an infinite plane of charge.
Method 2: Gauss's Law - A Different Perspective
Gauss's Law states that the flux of the electric field through a closed surface is proportional to the enclosed charge. While powerful, its direct application to the disk is less straightforward than the direct integration method. The symmetry isn't ideal for a simple Gaussian surface. The most appropriate Gaussian surface would be a cylinder, but the flux through the curved surface would be complicated to calculate. While possible, it's significantly more complex than the direct integration method in this case.
For simpler scenarios with higher degrees of symmetry (like a uniformly charged sphere), Gauss's law is much more efficient.
Comparing the Methods: Strengths and Weaknesses
| Method | Strengths | Weaknesses |
|---|---|---|
| Direct Integration | Intuitive, provides step-by-step understanding, applicable to various charge distributions | Can be mathematically challenging for complex geometries |
| Gauss's Law | Powerful for highly symmetric charge distributions | Requires careful selection of Gaussian surface; not ideal for this case |
Beyond the Axis: Electric Field at Off-Axis Points
The calculation of the electric field at points off the axis of the disk is considerably more complex. It requires integrating the components of the electric field from each infinitesimal charge element in two dimensions. This frequently involves double integrals and elliptic integrals, making analytical solutions more difficult. Numerical methods, such as finite element analysis or Monte Carlo simulations, are often used for off-axis calculations.
Applications of the Electric Field Due to a Disk of Charge
Understanding the electric field of a charged disk has significant applications in various fields:
- Modeling Capacitor Plates: Parallel plate capacitors are frequently modeled as disks. The calculated field helps determine capacitance and potential difference.
- Particle Accelerators: Charged disks can be used to create uniform electric fields for accelerating charged particles.
- Electrostatic Lenses: In electron microscopy and other applications, carefully shaped electrodes (approximated as disks) form electrostatic lenses to focus electron beams.
- Plasma Physics: Disks of charge can provide a simplified model for understanding plasma behavior in certain scenarios.
Conclusion: A Powerful Tool in Electromagnetism
Calculating the electric field due to a disk of charge is a fundamental problem that showcases the power of calculus and the laws of electromagnetism. While direct integration provides the clearest path to an analytical solution, Gauss's law serves as a powerful tool in other symmetrical situations. This understanding is crucial for tackling more complex problems in electromagnetism and has wide-ranging applications in various scientific and technological domains. Further exploration of this topic could include investigating non-uniform charge distributions on the disk, or examining the electric potential due to the disk, which is a related but distinct calculation. Remember to always consider the geometry and symmetry when choosing the most efficient method for calculating electric fields.
Latest Posts
Latest Posts
-
What Is A Standard Curve In Biology
Apr 10, 2025
-
How Hot Can Hot Plates Get
Apr 10, 2025
-
What States Of Matter Are Compressible
Apr 10, 2025
-
How To Turn Atoms Into Grams
Apr 10, 2025
-
How Do You Find The Roots
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about Electric Field Due To A Disk Of Charge . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.