Find The Limit Of Trigonometric Functions
Muz Play
Apr 06, 2025 · 6 min read
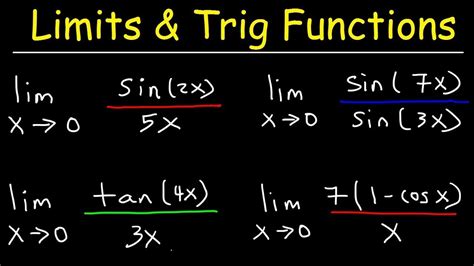
Table of Contents
Finding the Limit of Trigonometric Functions: A Comprehensive Guide
Finding the limit of trigonometric functions is a crucial aspect of calculus, often encountered in various applications. This comprehensive guide delves into the intricacies of evaluating these limits, equipping you with the necessary techniques and understanding to tackle a wide range of problems. We'll explore different approaches, including direct substitution, algebraic manipulation, L'Hôpital's Rule, and the squeeze theorem, providing numerous examples to solidify your grasp of the concepts.
Understanding Limits in Trigonometric Functions
Before diving into specific techniques, it's crucial to understand the fundamental concept of a limit. In the context of trigonometric functions, the limit of a function f(x) as x approaches a value c (written as lim<sub>x→c</sub> f(x)) represents the value that f(x) approaches as x gets arbitrarily close to c, but not necessarily equal to c. This is particularly important when dealing with trigonometric functions, which often have discontinuities or undefined values at specific points.
Key Concepts:
- Continuity: A function is continuous at a point if its limit at that point exists and is equal to the function's value at that point. Many trigonometric functions are continuous throughout their domains.
- Indeterminate Forms: When evaluating limits, you may encounter indeterminate forms like 0/0 or ∞/∞. These forms require further analysis using techniques described later in this article.
- Trigonometric Identities: A deep understanding of trigonometric identities is essential for simplifying expressions and resolving indeterminate forms. Familiarize yourself with identities such as the Pythagorean identities (sin²x + cos²x = 1, etc.), sum-to-product and product-to-sum formulas, and double-angle formulas.
Methods for Evaluating Limits of Trigonometric Functions
Let's examine various methods used to determine the limits of trigonometric functions:
1. Direct Substitution
The simplest method is direct substitution. If the function is continuous at the point c, then the limit is simply the function's value at c:
lim<sub>x→c</sub> f(x) = f(c)
Example:
Find lim<sub>x→π/4</sub> sin(x)
Since sin(x) is continuous at x = π/4, we can directly substitute:
lim<sub>x→π/4</sub> sin(x) = sin(π/4) = √2/2
2. Algebraic Manipulation
Often, algebraic manipulation, including factoring and rationalization, is necessary to simplify the expression before applying direct substitution or other methods. This is particularly useful when dealing with indeterminate forms.
Example:
Find lim<sub>x→0</sub> (sin(x) / x)
This limit is an indeterminate form (0/0). However, using the known limit lim<sub>x→0</sub> (sin(x) / x) = 1, we can directly solve this.
3. L'Hôpital's Rule
L'Hôpital's Rule is a powerful tool for evaluating limits of indeterminate forms (0/0 or ∞/∞). It states that if the limit of f(x)/g(x) as x approaches c is indeterminate, then:
lim<sub>x→c</sub> f(x)/g(x) = lim<sub>x→c</sub> f'(x)/g'(x)
provided the limit on the right-hand side exists.
Example:
Find lim<sub>x→0</sub> (sin(x) / x) using L'Hôpital's Rule.
This is an indeterminate form (0/0). Applying L'Hôpital's rule:
lim<sub>x→0</sub> (sin(x) / x) = lim<sub>x→0</sub> (cos(x) / 1) = cos(0) = 1
4. Squeeze Theorem (Sandwich Theorem)
The Squeeze Theorem states that if f(x) ≤ g(x) ≤ h(x) for all x in an interval around c, and lim<sub>x→c</sub> f(x) = lim<sub>x→c</sub> h(x) = L, then lim<sub>x→c</sub> g(x) = L. This is particularly useful for limits involving trigonometric functions that are difficult to evaluate directly.
Example:
Find lim<sub>x→0</sub> (x²sin(1/x)).
We know that -1 ≤ sin(1/x) ≤ 1 for all x ≠ 0. Therefore, -x² ≤ x²sin(1/x) ≤ x².
Since lim<sub>x→0</sub> (-x²) = 0 and lim<sub>x→0</sub> (x²) = 0, by the Squeeze Theorem, lim<sub>x→0</sub> (x²sin(1/x)) = 0.
Advanced Techniques and Applications
The techniques discussed above form the foundation for evaluating the limits of trigonometric functions. However, more complex scenarios may require a combination of these methods or more advanced calculus concepts.
Limits Involving Multiple Trigonometric Functions
Problems often involve multiple trigonometric functions. In these cases, strategically using trigonometric identities to simplify the expression before applying any of the above methods is crucial.
Example:
Find lim<sub>x→0</sub> [(1 - cos(x))/x²]
This is an indeterminate form (0/0). We can use L'Hôpital's rule or manipulate the expression using trigonometric identities:
Using L'Hôpital's Rule twice:
lim<sub>x→0</sub> [(1 - cos(x))/x²] = lim<sub>x→0</sub> [sin(x)/2x] = lim<sub>x→0</sub> [cos(x)/2] = 1/2
Limits Involving Infinite Sequences
Limits can also be applied to infinite sequences involving trigonometric functions. The techniques are similar, often involving manipulating the expressions using trigonometric identities and then applying limit properties.
Example: Consider the sequence a<sub>n</sub> = sin(nπ/2).
The sequence does not converge to a single value as it alternates between -1, 0, 1, 0. Therefore, the limit does not exist.
Applications in Physics and Engineering
Finding the limits of trigonometric functions has significant applications in various fields, particularly physics and engineering. Examples include:
- Calculating instantaneous velocity and acceleration: The derivative of a position function (often involving trigonometric functions) provides the instantaneous velocity, and the derivative of the velocity function gives the acceleration. Limits are essential for calculating these derivatives.
- Analyzing oscillatory motion: Trigonometric functions are fundamental in modeling oscillatory systems, like simple harmonic motion of a pendulum. Limits help to analyze the behavior of these systems as time approaches specific values.
- Optics and wave phenomena: Limits are crucial in studying wave interference and diffraction patterns, where trigonometric functions describe the wave behavior.
Conclusion
Mastering the techniques for finding the limits of trigonometric functions is essential for success in calculus and its various applications. This guide has provided a comprehensive overview of the key methods, including direct substitution, algebraic manipulation, L'Hôpital's Rule, and the Squeeze Theorem. By understanding these techniques and practicing with numerous examples, you will develop the confidence and skill to tackle a broad range of problems involving limits of trigonometric functions. Remember to always simplify expressions as much as possible using trigonometric identities before attempting to apply limit rules. With consistent practice and a firm grasp of the underlying concepts, you can confidently navigate the world of limits in trigonometric functions.
Latest Posts
Latest Posts
-
What Is A Row In A Periodic Table
Apr 08, 2025
-
Sodium Potassium Pump Is An Example Of
Apr 08, 2025
-
Nations Usually Specialize In Industries Where They Have A
Apr 08, 2025
-
What Is The Characteristics Of Liquid
Apr 08, 2025
-
System Of Equations Examples With Answers
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Find The Limit Of Trigonometric Functions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.