How Do You Divide A Square Root
Muz Play
Mar 28, 2025 · 5 min read
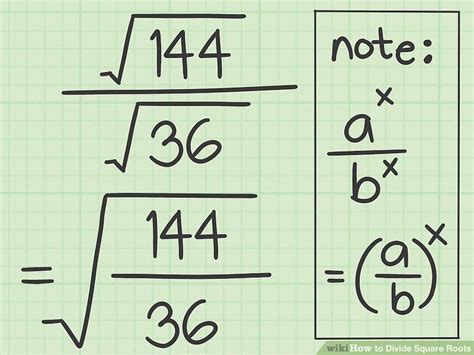
Table of Contents
How Do You Divide a Square Root? A Comprehensive Guide
Dividing square roots might seem daunting at first, but with a clear understanding of the underlying principles, it becomes a straightforward process. This comprehensive guide will delve into the intricacies of dividing square roots, equipping you with the knowledge and techniques to tackle various scenarios with confidence. We'll cover everything from basic division to more complex examples, ensuring you master this essential mathematical skill.
Understanding Square Roots and Their Properties
Before diving into division, let's refresh our understanding of square roots. A square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 9 (√9) is 3, because 3 x 3 = 9.
Key Properties to Remember:
- Product Property: The square root of a product is equal to the product of the square roots. √(ab) = √a * √b
- Quotient Property: This is crucial for division! The square root of a quotient is equal to the quotient of the square roots. √(a/b) = √a / √b
- Simplifying Square Roots: Always simplify square roots by factoring out perfect squares. For example, √12 can be simplified to √(4*3) = √4 * √3 = 2√3.
Dividing Square Roots: The Fundamentals
The simplest form of dividing square roots involves applying the quotient property directly. Let's illustrate this with an example:
Example 1: √16 / √4
Using the quotient property, we can rewrite this as:
√(16/4) = √4 = 2
This demonstrates the fundamental principle: dividing square roots is often as simple as dividing the numbers under the radical sign (the number inside the square root symbol) and then simplifying the resulting square root.
Handling More Complex Divisions
However, not all divisions are as straightforward as the example above. Often, you'll encounter situations where the numbers under the radical signs are not perfectly divisible. Let's explore a more challenging scenario:
Example 2: √27 / √3
Again, we apply the quotient property:
√(27/3) = √9 = 3
Here, the numbers neatly divided, leaving us with a perfect square. But what happens when the division doesn't result in a perfect square?
Dividing Square Roots with Non-Perfect Squares
This is where the process becomes slightly more involved, requiring us to utilize both the quotient property and the simplification of square roots. Let’s consider this example:
Example 3: √18 / √2
Applying the quotient property gives us:
√(18/2) = √9 = 3
This is a relatively simple example. Let's look at a more challenging case:
Example 4: √75 / √3
Using the quotient property:
√(75/3) = √25 = 5
Example 5: √50 / √2
Applying the quotient property:
√(50/2) = √25 = 5
Now, let’s look at an example where the division doesn't result in a perfect square:
Example 6: √24 / √6
Using the quotient property:
√(24/6) = √4 = 2
This example highlights that simplification is key. Even though we initially divided, further simplification was necessary to arrive at the final answer.
Rationalizing the Denominator
A crucial concept when working with square roots is rationalizing the denominator. This means eliminating any square roots from the denominator of a fraction. This is considered good mathematical practice and often simplifies calculations.
Example 7: 1 / √2
To rationalize the denominator, we multiply both the numerator and the denominator by √2:
(1 * √2) / (√2 * √2) = √2 / 2
By multiplying by √2/√2 (which is essentially 1), we haven't changed the value of the fraction but have eliminated the square root from the denominator.
Combining Simplification and Rationalization
Sometimes, we need to combine simplification and rationalization.
Example 8: √6 / √12
First, we simplify the fraction under the radical using the quotient property:
√(6/12) = √(1/2)
Now, we rationalize the denominator:
√(1/2) = √1 / √2 = 1 / √2 = √2 / 2
Dividing Square Roots with Variables
The same principles apply when dealing with square roots containing variables. Remember the rules of exponents.
Example 9: √(x⁶y⁴) / √(x²y²)
Using the quotient property:
√(x⁶y⁴ / x²y²) = √(x⁴y²) = x²y
Example 10: √(a⁸b⁶) / √(a⁴b²)
Using the quotient property:
√(a⁸b⁶ / a⁴b²) = √(a⁴b⁴) = a²b²
Advanced Techniques and Problem Solving
Let's tackle some more complex scenarios that integrate multiple techniques:
Example 11: (√12 + √3) / √3
Here, we need to distribute the denominator:
(√12 / √3) + (√3 / √3) = √4 + 1 = 2 + 1 = 3
Example 12: (√20 - √5) / √5
Similar to the previous example, we distribute the denominator:
(√20 / √5) - (√5 / √5) = √4 - 1 = 2 - 1 = 1
Real-World Applications of Dividing Square Roots
Understanding how to divide square roots isn't just an academic exercise; it has practical applications in various fields, including:
- Physics: Calculations involving distance, velocity, and acceleration often involve square roots.
- Engineering: Structural design and calculations related to forces and stresses frequently utilize square root operations.
- Geometry: Finding lengths of diagonals and other geometric properties often requires dividing square roots.
Conclusion: Mastering Square Root Division
Dividing square roots, while initially appearing complex, becomes manageable with a thorough understanding of the quotient property, simplification techniques, and the process of rationalizing the denominator. By mastering these concepts, you'll equip yourself with a crucial mathematical skill applicable across various disciplines. Remember to practice consistently to solidify your understanding and build confidence in tackling even the most challenging problems. Through consistent practice and application, you can confidently navigate the world of square root division and use this knowledge to solve complex mathematical problems. Remember to always simplify your answers to their most basic form for the most accurate and efficient results.
Latest Posts
Latest Posts
-
Example Of A Line In A Poem
Mar 31, 2025
-
Interval Of Convergence Of A Taylor Series
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How Do You Divide A Square Root . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
