How To Find Moles From Volume
Muz Play
Mar 30, 2025 · 6 min read
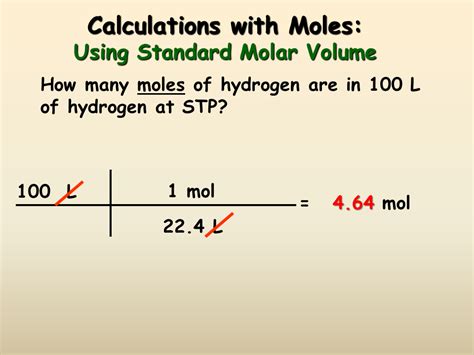
Table of Contents
How to Find Moles from Volume: A Comprehensive Guide
Finding the number of moles from volume requires a deep understanding of chemistry, specifically the relationship between volume, molar mass, and density. This comprehensive guide will walk you through the process, covering various scenarios and offering practical examples. We'll explore different approaches, highlighting the importance of accurate measurements and the application of the ideal gas law in specific situations.
Understanding the Fundamentals: Moles, Volume, and Density
Before diving into the calculations, let's establish a solid foundation on the key concepts:
Moles (mol): A mole is a fundamental unit in chemistry representing a specific number of particles (atoms, molecules, ions, etc.). This number, known as Avogadro's number, is approximately 6.022 x 10<sup>23</sup>. The mole is a crucial concept for relating macroscopic properties (like mass and volume) to microscopic properties (like the number of atoms or molecules).
Volume (L or mL): Volume is the amount of three-dimensional space occupied by a substance. It's usually measured in liters (L) or milliliters (mL) in chemistry. The volume of a substance is directly related to its quantity, especially when dealing with liquids and solids. Gases, however, require a more nuanced approach (explained later).
Density (g/mL or g/L): Density is the mass of a substance per unit volume. It's typically expressed in grams per milliliter (g/mL) or grams per liter (g/L). Density is crucial for converting volume to mass, a necessary step in many mole calculations. The density of a substance is temperature and pressure-dependent, particularly for gases.
Molar Mass (g/mol): Molar mass is the mass of one mole of a substance. It's expressed in grams per mole (g/mol) and is numerically equivalent to the atomic weight (for elements) or the sum of atomic weights (for compounds). This value is readily available from the periodic table or chemical formula.
Calculating Moles from Volume: Different Scenarios
The methods for calculating moles from volume vary depending on whether you're dealing with a solid, liquid, or gas.
1. Solids and Liquids: Using Density and Molar Mass
For solids and liquids, the calculation is straightforward:
Step 1: Determine the mass. Use the density and volume of the substance to calculate its mass using the formula:
Mass (g) = Density (g/mL or g/L) x Volume (mL or L)
Step 2: Calculate the number of moles. Once you have the mass, use the molar mass to calculate the number of moles using the formula:
Moles (mol) = Mass (g) / Molar Mass (g/mol)
Example:
Let's say you have 100 mL of ethanol with a density of 0.789 g/mL. The molar mass of ethanol (C<sub>2</sub>H<sub>5</sub>OH) is approximately 46.07 g/mol.
- Mass: 0.789 g/mL * 100 mL = 78.9 g
- Moles: 78.9 g / 46.07 g/mol ≈ 1.71 mol
Therefore, 100 mL of ethanol contains approximately 1.71 moles.
2. Gases: Using the Ideal Gas Law
Gases are more complex because their volume is highly sensitive to temperature and pressure. The ideal gas law is crucial for calculating moles from the volume of a gas:
PV = nRT
Where:
- P is the pressure (usually in atmospheres, atm)
- V is the volume (usually in liters, L)
- n is the number of moles (mol)
- R is the ideal gas constant (0.0821 L·atm/mol·K)
- T is the temperature (in Kelvin, K)
To find the number of moles (n), rearrange the equation:
n = PV / RT
Example:
Suppose you have a gas sample with a volume of 5.00 L at a pressure of 1.50 atm and a temperature of 298 K.
- Moles: (1.50 atm * 5.00 L) / (0.0821 L·atm/mol·K * 298 K) ≈ 0.306 mol
Therefore, the gas sample contains approximately 0.306 moles.
3. Solutions: Using Molarity
When dealing with solutions (a solute dissolved in a solvent), molarity (M) is a crucial concept. Molarity is defined as the number of moles of solute per liter of solution.
Molarity (M) = Moles (mol) / Volume (L)
To find moles, rearrange the equation:
Moles (mol) = Molarity (M) x Volume (L)
Example:
You have 250 mL of a 0.50 M solution of sodium chloride (NaCl).
- Moles: 0.50 mol/L * 0.250 L = 0.125 mol
Therefore, 250 mL of the 0.50 M NaCl solution contains 0.125 moles of NaCl.
Important Considerations and Potential Pitfalls
-
Units: Maintaining consistent units throughout your calculations is crucial. Convert all measurements to the appropriate units before applying the formulas. Inconsistency in units is a major source of errors.
-
Temperature and Pressure (for gases): The ideal gas law assumes ideal behavior, which is a simplification. Real gases deviate from ideal behavior at high pressures and low temperatures. For accurate results with real gases, you may need to use more complex equations of state.
-
Significant Figures: Pay attention to the significant figures in your measurements and calculations. The final answer should reflect the precision of the input data.
-
Density Variations: The density of a substance can vary with temperature. Ensure you use the appropriate density value for the given temperature.
-
Purity of Substances: The calculations assume the substance is pure. Impurities can affect the mass and therefore the calculated number of moles.
-
Choosing the Right Method: Carefully select the appropriate method based on the state of matter (solid, liquid, or gas) and the available information.
Advanced Applications and Further Exploration
The principles discussed here form the foundation for many more complex chemical calculations. Here are some areas to explore further:
-
Stoichiometry: Once you determine the number of moles, you can use stoichiometry to determine the amounts of reactants and products involved in chemical reactions.
-
Titrations: Titration involves reacting a solution of known concentration with a solution of unknown concentration to determine the unknown concentration, often requiring mole calculations.
-
Gas Laws: Beyond the ideal gas law, exploring real gas laws and their implications will enhance your understanding of gas behavior and calculations.
-
Solution Chemistry: Delving deeper into solution chemistry concepts like molality, mole fraction, and percent composition will improve your ability to handle complex solution calculations.
-
Thermochemistry: Mole calculations are fundamental to thermochemistry, where they are used to relate the heat of reaction to the amounts of substances involved.
Conclusion
Determining the number of moles from volume is a cornerstone of many chemical calculations. Mastering this skill requires a clear understanding of moles, volume, density, molar mass, and the ideal gas law. By carefully considering the specific situation and adhering to the correct procedures, you can accurately determine the number of moles and use this information in various chemical applications. Remember to pay close attention to units, significant figures, and potential deviations from ideal behavior to ensure accurate and reliable results. Continuous practice and a deeper exploration of related chemical concepts will solidify your understanding and ability to solve complex problems.
Latest Posts
Latest Posts
-
Root Mean Square Velocity Of Gas
Apr 01, 2025
-
Where Is The Respiratory Center Located In The Brain
Apr 01, 2025
-
What Is The Difference Between Cellular Respiration And Fermentation
Apr 01, 2025
-
What Are Two Functional Groups Found In Amino Acids
Apr 01, 2025
-
Foundations Of Education 13th Edition Pdf Free Download
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How To Find Moles From Volume . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
