How To Solve Two Step Equations With Division
Muz Play
Apr 06, 2025 · 5 min read
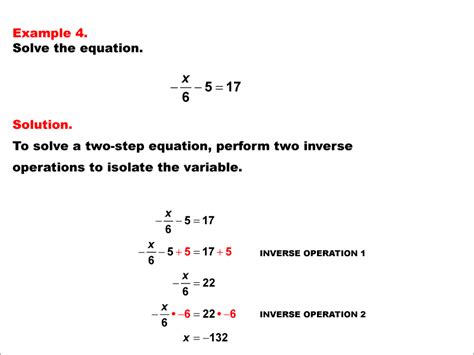
Table of Contents
How to Solve Two-Step Equations with Division: A Comprehensive Guide
Solving equations is a fundamental skill in algebra. Understanding how to manipulate equations to isolate the variable is crucial for success in mathematics and many related fields. This comprehensive guide focuses specifically on solving two-step equations that involve division, providing a step-by-step approach, numerous examples, and tips to improve your problem-solving skills. We'll cover everything from the basic principles to more complex scenarios, ensuring you gain a solid understanding of this essential algebraic concept.
Understanding Two-Step Equations
A two-step equation is an algebraic equation that requires two steps to solve for the unknown variable. These steps typically involve performing inverse operations (opposite operations) to isolate the variable. Equations involving division often require multiplication as the inverse operation. The general form of a two-step equation with division is:
ax / b + c = d
Where:
- a and b are coefficients (numbers multiplying the variable)
- c and d are constants (numbers without variables)
- x is the variable we need to solve for
The Steps to Solving Two-Step Equations with Division
Solving two-step equations with division requires a methodical approach. Here's a step-by-step process:
Step 1: Isolate the Term with the Variable
The first step involves isolating the term containing the variable. This means getting rid of any constants added or subtracted to that term. To do this, perform the inverse operation. If a constant is added, subtract it from both sides of the equation. If a constant is subtracted, add it to both sides.
Step 2: Isolate the Variable
Once the term with the variable is isolated, focus on eliminating any coefficients multiplying or dividing the variable. Since we're dealing with equations involving division, you'll often need to multiply both sides of the equation by the denominator to isolate the variable.
Step 3: Simplify and Check Your Answer
After performing the two steps, simplify the equation to find the value of the variable. Always check your answer by substituting it back into the original equation to ensure it satisfies the equation.
Examples of Solving Two-Step Equations with Division
Let's work through several examples to solidify your understanding.
Example 1: A Basic Equation
Solve for x: (x/3) + 5 = 8
Step 1: Isolate the term with the variable. Subtract 5 from both sides:
(x/3) + 5 - 5 = 8 - 5 x/3 = 3
Step 2: Isolate the variable. Multiply both sides by 3:
3 * (x/3) = 3 * 3 x = 9
Step 3: Check the solution. Substitute x = 9 back into the original equation:
(9/3) + 5 = 8 3 + 5 = 8 8 = 8 (The equation is true, so our solution is correct)
Example 2: Equation with Negative Numbers
Solve for y: (y/(-2)) - 4 = 6
Step 1: Add 4 to both sides:
(y/(-2)) - 4 + 4 = 6 + 4 y/(-2) = 10
Step 2: Multiply both sides by -2:
-2 * (y/(-2)) = 10 * (-2) y = -20
Step 3: Check the solution:
(-20)/(-2) - 4 = 6 10 - 4 = 6 6 = 6 (The solution is correct)
Example 3: Equation with Fractions
Solve for z: (2z/5) + 1/2 = 3/2
Step 1: Subtract 1/2 from both sides:
(2z/5) + 1/2 - 1/2 = 3/2 - 1/2 2z/5 = 1
Step 2: Multiply both sides by 5/2 (the reciprocal of 2/5):
(5/2) * (2z/5) = 1 * (5/2) z = 5/2 or 2.5
Step 3: Check the solution:
(2 * (5/2))/5 + 1/2 = 3/2 1 + 1/2 = 3/2 3/2 = 3/2 (The solution is correct)
Example 4: Equation with a Decimal
Solve for a: (a/2.5) + 3 = 7
Step 1: Subtract 3 from both sides:
(a/2.5) + 3 -3 = 7 - 3 a/2.5 = 4
Step 2: Multiply both sides by 2.5:
2.5 * (a/2.5) = 4 * 2.5 a = 10
Step 3: Check the solution:
(10/2.5) + 3 = 7 4 + 3 = 7 7 = 7 (The solution is correct)
Dealing with More Complex Scenarios
While the examples above illustrate the core process, some equations may present slight variations. Here are a few scenarios to consider:
- Equations with Parentheses: If the equation contains parentheses, simplify the expression within the parentheses first before applying the two-step process.
- Equations with Multiple Variables: If the equation has multiple variables, you might need to rearrange the equation to solve for a specific variable before applying the steps for solving two-step equations with division.
- Equations with Combined Operations: Some equations might involve a combination of addition, subtraction, multiplication, and division. Always follow the order of operations (PEMDAS/BODMAS) to simplify the equation before solving for the variable.
Tips for Success
- Practice Regularly: The key to mastering two-step equations is consistent practice. Work through numerous problems to build your confidence and understanding.
- Show Your Work: Write down each step clearly to avoid errors and to allow you to easily identify where mistakes might have occurred.
- Check Your Answers: Always substitute your solution back into the original equation to verify its accuracy.
- Seek Help When Needed: If you encounter difficulties, don't hesitate to ask for help from a teacher, tutor, or classmate. There are many online resources available as well.
Conclusion
Solving two-step equations with division is a fundamental algebraic skill that builds a strong foundation for more advanced mathematical concepts. By understanding the step-by-step process, practicing regularly, and checking your work, you can master this skill and confidently tackle more complex equations. Remember to break down the problem into manageable steps, and always verify your solution. With dedication and practice, you'll become proficient in solving two-step equations and beyond.
Latest Posts
Latest Posts
-
1 Usos De Ser Y Estar
Apr 09, 2025
-
Sambia Tribe Of Papua New Guinea
Apr 09, 2025
-
What Is The Optimal Ph For Pepsin
Apr 09, 2025
-
Microscopic Anatomy Of Skeletal Muscle Worksheet Answers
Apr 09, 2025
-
Regents Biology Genetics Practice 3 Blood Type Genetics Answer Key
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about How To Solve Two Step Equations With Division . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.