In Dimensional Analysis What Is A Conversion Factor
Muz Play
Mar 26, 2025 · 6 min read
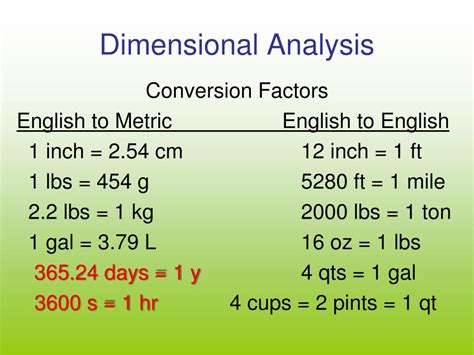
Table of Contents
In Dimensional Analysis: What is a Conversion Factor?
Dimensional analysis is a powerful tool used in various fields, from physics and engineering to chemistry and finance, to ensure the consistency and accuracy of calculations. At its heart lies the concept of the conversion factor, a crucial element that allows us to seamlessly transition between different units of measurement while preserving the underlying physical quantity. This comprehensive guide delves into the intricacies of conversion factors within dimensional analysis, exploring their nature, application, and significance.
Understanding Dimensional Analysis
Before diving into conversion factors, let's establish a firm understanding of dimensional analysis itself. Dimensional analysis is a problem-solving technique that utilizes the dimensions (units) of physical quantities to check the validity of equations, derive relationships between quantities, and convert between units. The fundamental principle is that only quantities with the same dimensions can be added, subtracted, or equated.
This means if you're adding a length to a mass, something is fundamentally wrong with your equation. Dimensional analysis helps identify such errors early on, preventing potentially costly mistakes.
What is a Conversion Factor?
A conversion factor is a ratio that expresses the relationship between two different units that measure the same physical quantity. It's essentially a fraction where the numerator and denominator represent the same quantity but in different units, and their ratio equals 1. Because it equals 1, multiplying a quantity by a conversion factor does not change its value, only its representation.
For example, consider the relationship between meters and centimeters. We know that 1 meter is equal to 100 centimeters. Therefore, we can construct two conversion factors:
- 1 m / 100 cm (This converts from centimeters to meters)
- 100 cm / 1 m (This converts from meters to centimeters)
These conversion factors are crucial because they allow us to change the units of a measurement without altering its actual value.
How to Use Conversion Factors
Using conversion factors is straightforward. The key is to strategically select the factor that cancels out the undesired unit and introduces the desired unit. This involves multiplying the initial quantity by the appropriate conversion factor.
Let's say we want to convert 5 meters to centimeters. We'd use the second conversion factor above:
5 m * (100 cm / 1 m) = 500 cm
Notice how the "m" units cancel out, leaving us with the desired unit, "cm". This process can be extended to involve multiple conversion factors for more complex unit conversions.
Examples of Conversion Factors Across Disciplines
Conversion factors are ubiquitous across various scientific and engineering domains. Here are some examples showcasing their application:
Physics
- Converting speed: Let's say we have a speed of 60 miles per hour and need to convert it to meters per second. We would need several conversion factors involving miles to kilometers, kilometers to meters, and hours to seconds.
- Converting energy: Converting kilowatt-hours (kWh) to Joules requires a conversion factor relating these energy units.
- Converting pressure: Converting pressure from Pascals to atmospheres utilizes a conversion factor based on the defined relationship between these pressure units.
Chemistry
- Converting moles to grams: Using molar mass as a conversion factor allows us to convert between the number of moles of a substance and its mass in grams.
- Converting concentration units: Converting molarity to molality requires a conversion factor that considers the density of the solution.
Engineering
- Converting area: Converting square feet to square meters requires a conversion factor that accounts for the square of the length conversion.
- Converting volume: Converting cubic inches to liters employs a conversion factor based on the cubic relationship between the length units.
Finance
- Converting currencies: Exchange rates serve as conversion factors when converting between different currencies.
- Converting interest rates: Converting annual interest rates to monthly interest rates requires a conversion factor accounting for the time period.
Advanced Applications of Conversion Factors: Compound Unit Conversions
Many real-world scenarios necessitate the conversion of units involving multiple dimensions. For instance, converting from miles per hour to meters per second requires a series of conversion factors:
- Miles to kilometers: 1 mile = 1.609 kilometers
- Kilometers to meters: 1 kilometer = 1000 meters
- Hours to minutes: 1 hour = 60 minutes
- Minutes to seconds: 1 minute = 60 seconds
Let's convert 60 miles per hour to meters per second:
60 mph * (1.609 km/1 mile) * (1000 m/1 km) * (1 hour/60 minutes) * (1 minute/60 seconds) ≈ 26.82 m/s
Notice how each conversion factor is carefully chosen to cancel out the unwanted units, ultimately leaving us with the desired units of meters per second.
Strategies for Choosing the Right Conversion Factors
Selecting the appropriate conversion factors is paramount for accurate conversions. Here's a structured approach:
-
Identify the starting unit and the target unit. Clearly define what unit you're converting from and what unit you're converting to.
-
Find relevant conversion factors. Research or recall the established relationships between the units. Ensure the factors are expressed as ratios (fractions) with the correct units in the numerator and denominator.
-
Strategically arrange the conversion factors. Arrange the conversion factors so that the undesired units cancel out, leaving only the desired units. This often involves a chain of multiplications.
-
Perform the calculation. Once the conversion factors are correctly arranged, perform the mathematical calculation to obtain the converted value.
-
Verify the result. Check the units and the magnitude of the converted value to ensure it's reasonable and consistent with the initial quantity.
Common Pitfalls to Avoid
While conversion factors are simple in principle, some common mistakes can lead to incorrect results:
- Incorrect unit placement: Placing units in the wrong location within the conversion factor leads to incorrect cancellations. Pay close attention to unit placement.
- Inconsistent units: Using conversion factors with inconsistent units will result in errors. Always ensure that units are consistent within a given problem.
- Misinterpreting conversion factor values: Make sure you are using the correct numerical values within the conversion factor.
- Round-off errors: Be mindful of round-off errors during calculations, especially when dealing with multiple conversion factors. Consider carrying more significant figures during intermediate steps to minimize errors.
Conclusion
Conversion factors are the cornerstone of dimensional analysis, providing a systematic and reliable method for unit conversions. By understanding their nature, application, and potential pitfalls, you can confidently utilize them in various contexts to enhance the accuracy and clarity of your calculations. Mastering the art of conversion factors is crucial for anyone involved in scientific, engineering, or financial pursuits where accurate unit conversions are paramount. Through careful planning, diligent execution, and a keen eye for detail, you can effectively harness the power of conversion factors to solve complex unit conversion problems with precision and confidence. Remember, consistent practice and careful attention to detail are key to mastering this essential skill.
Latest Posts
Latest Posts
-
What Is Procedural History In A Case Brief
Mar 29, 2025
-
Is Sweating Negative Or Positive Feedback
Mar 29, 2025
-
Grinstead And Snell Introduction To Probability
Mar 29, 2025
-
What Is A Solution To An Equation
Mar 29, 2025
-
This Is Water By David Foster Wallace Pdf
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about In Dimensional Analysis What Is A Conversion Factor . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
