Newton's Law Of Cooling Differential Equations
Muz Play
Apr 06, 2025 · 6 min read
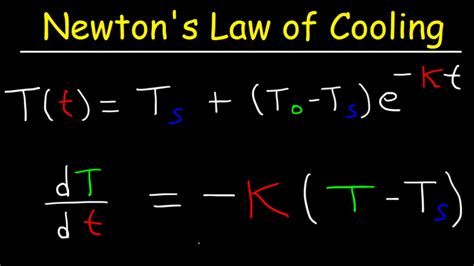
Table of Contents
Newton's Law of Cooling: A Deep Dive into Differential Equations
Newton's Law of Cooling, a cornerstone concept in thermodynamics, elegantly describes how the temperature of an object changes over time as it approaches thermal equilibrium with its surroundings. While seemingly simple, its underlying principles and applications are far-reaching, extending into diverse fields like physics, engineering, and even forensic science. This article will delve into the mathematical formulation of Newton's Law of Cooling using differential equations, exploring its solutions, applications, and limitations.
Understanding the Law
Newton's Law of Cooling states that the rate of heat loss of a body is directly proportional to the difference in temperatures between the body and its surroundings. This seemingly straightforward statement has profound implications when translated into mathematical language. Let's break it down:
-
Rate of heat loss: This refers to how quickly the object's temperature is changing, which is represented by the derivative of temperature with respect to time, dT/dt.
-
Directly proportional: This means that the rate of change is proportional to another quantity. In this case, the proportionality is to the temperature difference.
-
Temperature difference: This is the difference between the object's temperature (T) and the ambient temperature (T<sub>a</sub>), which is the constant temperature of the surroundings.
Mathematical Formulation: The Differential Equation
Translating Newton's Law of Cooling into a differential equation yields:
dT/dt = -k(T - T<sub>a</sub>)
Where:
- dT/dt: Represents the rate of change of temperature with respect to time.
- T: Represents the temperature of the object at time t.
- T<sub>a</sub>: Represents the ambient temperature (constant).
- k: Represents the positive proportionality constant, known as the cooling constant. This constant depends on factors like the object's surface area, material properties, and the heat transfer mechanism (conduction, convection, or radiation). A larger k implies faster cooling. The negative sign indicates that the temperature decreases as time passes.
Solving the Differential Equation
This is a first-order, separable differential equation, meaning we can solve it using techniques from calculus. We separate the variables:
(1/(T - T<sub>a</sub>)) dT = -k dt
Now we integrate both sides:
∫(1/(T - T<sub>a</sub>)) dT = ∫-k dt
This gives us:
ln|T - T<sub>a</sub>| = -kt + C
Where C is the constant of integration. To solve for T, we exponentiate both sides:
|T - T<sub>a</sub>| = e<sup>-kt + C</sup> = e<sup>-kt</sup> * e<sup>C</sup>
Since e<sup>C</sup> is just another constant, let's call it A:
T - T<sub>a</sub> = ±Ae<sup>-kt</sup>
Finally, we can solve for T:
T(t) = T<sub>a</sub> ± Ae<sup>-kt</sup>
The constant A is determined by the initial conditions—the temperature of the object at time t=0. Let's assume that at t=0, the temperature of the object is T<sub>0</sub>. Then:
T<sub>0</sub> = T<sub>a</sub> ± Ae<sup>0</sup> = T<sub>a</sub> ± A
Therefore, A = T<sub>0</sub> - T<sub>a</sub>. Substituting this back into the equation, we get the final solution:
T(t) = T<sub>a</sub> + (T<sub>0</sub> - T<sub>a</sub>)e<sup>-kt</sup>
Analyzing the Solution
This equation provides a complete description of the temperature of the object as a function of time. Let's analyze its key features:
-
As t approaches infinity (t → ∞), e<sup>-kt</sup> approaches 0. This means that the temperature of the object (T(t)) approaches the ambient temperature (T<sub>a</sub>). This is expected, as the object eventually reaches thermal equilibrium with its surroundings.
-
The constant k determines the rate of cooling. A larger k implies faster exponential decay, meaning the object cools down more quickly.
-
The initial temperature difference (T<sub>0</sub> - T<sub>a</sub>) scales the exponential decay. A larger initial temperature difference will result in a larger temperature change over time.
Determining the Cooling Constant (k)
The cooling constant k is crucial for accurate predictions using Newton's Law of Cooling. It can be determined experimentally by measuring the temperature of the object at different times and then fitting the data to the equation T(t) = T<sub>a</sub> + (T<sub>0</sub> - T<sub>a</sub>)e<sup>-kt</sup>. This typically involves using techniques like linear regression after transforming the equation using logarithms. For instance, taking the natural logarithm of both sides:
ln(T(t) - T<sub>a</sub>) = ln(T<sub>0</sub> - T<sub>a</sub>) - kt
Plotting ln(T(t) - T<sub>a</sub>) against t yields a straight line with a slope of -k.
Applications of Newton's Law of Cooling
Newton's Law of Cooling finds applications in diverse fields:
1. Forensic Science: Determining Time of Death
One of the most intriguing applications is in forensic science to estimate the time of death. By measuring the body temperature and knowing the ambient temperature, investigators can use Newton's Law of Cooling to estimate the time elapsed since death. However, this method is subject to several limitations, including the influence of factors such as clothing, body size, and environmental conditions.
2. Engineering: Thermal Design and Control
In engineering, the law is crucial in designing systems involving heat transfer, such as:
-
HVAC systems: Designing efficient heating, ventilation, and air conditioning systems requires understanding how objects cool or heat up in different environments.
-
Electronics cooling: Maintaining optimal operating temperatures for electronic components, especially high-power devices, often relies on heat sinks and cooling systems designed based on principles of heat transfer, including Newton's Law of Cooling.
-
Food processing: Controlling the temperature of food during processing and storage is essential to maintain quality and safety.
3. Meteorology: Studying Atmospheric Processes
Newton's Law of Cooling is applicable in atmospheric sciences to model the rate of cooling of air masses as they rise and expand.
Limitations of Newton's Law of Cooling
While a powerful tool, Newton's Law of Cooling has limitations:
-
Assumption of constant ambient temperature: The law assumes a constant ambient temperature, which may not always hold true in real-world scenarios. Fluctuations in ambient temperature will affect the accuracy of the model.
-
Assumption of uniform temperature: The law assumes that the object's temperature is uniform throughout. This may not be the case for large objects or those with poor thermal conductivity.
-
Heat transfer mechanism: The law assumes a predominantly convective heat transfer mechanism. For objects that lose heat primarily through radiation (e.g., a very hot object), the law may not be entirely accurate. More complex models incorporating radiative heat transfer may be necessary.
Conclusion
Newton's Law of Cooling, despite its relative simplicity, provides a valuable framework for understanding and modeling the cooling (or heating) of objects. Its differential equation formulation allows for quantitative analysis and prediction of temperature changes over time. While it has limitations, its applications across various scientific and engineering disciplines highlight its enduring relevance. Further investigation into more complex heat transfer models can improve the accuracy of predictions, especially in scenarios where the underlying assumptions of Newton's Law are violated. Understanding these limitations and exploring advanced models are crucial for accurate and reliable applications in diverse fields.
Latest Posts
Latest Posts
-
What Is A Transition State Analog
Apr 09, 2025
-
Conservation Of Energy Of A Spring
Apr 09, 2025
-
Convert The Fischer Projection To A Perspective Formula
Apr 09, 2025
-
What Bases Are Found In Rna But Not Dna
Apr 09, 2025
-
Which Term Describes An Enzyme Substrate Reactant Catalyst Product
Apr 09, 2025
Related Post
Thank you for visiting our website which covers about Newton's Law Of Cooling Differential Equations . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.