Polynomial Long Division Worksheet With Answers
Muz Play
Mar 28, 2025 · 4 min read
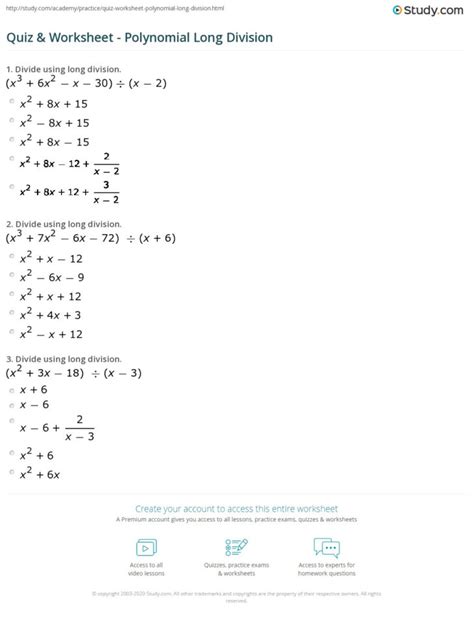
Table of Contents
Polynomial Long Division Worksheet with Answers: A Comprehensive Guide
Polynomial long division might seem daunting at first, but with practice and a structured approach, it becomes manageable. This comprehensive guide provides a detailed explanation of the process, accompanied by a worksheet with solved examples to solidify your understanding. We'll cover the fundamentals, tackle various problem types, and offer strategies for success.
Understanding Polynomial Long Division
Polynomial long division is a method for dividing a polynomial by another polynomial of a lower or equal degree. The process is analogous to long division with numbers, but instead of digits, we work with terms containing variables and exponents. The result is a quotient and a remainder.
Key Components:
- Dividend: The polynomial being divided (the larger one).
- Divisor: The polynomial doing the dividing (the smaller one).
- Quotient: The result of the division.
- Remainder: The amount left over after division.
The general form is:
Dividend = (Divisor)(Quotient) + Remainder
Step-by-Step Guide to Polynomial Long Division
Let's illustrate the process with an example: Divide (3x³ + 5x² – 7x + 2) by (x + 2).
Step 1: Set up the problem.
Write the problem in long division format:
x + 2 | 3x³ + 5x² – 7x + 2
Step 2: Divide the leading terms.
Divide the leading term of the dividend (3x³) by the leading term of the divisor (x): 3x³/x = 3x². This is the first term of your quotient.
3x²
x + 2 | 3x³ + 5x² – 7x + 2
Step 3: Multiply the quotient term by the divisor.
Multiply 3x² by (x + 2): 3x²(x + 2) = 3x³ + 6x².
3x²
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
Step 4: Subtract.
Subtract the result from the dividend: (3x³ + 5x²) – (3x³ + 6x²) = -x². Bring down the next term (-7x).
3x²
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
---------
-x² – 7x
Step 5: Repeat steps 2-4.
Divide the leading term of the new dividend (-x²) by the leading term of the divisor (x): -x²/x = -x. This is the next term of your quotient.
3x² - x
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
---------
-x² – 7x
Multiply -x by (x + 2): -x(x + 2) = -x² - 2x.
3x² - x
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
---------
-x² – 7x
-x² - 2x
Subtract: (-x² – 7x) – (-x² - 2x) = -5x. Bring down the next term (+2).
3x² - x
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
---------
-x² – 7x
-x² - 2x
---------
-5x + 2
Step 6: Repeat steps 2-4 one last time.
Divide -5x by x: -5x/x = -5. This is the final term of your quotient.
3x² - x - 5
x + 2 | 3x³ + 5x² – 7x + 2
3x³ + 6x²
---------
-x² – 7x
-x² - 2x
---------
-5x + 2
-5x -10
Subtract: (-5x + 2) – (-5x - 10) = 12. This is the remainder.
Step 7: Write the final answer.
The quotient is 3x² - x - 5, and the remainder is 12. Therefore, the complete answer is:
3x² - x - 5 + 12/(x + 2)
Polynomial Long Division Worksheet
Now let's put your skills to the test! Here's a worksheet with various polynomial long division problems. Remember to follow the steps outlined above.
Problems:
- Divide (2x³ + 7x² + 5x + 2) by (x + 2)
- Divide (x⁴ – 2x³ + 3x² – 4x + 5) by (x – 1)
- Divide (4x³ – 3x² + 2x – 1) by (2x + 1)
- Divide (6x⁴ + 5x³ – 8x² + 7x – 2) by (3x – 1)
- Divide (x⁵ + 2x⁴ – 3x³ + x² – 2x + 1) by (x² + 1)
Answers:
- 2x² + 3x -1
- x³ - x² + 2x -2 + 3/(x-1)
- 2x² - (5/2)x + (9/4) - (13/4)/(2x+1)
- 2x³ + 7x² - x + 2
- x³ + 2x² -4x + 5 - 6x +6/(x²+1)
Advanced Techniques and Considerations
-
Missing Terms: If the dividend is missing a term (e.g., no x² term), insert a zero as a placeholder to maintain column alignment during subtraction. For example, when dividing (x³ + 2x - 1) by (x - 1), rewrite the dividend as (x³ + 0x² + 2x - 1).
-
Synthetic Division: For divisors of the form (x - c), synthetic division offers a more efficient method. This streamlined approach is particularly useful for higher-degree polynomials. However, long division provides a more visual and conceptually understandable method, especially for beginners.
-
Remainder Theorem: The remainder obtained from polynomial long division is equal to the value of the dividend when the divisor's variable is replaced with the root of the divisor. In our first example, if you substitute x = -2 into 3x³ + 5x² – 7x + 2, you will also get 12, the remainder. This theorem is a powerful tool for verification and problem-solving.
Practicing for Mastery
Consistent practice is key to mastering polynomial long division. Work through numerous problems, starting with simpler ones and gradually increasing complexity. Utilize online resources and textbooks for additional problems and explanations. Don't be afraid to make mistakes – they are valuable learning opportunities! By understanding the underlying principles and practicing diligently, you'll build confidence and proficiency in this essential algebraic technique. Remember to check your work carefully, and consider using different methods like synthetic division (if appropriate) to verify your results. The more you practice, the more intuitive the process will become, paving the way for success in advanced algebraic concepts.
Latest Posts
Latest Posts
-
What Is The Coefficient In A Chemical Equation
Mar 31, 2025
-
The Building Blocks Of Nucleic Acids Are
Mar 31, 2025
-
Why A Cells Size Is Limited
Mar 31, 2025
-
Bones Of The Lower Limb Diagram
Mar 31, 2025
-
Explain How The Skin Helps In Regulating Body Temperature
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Polynomial Long Division Worksheet With Answers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
