Second Moment Of Inertia Parallel Axis Theorem
Muz Play
Apr 01, 2025 · 6 min read
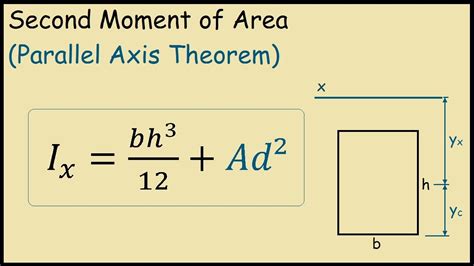
Table of Contents
Second Moment of Area: Parallel Axis Theorem Explained
The second moment of area, also known as the area moment of inertia, is a crucial concept in engineering and physics, particularly in structural analysis and mechanics of materials. It quantifies how the area of a cross-section is distributed relative to a given axis. This property is vital for calculating bending stresses, deflections, and torsional stiffness in beams and other structural elements. Understanding the second moment of area is fundamental to designing safe and efficient structures. This article delves into the intricacies of the second moment of area, focusing particularly on the parallel axis theorem, a powerful tool for simplifying calculations.
What is the Second Moment of Area?
The second moment of area describes the resistance of a cross-sectional area to bending. Imagine bending a beam; a beam with a larger second moment of area will resist bending more effectively than one with a smaller value. It's essentially a measure of a shape's stiffness.
Mathematically, the second moment of area (I) about a given axis is defined as:
I = ∫ r² dA
Where:
- I represents the second moment of area.
- r is the perpendicular distance from an infinitesimal area element (dA) to the axis of rotation.
- dA is an infinitesimal area element.
- ∫ denotes integration over the entire cross-sectional area.
The integration process sums up the contributions of each infinitesimal area element, weighted by the square of its distance from the axis. This weighting emphasizes the importance of areas further from the axis – areas further away contribute more significantly to the overall second moment of area.
Different Axes and Notations
The second moment of area is typically calculated about different axes:
- I<sub>x</sub>: Second moment of area about the x-axis.
- I<sub>y</sub>: Second moment of area about the y-axis.
- I<sub>z</sub>: Second moment of area about the z-axis (often used for three-dimensional analysis).
- I<sub>xy</sub>: Product moment of inertia (measures the distribution of area relative to both x and y axes). This is important for situations involving shear stresses and unsymmetrical bending.
The units of the second moment of area are length to the fourth power (e.g., mm⁴, in⁴).
Calculating the Second Moment of Area: Common Shapes
For simple geometric shapes, standard formulas exist for calculating the second moment of area about their centroidal axes (axes passing through the centroid of the shape). These formulas are derived from the basic integral definition:
- Rectangle: I<sub>x</sub> = (bh³) / 12, where b is the base and h is the height.
- Circle: I<sub>x</sub> = (πd⁴) / 64, where d is the diameter.
- Triangle: I<sub>x</sub> = (bh³) / 36, where b is the base and h is the height (about the base).
- I-Beam: The calculation for an I-beam is more complex, usually involving breaking it down into rectangular components and using the parallel axis theorem (discussed below).
However, for many engineering applications, the axis of rotation is not coincident with the centroidal axis of the shape. This is where the parallel axis theorem becomes incredibly valuable.
The Parallel Axis Theorem: A Powerful Simplification
The parallel axis theorem provides a straightforward method for calculating the second moment of area about any axis parallel to the centroidal axis. It states:
I<sub>axis</sub> = I<sub>c</sub> + Ad²
Where:
- I<sub>axis</sub> is the second moment of area about the parallel axis.
- I<sub>c</sub> is the second moment of area about the centroidal axis (often readily available from standard formulas).
- A is the total area of the cross-section.
- d is the perpendicular distance between the centroidal axis and the parallel axis.
This theorem significantly simplifies calculations because it allows us to use the readily available centroidal second moment of area and then adjust it based on the distance between the centroid and the desired axis. Instead of performing complex integrations for every axis, we can leverage the simpler centroidal calculations.
Applying the Parallel Axis Theorem: A Step-by-Step Example
Let's consider a rectangular cross-section with base 'b' and height 'h'. We want to find the second moment of area (I<sub>x</sub>) about an axis located at a distance 'd' above the centroidal axis.
-
Find the centroidal second moment of area: Using the standard formula for a rectangle, I<sub>c</sub> = (bh³) / 12.
-
Calculate the area: A = bh
-
Determine the distance: The distance 'd' is already defined in the problem statement.
-
Apply the parallel axis theorem: I<sub>x</sub> = I<sub>c</sub> + Ad² = (bh³) / 12 + bh * d²
This calculation is much simpler than directly integrating to find the second moment of area about the new axis. The parallel axis theorem provides a significant shortcut.
Importance of the Parallel Axis Theorem in Structural Engineering
The parallel axis theorem is indispensable in structural engineering for several reasons:
- Simplified Calculations: It drastically simplifies calculations for complex cross-sections. Often, engineers decompose complex shapes into simpler components, calculate the second moment of area for each component using centroidal formulas, and then use the parallel axis theorem to find the total second moment of area about the desired axis.
- Accurate Stress and Deflection Analysis: Accurate calculation of the second moment of area is critical for determining bending stresses and deflections in beams. The parallel axis theorem ensures accurate results, even for asymmetrical loading or complex cross-sections.
- Optimizing Structural Design: Engineers use the parallel axis theorem to explore various cross-sectional shapes and axis locations to optimize the design for maximum stiffness and minimum weight. Understanding how the second moment of area changes with changes in shape and axis location is crucial for this process.
- Composite Sections: It's essential when dealing with composite sections (e.g., a steel beam with a concrete slab on top), allowing for the calculation of the overall second moment of area about a common axis.
Beyond the Basics: Polar Moment of Inertia and Applications
The concept of the second moment of area extends beyond the rectangular and polar coordinate systems. The polar moment of inertia (J) represents the resistance to torsion (twisting). It's defined as the second moment of area about an axis perpendicular to the plane of the cross-section. The parallel axis theorem can also be applied to the polar moment of inertia:
J<sub>axis</sub> = J<sub>c</sub> + Ad²
Where:
- J<sub>axis</sub> is the polar moment of inertia about the parallel axis.
- J<sub>c</sub> is the polar moment of inertia about the centroidal axis.
- A is the area.
- d is the distance between the centroidal axis and the parallel axis.
This has significant implications for designing shafts and other components subjected to torsional loads. Understanding the polar moment of inertia is vital for preventing excessive twisting and ensuring the structural integrity of rotating machinery.
Conclusion: Mastering the Second Moment of Area and Parallel Axis Theorem
The second moment of area and the parallel axis theorem are fundamental tools in engineering analysis. Mastering these concepts is crucial for any engineer working with beams, shafts, or other structural elements. The parallel axis theorem significantly simplifies calculations, enabling accurate and efficient design of structures that can withstand bending and torsional loads. Through a clear understanding of these principles and their practical applications, engineers can ensure the safety and reliability of their designs. Continuous practice and problem-solving will strengthen your grasp of these concepts and their far-reaching implications in various engineering disciplines. By applying the parallel axis theorem correctly, you can accurately predict the behaviour of structures under load, leading to better and more efficient structural designs.
Latest Posts
Latest Posts
-
Pick A Number Between 1 And 51
Apr 02, 2025
-
Do Protists Have Membrane Bound Organelles
Apr 02, 2025
-
Naming Ionic Compounds With Transition Metals
Apr 02, 2025
-
The Subunits Of A Triglyceride Are
Apr 02, 2025
-
Write An Equation Of The Line In Standard Form
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Second Moment Of Inertia Parallel Axis Theorem . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
