Sodium Acetate And Acetic Acid Buffer Equation
Muz Play
Apr 02, 2025 · 6 min read
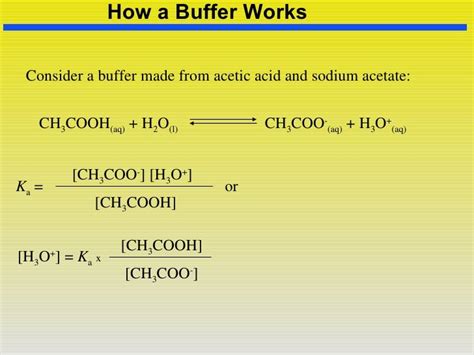
Table of Contents
- Sodium Acetate And Acetic Acid Buffer Equation
- Table of Contents
- Sodium Acetate and Acetic Acid Buffer Equation: A Deep Dive
- Understanding Buffer Solutions
- The Sodium Acetate/Acetic Acid Buffer System
- The Henderson-Hasselbalch Equation
- Calculating the pH of a Sodium Acetate/Acetic Acid Buffer
- Buffer Capacity and its Implications
- Applications of the Sodium Acetate/Acetic Acid Buffer
- Limitations of the Sodium Acetate/Acetic Acid Buffer
- Advanced Considerations: Ionic Strength and Activity Coefficients
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
Sodium Acetate and Acetic Acid Buffer Equation: A Deep Dive
The sodium acetate and acetic acid buffer system is a classic example of a weak acid-conjugate base buffer solution. Understanding its workings, the underlying equilibrium, and the calculations involved is crucial for anyone studying chemistry, biochemistry, or related fields. This comprehensive guide will explore the sodium acetate/acetic acid buffer in detail, explaining its composition, how it works, its applications, and the calculations necessary to determine its pH and buffer capacity.
Understanding Buffer Solutions
Before diving into the specifics of the sodium acetate/acetic acid buffer, let's establish a foundational understanding of buffer solutions in general. A buffer solution resists changes in pH upon the addition of small amounts of acid or base. This resistance to pH change is crucial in many chemical and biological systems where maintaining a stable pH is essential for optimal function. Buffers achieve this stability through the presence of a weak acid and its conjugate base, or a weak base and its conjugate acid.
The effectiveness of a buffer is quantified by its buffer capacity, which represents the amount of acid or base that can be added before a significant change in pH occurs. Buffer capacity is dependent on the concentrations of the weak acid and its conjugate base, as well as the proximity of the solution's pH to the buffer's pKa (the negative logarithm of the acid dissociation constant).
The Sodium Acetate/Acetic Acid Buffer System
This specific buffer utilizes acetic acid (CH₃COOH), a weak acid, and its conjugate base, sodium acetate (CH₃COONa). When dissolved in water, acetic acid partially dissociates according to the following equilibrium:
CH₃COOH(aq) ⇌ CH₃COO⁻(aq) + H⁺(aq)
The addition of sodium acetate introduces a significant concentration of acetate ions (CH₃COO⁻), which are the conjugate base of acetic acid. This increase in the conjugate base concentration shifts the equilibrium to the left, according to Le Chatelier's principle, reducing the concentration of H⁺ ions and thus increasing the pH.
Key characteristics of this buffer:
- Weak acid: Acetic acid is a weak acid, meaning it only partially dissociates in water. This partial dissociation is key to the buffer's ability to resist pH changes.
- Conjugate base: Sodium acetate provides the conjugate base, acetate ions, necessary for the buffer's functionality.
- pH range: This buffer system is most effective within a pH range of approximately 4.74 ± 1, centered around its pKa of 4.74.
The Henderson-Hasselbalch Equation
The pH of a buffer solution can be calculated using the Henderson-Hasselbalch equation:
pH = pKa + log([A⁻]/[HA])
Where:
- pH: The pH of the buffer solution.
- pKa: The negative logarithm of the acid dissociation constant (Ka) of the weak acid (acetic acid in this case). For acetic acid, pKa ≈ 4.74.
- [A⁻]: The concentration of the conjugate base (acetate ions, CH₃COO⁻).
- [HA]: The concentration of the weak acid (acetic acid, CH₃COOH).
This equation is incredibly useful for calculating the pH of a buffer solution given the concentrations of the weak acid and its conjugate base, or for determining the necessary concentrations to achieve a desired pH.
Calculating the pH of a Sodium Acetate/Acetic Acid Buffer
Let's work through an example:
Suppose we have a buffer solution containing 0.1 M acetic acid and 0.2 M sodium acetate. To calculate the pH:
-
Identify the pKa: The pKa of acetic acid is approximately 4.74.
-
Substitute into the Henderson-Hasselbalch equation:
pH = 4.74 + log(0.2 M / 0.1 M)
-
Calculate the pH:
pH = 4.74 + log(2) ≈ 4.74 + 0.30 ≈ 5.04
Therefore, the pH of this buffer solution is approximately 5.04.
Buffer Capacity and its Implications
The buffer capacity refers to the amount of acid or base that can be added to a buffer solution before a significant change in pH occurs. A buffer's capacity is maximized when the concentrations of the weak acid and its conjugate base are equal ([A⁻] = [HA]). In this situation, the pH is equal to the pKa, and the buffer is most resistant to pH changes.
Several factors influence buffer capacity:
- Concentrations of the weak acid and conjugate base: Higher concentrations lead to greater buffer capacity.
- Ratio of weak acid to conjugate base: A ratio closer to 1:1 results in higher buffer capacity.
- The addition of strong acid or strong base: Adding a significant amount of strong acid or base will eventually exceed the buffer's capacity, leading to a sharp change in pH.
Applications of the Sodium Acetate/Acetic Acid Buffer
The sodium acetate/acetic acid buffer finds numerous applications in various fields, including:
-
Biological systems: Maintaining a stable pH in biological experiments and processes is crucial. This buffer is often used in biochemical assays and cell culture to mimic the pH of physiological environments.
-
Food preservation: Acetic acid, the main component of vinegar, is a natural preservative. The buffer system can help control the pH of food products, inhibiting microbial growth and extending shelf life.
-
Chemical analysis: Many chemical reactions require a stable pH for optimal performance. This buffer provides a controlled environment for various analytical techniques.
-
Textile industry: The buffer can help control the pH of dyeing and finishing processes, influencing the quality and color consistency of fabrics.
-
Photography: Some photographic processes require a stable pH environment during development, and this buffer can contribute to the quality and consistency of the results.
Limitations of the Sodium Acetate/Acetic Acid Buffer
While effective within its operational pH range, this buffer has certain limitations:
-
Limited pH range: It is most effective within a relatively narrow pH range around its pKa. Outside this range, its buffering capacity diminishes.
-
Temperature sensitivity: The pKa of acetic acid, and therefore the buffer's pH, is slightly temperature-dependent. Precise pH control may require temperature compensation in sensitive applications.
-
Ionic strength effects: High ionic strength can affect the activity coefficients of the ions, influencing the buffer's effective pH.
Advanced Considerations: Ionic Strength and Activity Coefficients
In more rigorous calculations, particularly at higher concentrations, the activity coefficients of the ions must be considered. The Henderson-Hasselbalch equation, as presented earlier, assumes ideal behavior, where the activity of the ions equals their concentration. However, at higher concentrations, interionic interactions become significant, and the activity coefficients deviate from unity. This requires modifications to the Henderson-Hasselbalch equation to incorporate activity coefficients:
pH = pKa + log(γ_[A⁻][A⁻]/γ_[HA][HA])
Where γ_[A⁻] and γ_[HA] are the activity coefficients of the acetate ion and acetic acid, respectively. Calculating activity coefficients requires considering the ionic strength of the solution and using an appropriate model, such as the Debye-Hückel equation or its extensions.
Conclusion
The sodium acetate/acetic acid buffer system is a simple yet powerful tool for maintaining a stable pH in a wide array of applications. Understanding the underlying equilibrium, the Henderson-Hasselbalch equation, and the factors influencing buffer capacity are crucial for effective use. While the simplified Henderson-Hasselbalch equation provides a good approximation in many cases, more advanced considerations like ionic strength and activity coefficients are necessary for precise pH control in more demanding applications. This detailed explanation provides a solid foundation for anyone working with buffer solutions, emphasizing the importance of understanding both theoretical principles and practical considerations. Further exploration into more complex buffer systems and their applications can build upon this knowledge base.
Latest Posts
Latest Posts
-
Plants Are Photosynthetic Autotrophs What Does This Mean
Apr 07, 2025
-
Equilibrium And Le Chateliers Principle Lab Answer Key
Apr 07, 2025
-
Bile Is Stored And Concentrated In The
Apr 07, 2025
-
Moment Of Inertia Rod About Center
Apr 07, 2025
-
Was Adolf Hitler A Great Leader
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Sodium Acetate And Acetic Acid Buffer Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
