At What Angle Is The Maximum Range In Projectile Motion
Muz Play
Mar 27, 2025 · 5 min read
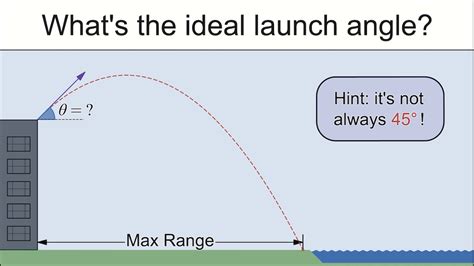
Table of Contents
At What Angle is the Maximum Range in Projectile Motion? A Deep Dive
Projectile motion, the seemingly simple flight of a ball, arrow, or any object launched into the air, reveals a rich tapestry of physics principles. Understanding this motion is crucial in fields ranging from sports science to aerospace engineering. One of the most fundamental questions regarding projectile motion is determining the launch angle that yields the maximum horizontal range – how far the projectile travels horizontally before hitting the ground. This article delves into this question, exploring the physics behind it and discussing factors that influence the optimal launch angle.
The Physics of Projectile Motion
Projectile motion is governed by two independent components: horizontal and vertical motion. Ignoring air resistance (a simplification often used in introductory physics), the horizontal velocity remains constant throughout the flight, while the vertical velocity is affected by gravity. Gravity causes a constant downward acceleration of approximately 9.8 m/s² (on Earth).
Horizontal Motion: Constant Velocity
Since we're neglecting air resistance, there are no horizontal forces acting on the projectile. Newton's first law of motion dictates that an object in motion will stay in motion with the same velocity unless acted upon by a net force. Therefore, the horizontal velocity (Vx) remains constant:
Vx = V₀cosθ
where:
- V₀ is the initial velocity of the projectile.
- θ is the launch angle (measured from the horizontal).
Vertical Motion: Constant Acceleration
The vertical motion is characterized by a constant downward acceleration due to gravity (g). The vertical velocity (Vy) changes over time:
Vy = V₀sinθ - gt
where:
- t is the time elapsed since launch.
The vertical displacement (y) is given by:
y = V₀sinθt - (1/2)gt²
The projectile reaches its maximum height when Vy = 0. This occurs at:
t_max = (V₀sinθ)/g
The maximum height (H) is then:
H = (V₀²sin²θ)/(2g)
Deriving the Range Equation
The total time of flight (T) is twice the time it takes to reach the maximum height:
T = 2(V₀sinθ)/g
The horizontal range (R) is the product of the horizontal velocity and the total time of flight:
R = Vx * T = (V₀cosθ) * (2V₀sinθ)/g = (V₀² * 2sinθcosθ)/g
Using the trigonometric identity 2sinθcosθ = sin(2θ), we get the simplified range equation:
R = (V₀²sin(2θ))/g
Finding the Angle for Maximum Range
From the range equation, it's evident that the range (R) is directly proportional to the square of the initial velocity (V₀²) and a function of the launch angle (θ). To find the angle that maximizes the range, we need to find the maximum value of sin(2θ).
The sine function has a maximum value of 1, which occurs when its argument (2θ) is 90 degrees. Therefore:
2θ = 90°
θ = 45°
Therefore, the maximum range is achieved when the launch angle is 45 degrees.
Factors Affecting the Optimal Launch Angle: Beyond the Ideal
The 45-degree angle for maximum range is a theoretical ideal based on the assumption of no air resistance. In the real world, several factors can influence the optimal launch angle:
Air Resistance
Air resistance is a significant force that opposes the motion of a projectile, especially at higher velocities. Air resistance acts opposite to the direction of motion, reducing both horizontal and vertical velocities. This effect is more pronounced at higher launch angles and velocities. The presence of air resistance generally causes the optimal launch angle to be less than 45 degrees.
Launch Height
If the projectile is launched from a height above the ground, the optimal launch angle changes. A higher launch point allows for a longer time of flight, increasing the range. In these scenarios, the optimal launch angle is generally less than 45 degrees.
Spin and Magnus Effect
Spinning projectiles, like baseballs or golf balls, experience the Magnus effect. This effect causes a force perpendicular to both the direction of motion and the axis of spin. The Magnus effect can significantly influence the trajectory and range, leading to deviations from the ideal 45-degree angle.
Wind
Wind can also affect the trajectory and range of a projectile, causing horizontal and vertical deviations. Headwinds decrease the range while tailwinds increase it. The optimal launch angle needs to be adjusted to compensate for the wind conditions.
Real-world Applications: Where the Theory Meets Practice
The principle of optimizing launch angle for maximum range is applied in various real-world scenarios:
- Sports: In sports like baseball, golf, and long jump, athletes instinctively or through training optimize their launch angle to maximize distance. While the 45-degree angle is a good starting point, they often adjust it based on the specific conditions and factors mentioned above.
- Military Applications: The trajectory and range of projectiles like artillery shells are carefully calculated, considering factors like air resistance, wind, and launch height to ensure accuracy and maximum range.
- Aerospace Engineering: The launch angle of rockets and other aerospace vehicles is critically important for reaching their intended destinations, with factors like atmospheric drag and gravitational pull needing careful consideration.
Conclusion: Beyond the Simple 45 Degrees
While the theoretical optimal launch angle for maximum range in projectile motion is 45 degrees, this is a simplification. In real-world applications, factors such as air resistance, launch height, spin, and wind significantly impact the optimal angle. Understanding these factors is crucial for accurately predicting and controlling the trajectory of projectiles in various fields. By considering these complex interactions, we can move beyond the simplified model and gain a deeper understanding of projectile motion’s intricacies and practical applications. The 45-degree angle serves as a fundamental starting point, a cornerstone of understanding, but the true art lies in adapting this knowledge to the specific complexities of each unique scenario.
Latest Posts
Latest Posts
-
Difference Between The Autonomic And Somatic Nervous System
Mar 30, 2025
-
Examples Of Literary Analysis Thesis Statements
Mar 30, 2025
-
Why Might Two Elements Possess Similar Chemical Properties
Mar 30, 2025
-
Where Does Water Enter The Plant
Mar 30, 2025
-
Cytochrome C Carries How Many Electrons
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about At What Angle Is The Maximum Range In Projectile Motion . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
