Definition For Subtraction Property Of Equality
Muz Play
Mar 30, 2025 · 5 min read
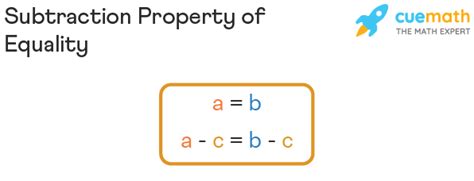
Table of Contents
Understanding the Subtraction Property of Equality: A Comprehensive Guide
The Subtraction Property of Equality is a fundamental concept in algebra, forming the bedrock for solving a wide variety of equations. Mastering this property is crucial for progressing through more advanced mathematical concepts. This comprehensive guide will delve into the definition, applications, and nuances of the Subtraction Property of Equality, providing you with a solid understanding and equipping you with the tools to confidently solve equations.
Defining the Subtraction Property of Equality
The Subtraction Property of Equality states that if you subtract the same number from both sides of an equation, the equation remains balanced, and the solution remains the same. In simpler terms, if you have an equation where both sides are equal, subtracting the same value from both sides will maintain the equality.
Formally, it can be represented as:
If a = b, then a - c = b - c, where 'a', 'b', and 'c' represent numbers or algebraic expressions.
This seemingly simple property is incredibly powerful. It allows us to manipulate equations strategically, isolating the variable and ultimately solving for its value. Let's explore this further.
The Importance of Maintaining Balance
The key concept underlying the Subtraction Property of Equality is the idea of maintaining balance in an equation. Imagine an equation as a perfectly balanced scale. If you add or subtract the same weight from both sides, the scale remains balanced. Similarly, subtracting the same value from both sides of an equation preserves the equality. This principle is fundamental to algebraic manipulation.
Distinguishing from Other Properties
It's important to differentiate the Subtraction Property of Equality from other properties, such as the Addition Property of Equality and the Multiplication Property of Equality. While all three properties contribute to solving equations, they involve different operations:
- Addition Property of Equality: If you add the same number to both sides of an equation, the equation remains true.
- Subtraction Property of Equality: If you subtract the same number from both sides of an equation, the equation remains true.
- Multiplication Property of Equality: If you multiply both sides of an equation by the same non-zero number, the equation remains true.
- Division Property of Equality: If you divide both sides of an equation by the same non-zero number, the equation remains true.
Understanding the distinction between these properties is crucial for selecting the appropriate method for solving a given equation.
Applying the Subtraction Property of Equality: Practical Examples
Let's illustrate the Subtraction Property of Equality with a series of examples, progressing from simple to more complex equations.
Example 1: Simple Linear Equation
Solve for x: x + 5 = 12
Solution:
To isolate 'x', we need to remove the '+5'. According to the Subtraction Property of Equality, we subtract 5 from both sides:
x + 5 - 5 = 12 - 5
This simplifies to:
x = 7
Therefore, the solution to the equation is x = 7.
Example 2: Equation with Variables on Both Sides
Solve for y: 3y + 7 = 2y + 10
Solution:
Here, we have variables on both sides of the equation. Our first step is to simplify by moving the '2y' term to the left side. We achieve this by subtracting 2y from both sides (using the Subtraction Property of Equality):
3y + 7 - 2y = 2y + 10 - 2y
This simplifies to:
y + 7 = 10
Now, we can isolate 'y' by subtracting 7 from both sides:
y + 7 - 7 = 10 - 7
Therefore, y = 3
Example 3: Equation with Fractions
Solve for z: z/2 + 3 = 7
Solution:
First, subtract 3 from both sides:
z/2 + 3 - 3 = 7 - 3
z/2 = 4
Now, to solve for z, we would typically use the Multiplication Property of Equality (multiplying both sides by 2), but the subtraction property was crucial in the first step to isolate the term containing 'z'. This highlights how multiple properties often work together to solve equations.
Therefore, z = 8.
Example 4: Equation with Parentheses
Solve for a: 2(a + 4) - 3 = 11
Solution:
First, simplify the equation by distributing the 2:
2a + 8 - 3 = 11
Combine like terms:
2a + 5 = 11
Subtract 5 from both sides:
2a + 5 - 5 = 11 - 5
2a = 6
Then, use the division property of equality to isolate 'a'. This demonstrates the collaborative nature of algebraic properties.
a = 3
These examples demonstrate the versatility of the Subtraction Property of Equality in solving diverse equations. It's a cornerstone technique for isolating variables and finding solutions.
Advanced Applications and Nuances
The Subtraction Property of Equality extends beyond simple linear equations. Let's explore some more advanced scenarios:
Equations with Inequalities
The Subtraction Property of Equality also applies to inequalities. If you subtract the same number from both sides of an inequality, the inequality remains true (the inequality symbol stays the same).
For example:
If x + 5 > 10, then x + 5 - 5 > 10 - 5, which simplifies to x > 5.
Solving Systems of Equations
While not the primary method, the Subtraction Property of Equality plays a supporting role in solving systems of equations, particularly through the elimination method. By subtracting one equation from another, you can eliminate a variable, enabling you to solve for the remaining variable.
Dealing with Negative Numbers
The Subtraction Property of Equality works equally well with negative numbers. Remember that subtracting a negative number is the same as adding its positive counterpart.
For example:
x - (-3) = 7 is equivalent to x + 3 = 7, leading to x = 4.
Common Mistakes to Avoid
While the Subtraction Property of Equality is relatively straightforward, some common mistakes can hinder your ability to solve equations correctly:
- Subtracting from only one side: Remember, the key is to maintain balance. Always subtract the same value from both sides of the equation.
- Incorrectly combining like terms: Ensure you correctly identify and combine like terms before applying the Subtraction Property of Equality.
- Neglecting to simplify: Simplify both sides of the equation after each step to prevent errors.
Conclusion
The Subtraction Property of Equality is a foundational concept in algebra that enables us to solve a wide range of equations and inequalities. By understanding its definition, applications, and potential pitfalls, you'll develop a stronger foundation in algebra and enhance your ability to tackle increasingly complex mathematical problems. Remember to practice consistently and apply the property diligently to master this essential algebraic skill. The more you practice, the more intuitive and efficient the process will become. Consistent practice with varied examples will solidify your understanding and build your confidence in solving algebraic equations.
Latest Posts
Latest Posts
-
Introduction To Chemical Reactions Answer Key
Apr 01, 2025
-
Is Melting An Ice Cube A Physical Or Chemical Change
Apr 01, 2025
-
Examples Of A Thesis Statement For A Literary Analysis
Apr 01, 2025
-
What Is The Difference Between Self Esteem And Self Efficacy
Apr 01, 2025
-
Classify Each Of The Substances As An Element Or Compound
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Definition For Subtraction Property Of Equality . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
