How Do You Write Complex Numbers In Standard Form
Muz Play
Apr 04, 2025 · 5 min read
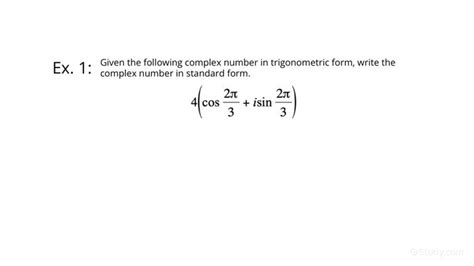
Table of Contents
How Do You Write Complex Numbers in Standard Form? A Comprehensive Guide
Complex numbers, a fascinating extension of the real number system, are essential in various fields like electrical engineering, quantum mechanics, and signal processing. Understanding how to represent these numbers in standard form is crucial for mastering complex number operations. This comprehensive guide will demystify the process, covering everything from the basics to advanced applications. We'll delve into the definition, explore different representations, and tackle numerous examples to solidify your understanding.
Understanding the Building Blocks: Real and Imaginary Parts
Before diving into the standard form, let's review the fundamental components of a complex number:
- Real Part: This is the familiar real number component. Think of it as the "ordinary" number you're used to working with.
- Imaginary Part: This part involves the imaginary unit, denoted by i (or sometimes j in electrical engineering). i is defined as the square root of -1: √(-1) = i. The imaginary part is a real number multiplied by i.
A complex number z is typically expressed as the sum of its real and imaginary parts:
z = a + bi
Where:
- a is the real part (a ∈ ℝ)
- b is the imaginary part (b ∈ ℝ)
- i is the imaginary unit (i² = -1)
This expression, a + bi, is known as the standard form of a complex number.
Visualizing Complex Numbers: The Complex Plane
The complex plane (also called the Argand diagram) provides a powerful visual representation of complex numbers. The horizontal axis represents the real part (a), and the vertical axis represents the imaginary part (b). Each complex number is plotted as a point in this plane, allowing for a geometrical interpretation of complex number operations.
Plotting Complex Numbers on the Argand Diagram:
To plot a complex number a + bi on the Argand diagram:
- Locate the point on the horizontal axis corresponding to the real part (a).
- Locate the point on the vertical axis corresponding to the imaginary part (b).
- The complex number a + bi is represented by the point whose coordinates are (a, b).
This visual representation is extremely helpful when dealing with complex number addition, subtraction, and modulus.
Writing Complex Numbers in Standard Form: Examples and Techniques
Let's explore various scenarios and techniques for expressing complex numbers in standard form:
Example 1: Simple Conversion
Convert 3 + 2i to standard form.
This is already in standard form! The real part is 3, and the imaginary part is 2.
Example 2: Dealing with Negative Imaginary Parts
Convert 5 - 4i to standard form.
This is also in standard form. The real part is 5, and the imaginary part is -4. Note the negative sign is incorporated into the imaginary part.
Example 3: Complex Numbers with Zero Real or Imaginary Parts
- Purely Real Numbers: A real number can be written in standard form as a + 0i. For example, 7 is equivalent to 7 + 0i.
- Purely Imaginary Numbers: A purely imaginary number is expressed as 0 + bi. For example, 6i is equivalent to 0 + 6i.
Example 4: Simplifying Expressions to Standard Form
Write (2 + 3i) + (1 - i) in standard form.
- Combine real parts: 2 + 1 = 3
- Combine imaginary parts: 3i - i = 2i
- Result: 3 + 2i (standard form)
Write (4 + 5i) - (2 + 7i) in standard form.
- Distribute the negative sign: 4 + 5i - 2 - 7i
- Combine real parts: 4 - 2 = 2
- Combine imaginary parts: 5i - 7i = -2i
- Result: 2 - 2i (standard form)
Example 5: Multiplication and Standard Form
Write (3 + 2i)(1 - i) in standard form.
This requires expanding the expression using the distributive property (FOIL):
- Expand: (3)(1) + (3)(-i) + (2i)(1) + (2i)(-i) = 3 - 3i + 2i - 2i²
- Remember i² = -1: 3 - 3i + 2i - 2(-1) = 3 - 3i + 2i + 2
- Combine real and imaginary parts: (3 + 2) + (-3i + 2i) = 5 - i (standard form)
Example 6: Division and Standard Form
Division of complex numbers requires a technique involving the complex conjugate. The complex conjugate of a complex number a + bi is a - bi. Multiplying the numerator and denominator by the conjugate of the denominator will eliminate the imaginary part from the denominator.
Write (2 + 3i) / (1 + i) in standard form.
- Find the conjugate of the denominator: The conjugate of 1 + i is 1 - i.
- Multiply the numerator and denominator by the conjugate: [(2 + 3i)(1 - i)] / [(1 + i)(1 - i)]
- Expand: [(2 - 2i + 3i - 3i²)] / [1 - i²]
- Simplify: [(2 + i + 3)] / [1 + 1] = (5 + i) / 2
- Rewrite in standard form: (5/2) + (1/2)i
Example 7: Dealing with Radicals and Standard Form
Write √(-9) in standard form.
- Rewrite the expression using i: √(-9) = √(9 * -1) = √9 * √(-1) = 3i
- Express in standard form: 0 + 3i
Advanced Applications and Further Exploration
Beyond the basics, complex numbers find applications in:
- Solving polynomial equations: Complex numbers are crucial for finding all roots of polynomial equations, even those with no real solutions.
- Signal processing: Complex numbers are used to represent and manipulate signals in frequency domain analysis.
- Quantum mechanics: Complex numbers are fundamental in describing quantum states and wave functions.
- Electrical engineering: Complex numbers are used extensively in AC circuit analysis.
Conclusion: Mastering Complex Numbers in Standard Form
Writing complex numbers in standard form is a foundational skill in working with these essential mathematical objects. By understanding the real and imaginary parts, utilizing the complex plane for visualization, and practicing the techniques demonstrated in the examples, you'll develop a solid grasp of complex numbers. This mastery will open doors to numerous advanced applications in science, engineering, and mathematics. Remember to always simplify your expressions and ensure the final form is in the a + bi structure to maintain consistency and clarity in your calculations. Through consistent practice and a deeper understanding of the underlying principles, you can confidently navigate the world of complex numbers and their diverse applications.
Latest Posts
Latest Posts
-
Most Of The Oxygen In The Blood Is Transported By
Apr 04, 2025
-
When Does Dna Replication Occur In A Eukaryotic Cell
Apr 04, 2025
-
What Was Shown By Both Redis And Pasteurs Experiments
Apr 04, 2025
-
The Elements In Group 1 Are Called The
Apr 04, 2025
-
Proof Of The Inverse Function Theorem
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about How Do You Write Complex Numbers In Standard Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
