How To Calculate Hydronium Ion Concentration
Muz Play
Apr 03, 2025 · 6 min read
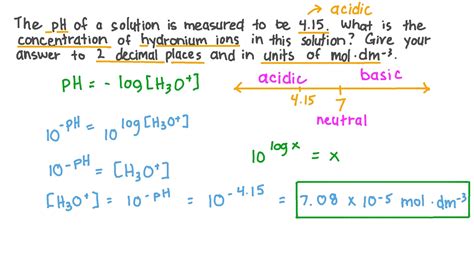
Table of Contents
How to Calculate Hydronium Ion Concentration: A Comprehensive Guide
Determining the hydronium ion concentration ([H₃O⁺]) is fundamental in chemistry, particularly in understanding acid-base reactions and pH calculations. This comprehensive guide will walk you through various methods for calculating [H₃O⁺], covering strong acids, weak acids, strong bases, weak bases, and even more complex scenarios involving polyprotic acids and buffers.
Understanding Hydronium Ions and pH
Before diving into calculations, let's solidify our understanding of hydronium ions and their relationship to pH. Hydronium ions (H₃O⁺) are formed when a proton (H⁺), which is essentially a hydrogen atom that has lost its electron, bonds with a water molecule (H₂O). In aqueous solutions, free protons are exceptionally reactive and quickly associate with water molecules, making H₃O⁺ the predominant form of hydrogen ions.
The pH of a solution is a measure of its acidity or basicity. It's defined as the negative logarithm (base 10) of the hydronium ion concentration:
pH = -log₁₀[H₃O⁺]
Conversely, if you know the pH, you can calculate the hydronium ion concentration:
[H₃O⁺] = 10⁻pH
Calculating [H₃O⁺] for Strong Acids
Strong acids completely dissociate in water, meaning every molecule of the acid donates a proton to a water molecule, forming hydronium ions. The calculation for [H₃O⁺] is straightforward in these cases.
Example: Calculate the hydronium ion concentration of a 0.1 M solution of hydrochloric acid (HCl).
HCl is a strong acid and dissociates completely:
HCl(aq) + H₂O(l) → H₃O⁺(aq) + Cl⁻(aq)
Since the dissociation is complete, the concentration of H₃O⁺ is equal to the initial concentration of HCl:
[H₃O⁺] = 0.1 M
Therefore, a 0.1 M HCl solution has a hydronium ion concentration of 0.1 M.
Calculating [H₃O⁺] for Weak Acids
Weak acids only partially dissociate in water. This means that an equilibrium is established between the undissociated acid and its ions. To calculate [H₃O⁺] for weak acids, we utilize the acid dissociation constant (Ka).
Example: Calculate the hydronium ion concentration of a 0.1 M solution of acetic acid (CH₃COOH), given that its Ka is 1.8 x 10⁻⁵.
The dissociation of acetic acid is represented by:
CH₃COOH(aq) + H₂O(l) ⇌ H₃O⁺(aq) + CH₃COO⁻(aq)
We can use an ICE (Initial, Change, Equilibrium) table to determine the equilibrium concentrations:
| Species | Initial (M) | Change (M) | Equilibrium (M) |
|---|---|---|---|
| CH₃COOH | 0.1 | -x | 0.1 - x |
| H₃O⁺ | 0 | +x | x |
| CH₃COO⁻ | 0 | +x | x |
The Ka expression is:
Ka = [H₃O⁺][CH₃COO⁻] / [CH₃COOH] = 1.8 x 10⁻⁵
Substituting the equilibrium concentrations from the ICE table:
1.8 x 10⁻⁵ = x² / (0.1 - x)
Since Ka is small, we can assume that x is negligible compared to 0.1, simplifying the equation to:
1.8 x 10⁻⁵ ≈ x² / 0.1
Solving for x:
x = √(1.8 x 10⁻⁶) ≈ 1.34 x 10⁻³ M
Therefore, [H₃O⁺] ≈ 1.34 x 10⁻³ M
Calculating [H₃O⁺] for Strong Bases
Strong bases completely dissociate in water, producing hydroxide ions (OH⁻). To find [H₃O⁺], we first need to calculate the pOH and then use the relationship between pH and pOH.
Example: Calculate the hydronium ion concentration of a 0.01 M solution of sodium hydroxide (NaOH).
NaOH is a strong base and dissociates completely:
NaOH(aq) → Na⁺(aq) + OH⁻(aq)
[OH⁻] = 0.01 M
We can calculate the pOH:
pOH = -log₁₀[OH⁻] = -log₁₀(0.01) = 2
Since pH + pOH = 14 at 25°C:
pH = 14 - pOH = 14 - 2 = 12
Now, we can calculate [H₃O⁺]:
[H₃O⁺] = 10⁻pH = 10⁻¹² M
Therefore, the hydronium ion concentration is 10⁻¹² M.
Calculating [H₃O⁺] for Weak Bases
Weak bases only partially dissociate in water. Similar to weak acids, we use the base dissociation constant (Kb) to calculate [OH⁻] first, then determine [H₃O⁺].
Example: Calculate the hydronium ion concentration of a 0.05 M solution of ammonia (NH₃), given that its Kb is 1.8 x 10⁻⁵.
The dissociation of ammonia is:
NH₃(aq) + H₂O(l) ⇌ NH₄⁺(aq) + OH⁻(aq)
Using an ICE table (similar to the weak acid example), we can find [OH⁻]. Once you have [OH⁻], calculate pOH and then pH, finally obtaining [H₃O⁺]. This process is analogous to the weak acid calculation, but using Kb instead of Ka.
Calculating [H₃O⁺] in More Complex Scenarios
Polyprotic Acids
Polyprotic acids can donate more than one proton. Calculations become more complex because you need to consider multiple equilibrium steps. Each dissociation step has its own Ka value. Usually, the first dissociation step is the most significant in determining [H₃O⁺], but in some cases, the second or subsequent steps may contribute significantly, especially in concentrated solutions.
Buffers
Buffer solutions resist changes in pH upon the addition of small amounts of acid or base. The [H₃O⁺] in a buffer solution can be calculated using the Henderson-Hasselbalch equation:
pH = pKa + log₁₀([A⁻]/[HA])
Where [A⁻] is the concentration of the conjugate base and [HA] is the concentration of the weak acid. Once you have the pH, you can calculate [H₃O⁺] as shown previously.
Practical Applications and Considerations
The ability to calculate hydronium ion concentration has significant applications across various scientific and engineering disciplines. Accurate determination of [H₃O⁺] is crucial in:
- Environmental Monitoring: Assessing the acidity of water bodies (lakes, rivers, oceans) is essential for maintaining aquatic life and ecosystem health.
- Chemical Engineering: Many industrial processes are pH-sensitive. Precise control of [H₃O⁺] is critical for optimal reaction yields and product quality.
- Medicine and Biology: Maintaining the proper pH balance in biological systems is crucial for enzyme function and overall health.
- Food Science: The pH of food products influences their texture, flavor, and shelf life.
Remember that the accuracy of [H₃O⁺] calculations depends on the accuracy of the initial data (concentration, Ka, Kb) and the assumptions made during the calculation process. In certain instances, iterative methods or more sophisticated mathematical techniques may be necessary for highly precise results. Furthermore, temperature significantly affects the equilibrium constants (Ka and Kb), so ensuring consistent temperature control is important for accurate measurements.
Conclusion
Calculating hydronium ion concentration is a critical skill in chemistry. This comprehensive guide detailed various methods for strong and weak acids and bases, and introduced more advanced concepts like polyprotic acids and buffers. By mastering these techniques, you'll gain a deeper understanding of acid-base chemistry and its wide-ranging implications across multiple scientific and technological fields. Remember to carefully consider the specific characteristics of the solution and apply the appropriate method for accurate results. Further exploration into more advanced topics like activity coefficients and ionic strength will provide even more refined calculations in complex systems.
Latest Posts
Latest Posts
-
How To Make A Contact Sheet On Bridge
Apr 04, 2025
-
Pretest And Posttest Control Group Design
Apr 04, 2025
-
Max Rate Of Change Directional Derivative
Apr 04, 2025
-
The Energy Of Random Atomic And Molecular Motion Is Called
Apr 04, 2025
-
Is Salt A Compound Or Mixture
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about How To Calculate Hydronium Ion Concentration . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
