How To Choose U And Dv In Integration By Parts
Muz Play
Apr 03, 2025 · 5 min read
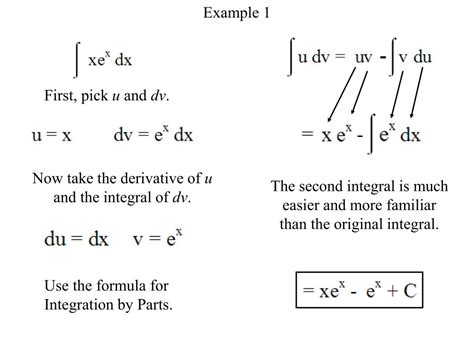
Table of Contents
How to Choose u and dv in Integration by Parts: A Comprehensive Guide
Integration by parts is a powerful technique in calculus that allows us to integrate products of functions. It's essentially the reverse of the product rule for differentiation. However, the effectiveness of this technique hinges heavily on making the right choice for u and dv. This article provides a comprehensive guide on how to strategically select u and dv to simplify the integration process and avoid getting stuck in an endless loop.
Understanding the Integration by Parts Formula
The integration by parts formula is derived from the product rule of differentiation:
d(uv) = u dv + v du
Integrating both sides, we get:
∫d(uv) = ∫u dv + ∫v du
uv = ∫u dv + ∫v du
Rearranging the equation, we obtain the integration by parts formula:
∫u dv = uv - ∫v du
This formula allows us to replace one integral (∫u dv) with another (∫v du), hopefully a simpler one. The key lies in choosing u and dv strategically.
The LIPET Rule: A Helpful Mnemonic
While there's no foolproof method to choose u and dv in every scenario, a helpful mnemonic device is LIPET. This acronym stands for:
- Logarithmic functions
- Inverse trigonometric functions
- Polynomial functions
- Exponential functions
- Trigonometric functions
The order in LIPET suggests a preference for choosing u. Generally, you should choose the function that appears earliest in the LIPET order as your u. The remaining part of the integrand becomes dv.
Let's illustrate this with examples.
Example 1: ∫x e<sup>x</sup> dx
Here, we have a product of a polynomial function (x) and an exponential function (e<sup>x</sup>). According to LIPET, polynomials come before exponentials. Therefore:
- u = x => du = dx
- dv = e<sup>x</sup> dx => v = e<sup>x</sup>
Substituting into the integration by parts formula:
∫x e<sup>x</sup> dx = xe<sup>x</sup> - ∫e<sup>x</sup> dx = xe<sup>x</sup> - e<sup>x</sup> + C
Notice how choosing u as the polynomial simplified the integral significantly.
Example 2: ∫x² cos(x) dx
In this example, we have a polynomial (x²) and a trigonometric function (cos(x)). LIPET suggests choosing the polynomial as u:
- u = x² => du = 2x dx
- dv = cos(x) dx => v = sin(x)
Applying the integration by parts formula:
∫x² cos(x) dx = x² sin(x) - ∫2x sin(x) dx
Observe that we've reduced the power of the polynomial. We'll need to apply integration by parts again to solve ∫2x sin(x) dx. This time:
- u = 2x => du = 2 dx
- dv = sin(x) dx => v = -cos(x)
Therefore:
∫2x sin(x) dx = -2x cos(x) + ∫2 cos(x) dx = -2x cos(x) + 2sin(x) + C
Combining the results:
∫x² cos(x) dx = x² sin(x) + 2x cos(x) - 2sin(x) + C
Example 3: ∫ln(x) dx
This example involves a logarithmic function. Since logarithms appear first in LIPET, we choose it as u:
- u = ln(x) => du = (1/x) dx
- dv = dx => v = x
Applying the integration by parts formula:
∫ln(x) dx = x ln(x) - ∫x(1/x) dx = x ln(x) - ∫1 dx = x ln(x) - x + C
When LIPET Doesn't Provide a Clear Winner
There will be instances where LIPET doesn't provide a clear-cut answer, or applying it leads to a more complex integral. In such cases, consider these additional strategies:
1. Cyclic Integrals:
Some integrals, when using integration by parts repeatedly, lead back to the original integral. These are called cyclic integrals. Let's look at an example:
∫e<sup>x</sup> sin(x) dx
Applying integration by parts twice will lead you back to the original integral. In these cases, you may need to solve the equation for the integral algebraically.
2. Tabular Integration:
For integrals involving polynomials multiplied by easily integrable functions (like exponentials or trigonometric functions), tabular integration offers a streamlined approach. This method avoids repetitive calculations and is particularly useful when dealing with high-degree polynomials.
In this method, you create a table with alternating differentiation and integration columns.
3. Trial and Error:
Sometimes, experimentation is necessary. Try different choices for u and dv and see which approach simplifies the integral. Experience plays a significant role here. The more practice you get, the better you become at intuitively selecting the appropriate u and dv.
Advanced Considerations
Integrating Inverse Trigonometric Functions:
When integrating functions involving inverse trigonometric functions, the choice of u and dv becomes even more crucial. Often, you'll choose the inverse trigonometric function as u, letting the remaining function be dv. Remember that the derivatives of inverse trigonometric functions are algebraic, thereby simplifying the integral ∫v du.
Integrating Products of Functions with Similar Complexity:
When dealing with products of functions of similar complexity (e.g., two trigonometric functions), carefully assess the derivatives and antiderivatives. The choice that results in a simpler integral should be favored, even if it deviates from the strict LIPET order.
The Importance of Practice
Mastering integration by parts requires substantial practice. The more integrals you solve, the better you'll become at recognizing patterns and making the right choices for u and dv. Work through a variety of problems, experimenting with different approaches and learning from your mistakes.
Conclusion
Choosing u and dv strategically is paramount to success with integration by parts. While the LIPET rule provides a valuable guideline, experience, intuition, and a willingness to experiment are equally important. Remember to always check your work by differentiating your final answer to ensure it matches the original integrand. By combining a systematic approach with consistent practice, you'll master this essential calculus technique and unlock the ability to solve a wide range of complex integrals. Don't be afraid to tackle challenging problems; each new challenge helps refine your skill and deepen your understanding of integration by parts.
Latest Posts
Related Post
Thank you for visiting our website which covers about How To Choose U And Dv In Integration By Parts . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
