How To Convert Rectangular To Polar Equations
Muz Play
Mar 24, 2025 · 5 min read
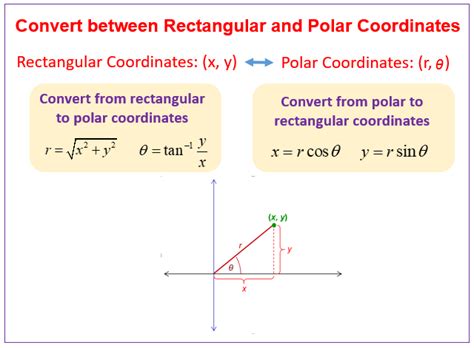
Table of Contents
- How To Convert Rectangular To Polar Equations
- Table of Contents
- How to Convert Rectangular to Polar Equations: A Comprehensive Guide
- Understanding Rectangular and Polar Coordinates
- Rectangular Coordinates (Cartesian Coordinates)
- Polar Coordinates
- The Conversion Formulas: The Bridge Between Two Worlds
- Converting Rectangular Equations to Polar Equations: A Step-by-Step Approach
- Example 1: Converting a Simple Linear Equation
- Example 2: Converting a Circle Equation
- Example 3: Converting a More Complex Equation
- Example 4: Handling Equations Involving both x and y terms
- Advanced Considerations and Challenges
- Practical Applications
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
How to Convert Rectangular to Polar Equations: A Comprehensive Guide
Converting between rectangular (Cartesian) and polar coordinate systems is a fundamental skill in mathematics, particularly useful in calculus, physics, and engineering. This comprehensive guide will walk you through the process of converting rectangular equations to polar equations, explaining the underlying principles and providing numerous examples to solidify your understanding.
Understanding Rectangular and Polar Coordinates
Before diving into the conversion process, let's refresh our understanding of both coordinate systems.
Rectangular Coordinates (Cartesian Coordinates)
Rectangular coordinates represent a point in a two-dimensional plane using its horizontal (x) and vertical (y) distances from the origin (0,0). The origin is the intersection point of the x-axis and the y-axis.
Polar Coordinates
Polar coordinates represent a point using its distance (r) from the origin and the angle (θ) it makes with the positive x-axis. The distance 'r' is always non-negative, and the angle 'θ' is measured in radians or degrees, typically counterclockwise from the positive x-axis.
The Conversion Formulas: The Bridge Between Two Worlds
The key to converting between these systems lies in the following relationships, derived from basic trigonometry:
- x = r cos θ
- y = r sin θ
- r² = x² + y²
- tan θ = y/x (Note: This formula only provides the angle in one quadrant; careful consideration of the signs of x and y is crucial to determine the correct quadrant)
These four equations are your essential tools. Understanding how to apply them effectively is the heart of the conversion process.
Converting Rectangular Equations to Polar Equations: A Step-by-Step Approach
The process typically involves substituting the rectangular coordinates (x and y) with their polar equivalents (r and θ) using the conversion formulas. Let's explore this through several examples, categorized by equation type.
Example 1: Converting a Simple Linear Equation
Let's convert the rectangular equation x = 3 to its polar equivalent.
-
Substitute: We replace 'x' with 'r cos θ':
r cos θ = 3 -
Solve for r (optional): While leaving the equation as
r cos θ = 3is perfectly valid, we can solve for 'r' to get:r = 3 / cos θ = 3 sec θ
Therefore, the polar equivalent of the rectangular equation x = 3 is r cos θ = 3 or r = 3 sec θ. This represents a vertical line.
Example 2: Converting a Circle Equation
Let's convert the rectangular equation x² + y² = 25 (a circle with radius 5 centered at the origin) to polar form.
-
Substitute: We use the relationship
r² = x² + y²:r² = 25 -
Solve for r: Taking the square root of both sides (remembering r is non-negative), we get
r = 5
The polar equation of a circle with radius 5 centered at the origin is simply r = 5. This elegantly illustrates the power of polar coordinates for describing circular shapes.
Example 3: Converting a More Complex Equation
Now let's tackle a more challenging equation: x² - y² = 4.
-
Substitute: Replace 'x' and 'y' with their polar equivalents:
(r cos θ)² - (r sin θ)² = 4 -
Simplify: Expand and simplify the equation:
r²(cos²θ - sin²θ) = 4 -
Use trigonometric identities: Recall the double-angle identity:
cos(2θ) = cos²θ - sin²θ. Substituting this, we get:r² cos(2θ) = 4 -
Solve for r (optional): We can solve for 'r':
r² = 4 / cos(2θ), and thusr = ±2 / √cos(2θ)(Note the ±, arising from the square root).
Therefore, the polar equivalent is r² cos(2θ) = 4 or r = ±2 / √cos(2θ). This equation represents a hyperbola.
Example 4: Handling Equations Involving both x and y terms
Let's convert the equation xy = 4.
-
Substitute: Substitute the polar equivalents:
(r cos θ)(r sin θ) = 4 -
Simplify:
r² cos θ sin θ = 4 -
Use trigonometric identities: Use the double-angle identity:
sin(2θ) = 2 sin θ cos θ. Therefore, the equation becomes:r² (sin(2θ) / 2) = 4 -
Solve for r (optional): Solving for 'r', we get:
r² = 8 / sin(2θ), and thusr = ±√(8 / sin(2θ))
The polar equivalent is r² sin(2θ) = 8 or r = ±√(8/sin(2θ)). This equation represents a rectangular hyperbola.
Advanced Considerations and Challenges
While the fundamental steps outlined above are sufficient for many conversions, certain equations present unique challenges:
-
Dealing with Asymptotes: Some rectangular equations have asymptotes (lines the graph approaches but never touches). Converting these requires careful analysis, and the resulting polar equation might reflect these asymptotes as undefined points or infinite values of 'r'.
-
Equations with Implicit Definitions: If the equation isn't explicitly solved for one variable in terms of the other, the conversion might involve more algebraic manipulation and the use of trigonometric identities to simplify the result.
-
Multiple Solutions: In certain cases, a single rectangular equation can translate into multiple polar equivalents, depending on the way you simplify and solve for 'r' or 'θ'. It's important to be aware of potential multiple solutions.
Practical Applications
The conversion between rectangular and polar coordinates finds numerous applications in various fields:
-
Physics and Engineering: Analyzing projectile motion, calculating forces, and modeling physical phenomena often benefit from using polar coordinates due to the inherent radial symmetry of many systems.
-
Computer Graphics: Polar coordinates are widely used in computer graphics and image processing for creating shapes, defining transformations, and managing circular or radial patterns.
-
Signal Processing: Analyzing signals with rotational symmetry or periodic patterns is often easier using polar coordinates.
Conclusion
Mastering the conversion between rectangular and polar equations is a crucial skill. This guide has provided a step-by-step approach to this conversion, equipped with multiple examples encompassing various equation types, and addressed potential complexities. Remember to practice regularly, experimenting with different equations and building your intuition for the relationships between the two coordinate systems. This understanding will equip you to solve problems effectively and efficiently in numerous mathematical and scientific contexts. By applying the fundamental formulas and understanding trigonometric identities, you'll confidently navigate the world of coordinate transformations.
Latest Posts
Latest Posts
-
Why Is Funding For Schools From Sponsorships A Bad Thing
Mar 29, 2025
-
Mg Oh 2 Is Acid Or Base
Mar 29, 2025
-
What Is The Density Of Maple Syrup
Mar 29, 2025
-
What Is Stronger C C Bond Or C Cl Bond
Mar 29, 2025
-
Where Is The Bacterial Chromosome Located
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about How To Convert Rectangular To Polar Equations . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
