Integration By Parts How To Choose U And Dv
Muz Play
Apr 01, 2025 · 5 min read
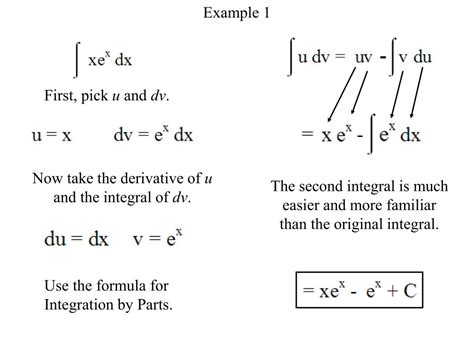
Table of Contents
Integration by Parts: Mastering the Art of Choosing u and dv
Integration by parts is a powerful technique in calculus used to solve integrals that cannot be easily solved using basic integration rules. It's essentially the reverse of the product rule for differentiation. The key to successfully applying integration by parts lies in strategically choosing the u and dv components of the integral. This article will delve deep into the process, providing a comprehensive guide on how to effectively choose u and dv, along with numerous examples to solidify your understanding.
Understanding the Integration by Parts Formula
The integration by parts formula is derived directly from the product rule for differentiation:
d(uv) = u dv + v du
Integrating both sides, we get:
∫d(uv) = ∫u dv + ∫v du
This simplifies to:
uv = ∫u dv + ∫v du
Rearranging the equation, we obtain the integration by parts formula:
∫u dv = uv - ∫v du
This formula allows us to transform a complex integral into a potentially simpler one. The success of this technique heavily depends on the judicious selection of u and dv.
The LIATE Rule: A Helpful Guideline
Choosing u and dv is often the most challenging aspect of integration by parts. While there's no foolproof method, the LIATE rule serves as a valuable heuristic. LIATE stands for:
- Logarithmic functions
- Inverse trigonometric functions
- Algebraic functions (polynomials)
- Trigonometric functions
- Exponential functions
The LIATE rule suggests that you prioritize selecting the function that comes first in the acronym as your u. This is because the u term will be differentiated, and the dv term will be integrated. The goal is to make the integral ∫v du easier to solve than the original integral ∫u dv.
Let's illustrate this with examples.
Examples Applying the LIATE Rule
Example 1: ∫x cos(x) dx
Here, we have an algebraic function (x) and a trigonometric function (cos(x)). Following LIATE, we choose:
u = x(Algebraic)dv = cos(x) dx
Now, we find du and v:
du = dxv = ∫cos(x) dx = sin(x)
Substituting these into the integration by parts formula:
∫x cos(x) dx = x sin(x) - ∫sin(x) dx = x sin(x) + cos(x) + C
Example 2: ∫x² eˣ dx
In this case, we have an algebraic function (x²) and an exponential function (eˣ). LIATE suggests:
u = x²(Algebraic)dv = eˣ dx
Then:
du = 2x dxv = ∫eˣ dx = eˣ
Applying the formula:
∫x² eˣ dx = x²eˣ - ∫2x eˣ dx
Notice that the resulting integral is still not trivial, but it's simpler than the original. We need to apply integration by parts again to solve ∫2x eˣ dx. This process of repeated application is perfectly acceptable. We'll continue this next step:
Let's apply integration by parts again to ∫2x eˣ dx:
u = 2xdv = eˣ dxdu = 2 dxv = eˣ
This gives us:
∫2x eˣ dx = 2x eˣ - ∫2eˣ dx = 2x eˣ - 2eˣ + C
Substituting this back into our original equation:
∫x² eˣ dx = x²eˣ - (2x eˣ - 2eˣ) + C = x²eˣ - 2x eˣ + 2eˣ + C
Example 3: ∫ln(x) dx
This example involves a logarithmic function. Let's choose:
u = ln(x)dv = dx
This leads to:
du = (1/x) dxv = x
Applying the integration by parts formula:
∫ln(x) dx = x ln(x) - ∫x (1/x) dx = x ln(x) - ∫1 dx = x ln(x) - x + C
When LIATE Doesn't Work: Alternative Strategies
While LIATE is a helpful guide, it's not always the definitive answer. Sometimes, you might need to deviate from LIATE or use other strategies. Here are a few scenarios and alternative approaches:
-
Cyclic Integrals: Some integrals, particularly those involving trigonometric functions, may lead to a cycle where repeated application of integration by parts returns you to the original integral. In such cases, you might need to manipulate the equation algebraically to solve for the integral.
-
Tabular Integration: For integrals with polynomial terms multiplied by functions that integrate repeatedly (like exponential or trigonometric functions), tabular integration provides a streamlined approach. It involves creating a table with alternating derivatives of the polynomial and integrals of the other function.
-
Recognizing Patterns: With experience, you'll start to recognize patterns and common integral forms that are amenable to integration by parts. This intuition will help you select
uanddvmore effectively. -
Trial and Error: Sometimes, the only way to find the optimal choice is through trial and error. Don't be afraid to experiment with different combinations of
uanddv.
Advanced Applications and Considerations
-
Definite Integrals: The integration by parts formula works equally well for definite integrals. Simply evaluate the
uvterm at the limits of integration. -
Multiple Applications: As seen in Example 2, it's often necessary to apply integration by parts multiple times to solve an integral completely.
Conclusion: Practice Makes Perfect
Mastering integration by parts requires practice. The more integrals you work through, the better you'll become at choosing u and dv. Start with simple examples, gradually increasing the complexity. Don't hesitate to consult resources like textbooks and online tutorials for additional examples and explanations. Remember, the LIATE rule is a guideline, not a rigid rule. Sometimes, intuition and experimentation are crucial in finding the most effective approach. With consistent practice and a strategic approach to selecting u and dv, you'll confidently tackle even the most challenging integration by parts problems. Remember to always check your work by differentiating the result to ensure it matches the original integrand. Happy integrating!
Latest Posts
Latest Posts
-
What Is The Conflict Of The Play
Apr 02, 2025
-
The Cell Is The Basic Unit Of
Apr 02, 2025
-
Comparison Of Somatic And Autonomic Nervous Systems Concept Map
Apr 02, 2025
-
How To Convert Molecules To Atoms
Apr 02, 2025
-
Ejemplo De Diagrama De Cuerpo Libre
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Integration By Parts How To Choose U And Dv . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
