Ejemplo De Diagrama De Cuerpo Libre
Muz Play
Apr 02, 2025 · 6 min read
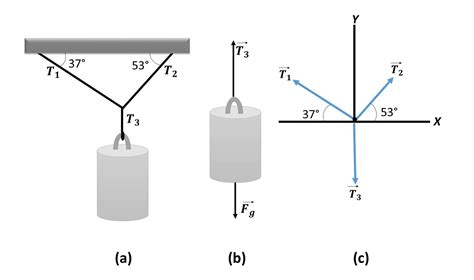
Table of Contents
A Deep Dive into Free Body Diagrams: Examples and Applications
Free body diagrams (FBDs) are fundamental tools in physics and engineering, providing a simplified visual representation of forces acting on a body. Mastering the art of creating accurate and informative FBDs is crucial for solving a wide range of problems, from simple statics to complex dynamics. This comprehensive guide will explore various examples of free body diagrams, detailing their construction and application across diverse scenarios.
What is a Free Body Diagram?
A free body diagram is a simplified sketch that isolates a single body (object, component, or system) from its surroundings. It depicts all the external forces acting on that body, omitting internal forces and complexities. The body is represented by a simple shape (often a box or circle), and each force is represented by an arrow indicating its direction and magnitude. The key is to isolate the body of interest and represent only the external forces influencing its motion or equilibrium.
Why are Free Body Diagrams Important?
FBDs are essential for several reasons:
- Visualization: They offer a clear, visual representation of complex force systems, making it easier to understand the interactions involved.
- Problem Solving: They simplify problem-solving by focusing on the essential forces affecting the body, enabling application of Newton's laws and other principles.
- Communication: They serve as a powerful communication tool, allowing engineers and physicists to clearly convey their analysis and understanding of a system to others.
- Accuracy: A meticulously crafted FBD minimizes errors by systematically accounting for all external forces, leading to more accurate solutions.
Steps to Constructing a Free Body Diagram
Creating a correct FBD involves a systematic approach:
- Identify the Body of Interest: Clearly define the specific object or system you are analyzing. This is the body that will be isolated in the diagram.
- Isolate the Body: Mentally separate the body from its surroundings. Imagine cutting all connections to other bodies.
- Represent the Body: Draw a simplified representation of the body. A simple shape suffices, often a box or a circle.
- Identify External Forces: List all external forces acting on the body. This includes gravitational forces (weight), applied forces (pushes or pulls), normal forces (from surfaces), frictional forces, and tension forces (from ropes or cables).
- Represent Forces as Arrows: Draw each force as an arrow originating from the body’s center of mass (or a point representing the body if the mass distribution is negligible). The arrow’s length should roughly represent the magnitude of the force (although precise scaling isn't strictly necessary), and its direction should accurately reflect the force's direction.
- Label Forces: Clearly label each force with its appropriate symbol (e.g., W for weight, N for normal force, F for applied force, f for friction force, T for tension force) and its magnitude (if known).
- Choose a Coordinate System: Establish a coordinate system (usually x-y or x-y-z) to aid in resolving forces into components.
Examples of Free Body Diagrams
Let's examine various examples to illustrate the construction and application of FBDs:
Example 1: A Block on a Horizontal Surface
Imagine a block resting on a horizontal, frictionless surface. The only external forces acting on the block are its weight (W) acting vertically downwards and the normal force (N) exerted by the surface acting vertically upwards. The FBD would simply show a box representing the block with two vertically aligned arrows: one downwards labeled 'W' and the other upwards labeled 'N'. Since the block is at rest, W = N.
Example 2: A Block on an Inclined Plane
Now, consider a block resting on a frictionless inclined plane. The weight (W) still acts vertically downwards, but it can be resolved into two components: one parallel to the incline (Wsinθ) and one perpendicular to the incline (Wcosθ). The normal force (N) acts perpendicular to the incline, opposing Wcosθ. The FBD would show the block with three arrows: W acting vertically downwards, N acting perpendicular to the incline, and Wsinθ acting parallel to the incline down the slope.
Example 3: A Hanging Mass
A mass hanging from a rope experiences two forces: its weight (W) acting vertically downwards and the tension (T) in the rope acting vertically upwards. The FBD will show a circle or box representing the mass with an arrow downwards labeled 'W' and an arrow upwards labeled 'T'. At equilibrium, W = T.
Example 4: A Pulley System
Consider two masses connected by a rope over a frictionless pulley. Each mass has its own FBD. For mass 1, the forces are its weight (W1) downwards and the tension (T) upwards. For mass 2, the forces are its weight (W2) downwards and the tension (T) upwards (the tension is the same throughout the rope, assuming a massless, frictionless rope).
Example 5: A Block Pulled at an Angle
A block is pulled across a rough horizontal surface by a force F applied at an angle θ above the horizontal. The forces acting on the block are: its weight (W) downwards, the normal force (N) upwards, the applied force F (resolved into horizontal and vertical components Fcosθ and Fsinθ), and the frictional force (f) opposing the motion. The FBD would illustrate all five forces with their respective labels and directions.
Example 6: A Simple Pendulum
A simple pendulum consists of a mass (m) attached to a string of length L. The forces acting on the mass are its weight (W) acting vertically downwards and the tension (T) in the string. The FBD would show the mass with these two forces. The tension force acts along the string towards the pivot point.
Example 7: Two Blocks Connected by a Spring
Two blocks are connected by a spring on a frictionless surface. The forces on Block 1 are its weight (W1), the normal force (N1), and the spring force (Fs) acting towards Block 2. The forces on Block 2 are its weight (W2), the normal force (N2), and the spring force (Fs) acting towards Block 1. The spring force has equal magnitude but opposite direction on each block.
Advanced Applications of Free Body Diagrams
Free body diagrams are not limited to simple scenarios. They are equally crucial in analyzing:
- Structural Analysis: Determining forces in beams, trusses, and other structural elements.
- Fluid Mechanics: Analyzing forces on submerged objects or fluid flow.
- Robotics: Modeling the forces and torques on robotic arms and manipulators.
- Vehicle Dynamics: Analyzing forces on vehicles during acceleration, braking, and turning.
- Aerospace Engineering: Analyzing aerodynamic forces on aircraft and spacecraft.
Tips for Mastering Free Body Diagrams
- Practice regularly: Consistent practice is key to developing proficiency in drawing and interpreting FBDs.
- Start simple, then progress to complex scenarios: Begin with basic examples and gradually increase the complexity.
- Pay attention to detail: Accuracy is crucial in FBDs; ensure correct force directions and labels.
- Use a consistent approach: Follow a systematic approach to ensure you don't miss any forces.
- Check your work: After completing an FBD, review it to verify its accuracy and completeness.
Conclusion
Free body diagrams are invaluable tools for understanding and solving problems in various fields of physics and engineering. By systematically identifying and representing all external forces acting on a body of interest, FBDs simplify complex systems, improve problem-solving efficiency, and enhance communication among professionals. Mastering this fundamental technique is essential for anyone seeking a deeper understanding of mechanics and its applications. Through consistent practice and attention to detail, you can develop the expertise to confidently analyze and solve a wide array of physics and engineering challenges using free body diagrams.
Latest Posts
Latest Posts
-
Light Amplification By The Stimulated Emission Of Radiation
Apr 03, 2025
-
Octet Rule Violation Vs Wrong Electron Total
Apr 03, 2025
-
What Are The Three Points Of Cell Theory
Apr 03, 2025
-
How Many Atoms Are In A Simple Cubic Unit Cell
Apr 03, 2025
-
Boiling Point Is A Chemical Property
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Ejemplo De Diagrama De Cuerpo Libre . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
