Practice Problems For Newton's Second Law Of Motion
Muz Play
Apr 04, 2025 · 7 min read
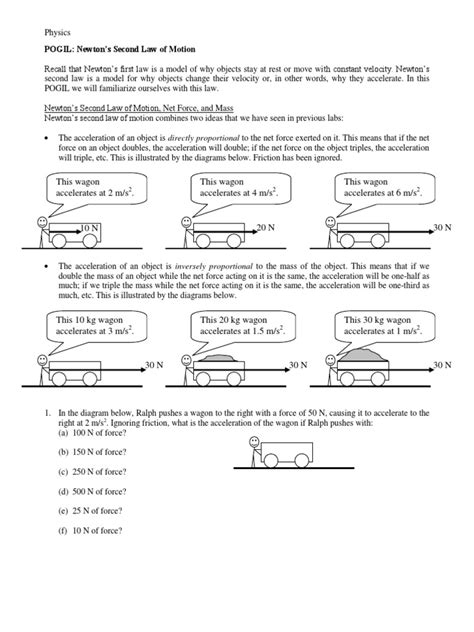
Table of Contents
Practice Problems for Newton's Second Law of Motion
Newton's Second Law of Motion, often summarized as F=ma (Force equals mass times acceleration), is a cornerstone of classical mechanics. Understanding this law is crucial for solving a wide variety of physics problems. This article provides a comprehensive collection of practice problems, ranging from simple to complex, designed to solidify your understanding of Newton's Second Law and its applications. We'll explore various scenarios, including forces acting in one and two dimensions, friction, and systems of multiple objects. Each problem will include a detailed solution, providing step-by-step guidance and explanations. Let's begin!
Understanding Newton's Second Law
Before we dive into the problems, let's reiterate the core concept of Newton's Second Law: The acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. This means:
- Greater force: A larger net force results in a greater acceleration.
- Greater mass: A larger mass results in a smaller acceleration for the same net force.
The equation F=ma encapsulates this relationship, where:
- F represents the net force acting on the object (measured in Newtons). It's crucial to remember that this is the vector sum of all forces acting on the object.
- m represents the mass of the object (measured in kilograms).
- a represents the acceleration of the object (measured in meters per second squared).
Remember, forces are vectors, meaning they have both magnitude and direction. Therefore, you must consider the direction of forces when calculating the net force.
One-Dimensional Motion Problems
These problems involve forces acting along a single line.
Problem 1: Simple Acceleration
A 10 kg object experiences a net force of 25 N. What is its acceleration?
Solution:
We can directly apply the formula F = ma. Solving for 'a', we get:
a = F/m = 25 N / 10 kg = 2.5 m/s²
Therefore, the object accelerates at 2.5 meters per second squared.
Problem 2: Finding the Net Force
A 5 kg object accelerates at 4 m/s². What is the net force acting on it?
Solution:
Using F = ma:
F = 5 kg * 4 m/s² = 20 N
The net force acting on the object is 20 Newtons.
Problem 3: Determining Mass
An object accelerates at 3 m/s² under the influence of a 12 N force. What is its mass?
Solution:
Rearranging the formula to solve for 'm':
m = F/a = 12 N / 3 m/s² = 4 kg
The mass of the object is 4 kilograms.
Problem 4: Force and Friction
A 2 kg block is pushed across a horizontal surface with a force of 15 N. The force of friction opposing the motion is 5 N. What is the acceleration of the block?
Solution:
First, find the net force:
Net Force = Applied Force - Frictional Force = 15 N - 5 N = 10 N
Then, use F = ma to find the acceleration:
a = F/m = 10 N / 2 kg = 5 m/s²
The block accelerates at 5 m/s².
Two-Dimensional Motion Problems
These problems involve forces acting in multiple directions. You'll need to use vector addition to find the net force.
Problem 5: Forces at Right Angles
A 3 kg object is subjected to two forces: a 12 N force acting east and an 8 N force acting north. What is the magnitude and direction of the object's acceleration?
Solution:
- Find the net force: Since the forces are at right angles, we use the Pythagorean theorem to find the magnitude of the net force:
Net Force = √(12² + 8²) = √(144 + 64) = √208 ≈ 14.42 N
- Find the direction of the net force: Use trigonometry to find the angle θ:
θ = tan⁻¹(8/12) ≈ 33.7° north of east
- Find the acceleration:
a = F/m = 14.42 N / 3 kg ≈ 4.81 m/s²
The object accelerates at approximately 4.81 m/s² at an angle of 33.7° north of east.
Problem 6: Forces at an Angle
A 5 kg crate is pulled across a frictionless surface by a rope exerting a force of 20 N at an angle of 30° above the horizontal. What is the horizontal acceleration of the crate?
Solution:
- Resolve the force into components: The horizontal component of the force is:
F_horizontal = 20 N * cos(30°) ≈ 17.32 N
- Find the horizontal acceleration:
a_horizontal = F_horizontal / m = 17.32 N / 5 kg ≈ 3.46 m/s²
The horizontal acceleration of the crate is approximately 3.46 m/s².
Problems Involving Multiple Objects and Systems
These problems require considering the interactions between multiple objects.
Problem 7: Connected Objects
Two blocks, one with a mass of 2 kg and the other with a mass of 3 kg, are connected by a massless string passing over a frictionless pulley. What is the acceleration of the system? (Assume g = 9.8 m/s²)
Solution:
-
Identify forces: The 3 kg block experiences a downward force of gravity (3kg * 9.8 m/s²) and an upward tension force (T). The 2 kg block experiences an upward force of gravity (2kg * 9.8 m/s²) and a downward tension force (T).
-
Apply Newton's Second Law to each block:
- 3 kg block: 3g - T = 3a
- 2 kg block: T - 2g = 2a
-
Solve the system of equations: Add the two equations to eliminate T:
g = 5a
a = g/5 = 9.8 m/s²/5 ≈ 1.96 m/s²
The acceleration of the system is approximately 1.96 m/s².
Problem 8: Atwood Machine
An Atwood machine consists of two masses, m1 = 4 kg and m2 = 6 kg, connected by a massless string over a frictionless pulley. Find the acceleration of the masses and the tension in the string.
Solution:
This problem is similar to Problem 7, requiring the creation and solution of simultaneous equations based on Newton's second law for each mass. The acceleration will be:
a = (m2 - m1)g / (m1 + m2) = (6kg - 4kg) * 9.8 m/s² / (6kg + 4kg) ≈ 1.96 m/s²
The tension can then be found by substituting 'a' back into either of the original equations.
Advanced Problems: Incorporating More Complex Concepts
These problems introduce additional concepts that build upon the fundamental principles of Newton's Second Law.
Problem 9: Inclined Plane
A 5 kg block slides down a frictionless inclined plane with an angle of 30° to the horizontal. What is its acceleration?
Solution:
- Resolve gravity: The component of gravity acting parallel to the incline is:
F_parallel = mg * sin(30°) = 5 kg * 9.8 m/s² * sin(30°) ≈ 24.5 N
- Find the acceleration:
a = F_parallel / m = 24.5 N / 5 kg ≈ 4.9 m/s²
The acceleration of the block down the incline is approximately 4.9 m/s².
Problem 10: Block and Pulley System with Friction
A 4 kg block rests on a rough horizontal surface (μk = 0.2). It's connected by a massless string over a frictionless pulley to a hanging 2 kg block. Find the acceleration of the system.
Solution:
This problem combines the concepts of Newton's Second Law, friction, and connected objects. It requires careful consideration of the forces acting on each block, including the frictional force opposing the motion of the 4 kg block. The solution involves setting up and solving simultaneous equations, accounting for the frictional force (F_friction = μk * m * g). The resulting acceleration will be lower than in a frictionless system.
These problems provide a solid foundation for understanding and applying Newton's Second Law. Remember to always:
- Draw a free-body diagram: This helps visualize all forces acting on each object.
- Resolve forces into components: Break down forces into their x and y components when necessary.
- Apply Newton's Second Law to each object: Write separate equations for each object in the system.
- Solve the resulting equations: Use algebra to solve for the unknowns (acceleration, tension, etc.).
By practicing these problems, you'll significantly improve your understanding of Newtonian mechanics and your ability to solve more complex physics problems. Remember to practice regularly and to seek clarification whenever needed. Good luck!
Latest Posts
Latest Posts
-
What Is The End Product Of Citric Acid Cycle
Apr 04, 2025
-
How To Find The Zeros Of A Function Algebraically
Apr 04, 2025
-
Which Is A Postulate Of The Kinetic Molecular Theory
Apr 04, 2025
-
What Are The Units For A Spring Constant
Apr 04, 2025
-
How To Make A Contact Sheet On Bridge
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Practice Problems For Newton's Second Law Of Motion . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
