Skewed Left Stem And Leaf Plot
Muz Play
Apr 02, 2025 · 6 min read
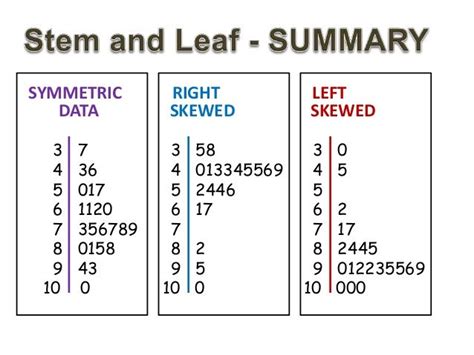
Table of Contents
Skewed Left Stem and Leaf Plots: Understanding and Interpreting Data Distribution
A stem and leaf plot is a valuable tool in descriptive statistics, providing a simple yet effective way to visualize the distribution of a dataset. Understanding how data is distributed is crucial for making informed decisions, identifying trends, and drawing meaningful conclusions. While symmetric distributions are ideal, real-world data often exhibits skewness, leaning either to the left (negatively skewed) or to the right (positively skewed). This article delves deep into the intricacies of skewed left stem and leaf plots, explaining what they represent, how to interpret them, and their significance in data analysis.
What is a Stem and Leaf Plot?
Before we delve into skewed left distributions, let's briefly review the basics of stem and leaf plots. A stem and leaf plot is a visual representation of data that organizes data points into "stems" and "leaves." The stem represents the leading digit(s) of a data point, while the leaf represents the trailing digit(s). This allows for a quick overview of the data's distribution, including the range, central tendency, and presence of outliers.
For example, consider the dataset: 12, 15, 18, 21, 23, 25, 28, 30, 32.
A stem and leaf plot for this data would look like this:
Stem | Leaf
-------
1 | 2 5 8
2 | 1 3 5 8
3 | 0 2
Here, the "stems" represent the tens digits (1, 2, 3), and the "leaves" represent the units digits.
Understanding Skewness in Data Distribution
Skewness refers to the asymmetry of a data distribution. A symmetric distribution is balanced, with data points evenly distributed around the mean. However, many real-world datasets are skewed, meaning the data is concentrated more on one side of the mean than the other.
-
Positively Skewed (Right Skewed): The tail of the distribution extends to the right, meaning there are more data points clustered at the lower end, with fewer higher values. Think of income distribution – most people earn lower incomes, with fewer earning extremely high incomes.
-
Negatively Skewed (Left Skewed): The tail of the distribution extends to the left, indicating a concentration of data points at the higher end, with fewer lower values. An example might be test scores where most students score high, with a few scoring significantly lower.
We are focusing on negatively skewed (left-skewed) distributions in this article.
Identifying a Skewed Left Stem and Leaf Plot
A negatively skewed stem and leaf plot exhibits several key characteristics:
-
Long Left Tail: The most prominent feature is a long tail extending towards the lower values (left side). This tail represents a smaller number of data points significantly lower than the majority.
-
Clustered Higher Values: The majority of the data points are clustered towards the higher end of the range. The leaves on the higher stem values will be more numerous and densely packed.
-
Mean Less Than Median: In a negatively skewed distribution, the mean (average) is typically less than the median (middle value). This is because the few low values pull the mean downwards.
-
Visual Representation: The plot itself visually shows the asymmetry. The data points are concentrated on the right-hand side, with a few outliers stretching out to the left.
Example of a Skewed Left Stem and Leaf Plot
Let's consider a dataset representing the ages of participants in a senior citizens' chess tournament:
72, 75, 78, 79, 80, 81, 82, 83, 84, 85, 86, 87, 88, 89, 90, 65, 68
The stem and leaf plot would look like this:
Stem | Leaf
-------
6 | 5 8
7 | 2 5 8 9
8 | 0 1 2 3 4 5 6 7 8 9
9 | 0
This plot displays a clear left skewness. Notice the long tail extending to the left (lower ages) with only two data points (65 and 68), while the majority of participants are clustered in the 70s, 80s, and 90s. The presence of these lower values significantly impacts the mean, pulling it lower than the median.
Interpreting a Skewed Left Stem and Leaf Plot
Interpreting a skewed left stem and leaf plot involves identifying key features and understanding their implications:
-
Identify the range: Determine the minimum and maximum values to understand the overall spread of the data.
-
Observe the clustering: Notice where the majority of the data points are concentrated. This area indicates the typical or modal value.
-
Examine the tail: The length and values in the left tail reveal the presence and magnitude of outliers or unusual low values. These outliers can significantly influence the mean, pulling it downwards.
-
Calculate the median and mean: Comparing the median and mean provides further insight into the skewness. In a left-skewed distribution, the median will be higher than the mean.
-
Consider the context: The interpretation of the skewness must always be considered within the context of the data. What do these values represent? What are the implications of this distribution?
Real-World Applications of Skewed Left Data
Understanding and interpreting left-skewed distributions is important across many fields:
-
Healthcare: Analyzing patient recovery times, where most patients recover quickly, with a few experiencing longer recovery periods.
-
Education: Examining test scores, where the majority of students perform well, but a few struggle significantly.
-
Finance: Assessing investment returns, where most investments show positive returns, but a few experience significant losses.
-
Manufacturing: Analyzing product lifespan, where most products last a long time, but a few malfunction early.
Advantages of Using Stem and Leaf Plots
Stem and leaf plots offer several advantages over other visualization methods, particularly when dealing with skewed data:
-
Simplicity: They are easy to understand and create, making them suitable for educational purposes and quick data exploration.
-
Data Retention: Unlike histograms, stem and leaf plots retain the original data values, providing a more detailed view.
-
Visual Clarity: They clearly show the distribution, including the spread, central tendency, and presence of outliers, making it easier to identify skewness.
-
Efficiency: They are a compact and efficient way to represent data, especially for moderately sized datasets.
Limitations of Stem and Leaf Plots
While stem and leaf plots are useful, they do have limitations:
-
Large Datasets: They are not suitable for extremely large datasets, as they can become cumbersome and difficult to interpret.
-
Decimal Data: Handling decimal data requires adjustments and can complicate the plot's readability.
-
Categorical Data: They are not suitable for categorical data; other visualization methods are better suited for such data.
Conclusion
Skewed left stem and leaf plots are a powerful tool for visualizing and understanding data distributions exhibiting negative skewness. By carefully examining the plot's features – the long left tail, clustered higher values, and the relationship between the mean and median – we can gain valuable insights into the underlying data. Recognizing the characteristics of a left-skewed distribution is critical for making informed decisions in various fields, from healthcare to finance. While stem and leaf plots have limitations, their simplicity, data retention, and visual clarity make them an invaluable tool in descriptive statistics, particularly for initial exploration and understanding of skewed data. Mastering the interpretation of these plots empowers data analysts to derive meaningful conclusions from their data, leading to better decision-making.
Latest Posts
Latest Posts
-
Does Electron Withdrawing Groups Increase Acidity
Apr 03, 2025
-
Is The Freezing Of Water A Chemical Change
Apr 03, 2025
-
What Are The Functions Of Stems
Apr 03, 2025
-
Heat Of Vaporization Of Water J Kg
Apr 03, 2025
-
What Happens To Plant Cells In A Hypertonic Solution
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Skewed Left Stem And Leaf Plot . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
