What Is A Net Change In Math
Muz Play
Apr 05, 2025 · 6 min read
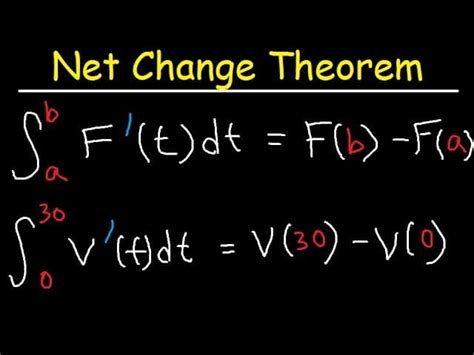
Table of Contents
What is Net Change in Math? A Comprehensive Guide
Net change, a fundamental concept in mathematics, represents the overall difference between a starting value and an ending value. It's a simple yet powerful tool used across various mathematical fields, from basic arithmetic to advanced calculus. Understanding net change is crucial for analyzing trends, comparing values, and solving problems in numerous real-world applications. This comprehensive guide will explore the concept of net change in detail, covering its definition, calculation methods, applications, and practical examples.
Understanding the Core Concept: Net Change
The core idea behind net change is to determine the final result after considering all increases and decreases. It's essentially the difference between the final state and the initial state. Imagine tracking your bank account balance throughout the day. You might have multiple deposits (increases) and withdrawals (decreases). The net change would be the overall difference in your balance from the beginning of the day to the end, regardless of the individual transactions.
Mathematically, net change is calculated by subtracting the initial value from the final value. This can be represented by the following formula:
Net Change = Final Value - Initial Value
This simple formula is applicable across various contexts, making it a versatile tool in mathematical analysis.
Calculating Net Change: Step-by-Step Examples
Let's illustrate the calculation of net change with several examples:
Example 1: Simple Arithmetic
Suppose you start with $100 in your savings account. You deposit $50 and then withdraw $20. What is the net change in your account balance?
- Initial Value: $100
- Final Value: $100 + $50 - $20 = $130
- Net Change: $130 - $100 = $30
The net change in your account balance is $30.
Example 2: Temperature Fluctuation
Imagine the temperature outside starts at 60°F. It increases by 15°F and then decreases by 8°F. What's the net change in temperature?
- Initial Value: 60°F
- Final Value: 60°F + 15°F - 8°F = 67°F
- Net Change: 67°F - 60°F = 7°F
The net change in temperature is 7°F.
Example 3: Stock Market Analysis
A company's stock price starts at $25 per share. It increases to $32 and then drops to $28. What is the net change in the stock price?
- Initial Value: $25
- Final Value: $28
- Net Change: $28 - $25 = $3
The net change in the stock price is $3. Note that we only consider the final value compared to the initial value, ignoring the intermediate fluctuations.
Net Change in More Complex Scenarios
While the basic formula remains consistent, calculating net change can become more complex when dealing with:
1. Multiple Variables:
When multiple factors contribute to the change, you need to account for each factor's impact. For example, calculating the net change in a company's profit might involve considering changes in revenue, cost of goods sold, and operating expenses. You would sum the individual impacts to find the overall net change in profit.
2. Functions and Graphs:
In calculus, net change is often represented graphically. The net change between two points on a function's graph is the difference in the y-values (function values) corresponding to the two x-values (input values). This can be visualized as the vertical distance between the two points. The concept extends to integral calculus where the definite integral represents the net change of a function over an interval.
3. Discrete vs. Continuous Change:
Net change can apply to both discrete and continuous data. Discrete data involves distinct, separate values (like the number of cars sold), while continuous data involves a continuous range of values (like temperature or time). The calculation of net change remains the same, but the interpretation might differ.
4. Negative Net Change:
A negative net change simply indicates a decrease in value from the initial state to the final state. This is perfectly valid and a common occurrence in various applications. For instance, a negative net change in a company's inventory signifies a reduction in stock levels.
Applications of Net Change in Real Life
Net change is a broadly applicable concept with uses across numerous fields:
1. Finance:
- Tracking account balances: As illustrated earlier, net change is essential for monitoring financial accounts.
- Investment analysis: Determining the net gain or loss on investments.
- Budgeting: Calculating the difference between planned spending and actual spending.
2. Science:
- Tracking population changes: Determining the net increase or decrease in a population over a period.
- Measuring physical quantities: Calculating net changes in temperature, pressure, or velocity.
- Analyzing chemical reactions: Monitoring net changes in reactant and product concentrations.
3. Business:
- Profit and loss analysis: Determining net profit or loss over a given period.
- Inventory management: Tracking the net change in inventory levels.
- Sales analysis: Calculating the net increase or decrease in sales revenue.
4. Engineering:
- Monitoring system performance: Tracking net changes in system parameters like voltage or current.
- Structural analysis: Evaluating the net change in stress or strain on a structure.
5. Everyday Life:
- Weight management: Tracking net weight gain or loss.
- Fuel efficiency: Calculating the net change in fuel level in a vehicle.
- Distance traveled: Determining the net displacement from a starting point to an ending point.
Beyond Basic Calculation: Analyzing Trends and Patterns
While calculating the net change provides a concise summary of overall difference, analyzing the individual changes that contribute to this net change can provide valuable insights. Consider the following scenarios:
- Consistent Growth: A consistently positive net change indicates a consistent upward trend.
- Consistent Decline: A consistently negative net change suggests a consistent downward trend.
- Fluctuations: A series of positive and negative changes could reveal cycles or seasonality.
- Accelerated Change: The magnitude of changes could indicate increasing or decreasing rates of growth or decline.
By analyzing the individual components and patterns within the changes, we gain a more profound understanding of the underlying dynamics driving the net change. This type of analysis is crucial for decision-making in various fields, allowing for more effective forecasting and planning.
Net Change in Advanced Mathematics: Integral Calculus
In integral calculus, the concept of net change takes on a more sophisticated form. The definite integral of a function over a given interval represents the net change of that function over that interval. This means the integral calculates the accumulated effect of the function's values across the entire interval, taking into account both positive and negative contributions. This is a powerful tool for calculating areas under curves and finding the total change in quantities that vary continuously over time or space.
Conclusion: The Power and Versatility of Net Change
Net change, though a seemingly simple mathematical concept, holds immense power and versatility. Its ability to quantify the overall difference between initial and final values makes it an indispensable tool across various disciplines. Understanding how to calculate and interpret net change, coupled with analyzing the underlying trends and patterns within the changes themselves, empowers individuals and organizations to make informed decisions and effectively navigate the complexities of the real world. From basic arithmetic to advanced calculus, the concept of net change remains a cornerstone of mathematical analysis and practical application.
Latest Posts
Latest Posts
-
How To Prove A Transformation Is Linear
Apr 06, 2025
-
Is The Mutant Allele Dominant Or Recessive
Apr 06, 2025
-
Why Do Covalent Compounds Have Low Melting Points
Apr 06, 2025
-
Which Of The Following Determines The Length Of The Base
Apr 06, 2025
-
Carboxylic Acids Can Be Made By The Hydrolysis Of Nitriles
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is A Net Change In Math . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
