What Is A Quarter In Percentage
Muz Play
Mar 28, 2025 · 5 min read
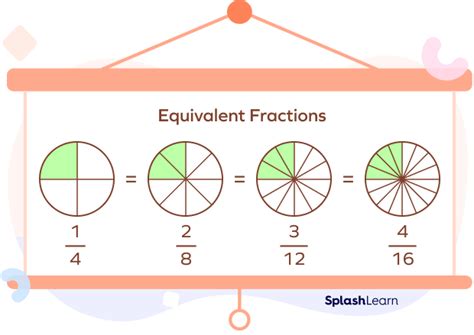
Table of Contents
What is a Quarter in Percentage? A Comprehensive Guide
Understanding fractions and their percentage equivalents is fundamental to various aspects of life, from calculating discounts and taxes to comprehending statistical data and financial reports. This comprehensive guide will delve deep into the concept of "a quarter" and its representation as a percentage, clarifying any confusion and providing practical applications. We'll explore the relationship between fractions, decimals, and percentages, equipping you with the knowledge to confidently handle these conversions in diverse situations.
Understanding Fractions: The Building Blocks
Before diving into percentages, let's solidify our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The denominator indicates the total number of equal parts the whole is divided into, while the numerator specifies how many of those parts are being considered.
For example, the fraction 1/4 (one-quarter) signifies that a whole has been divided into four equal parts, and we are focusing on one of those parts. Other common fractions include 1/2 (one-half), 1/3 (one-third), and 2/3 (two-thirds). Understanding these basic fractions is crucial for grasping the concept of a quarter in percentage terms.
Decimals: The Bridge Between Fractions and Percentages
Decimals provide a bridge between fractions and percentages. They are a way of representing fractions using base-10 notation. To convert a fraction to a decimal, you simply divide the numerator by the denominator.
For example, to convert the fraction 1/4 to a decimal, we perform the division: 1 ÷ 4 = 0.25. This means that one-quarter is equal to 0.25 in decimal form. Similarly, 1/2 = 0.5, 1/3 ≈ 0.333 (recurring decimal), and 2/3 ≈ 0.667 (approximate). The decimal representation makes it easier to visualize the magnitude of the fraction and facilitates conversion to percentages.
Percentages: Expressing Parts as a Hundredth
Percentages are a special type of fraction where the denominator is always 100. They represent a portion of a whole as parts per hundred. The percentage symbol (%) indicates that a number is expressed as a percentage.
To convert a decimal to a percentage, we multiply the decimal by 100 and add the percentage symbol. Conversely, to convert a percentage to a decimal, we divide the percentage by 100.
Let's apply this to our example of one-quarter (1/4):
- Fraction: 1/4
- Decimal: 0.25
- Percentage: 0.25 x 100% = 25%
Therefore, one-quarter is equal to 25%. This is a crucial equivalence to remember, as it's frequently encountered in various contexts.
Practical Applications of Understanding "A Quarter" as 25%
The understanding that a quarter equates to 25% has far-reaching practical implications across diverse fields. Let's explore some key applications:
1. Financial Calculations:
- Discounts: A 25% discount on an item means you pay only 75% (100% - 25%) of the original price. For instance, a $100 item with a 25% discount will cost $75.
- Interest Rates: Interest rates on loans or savings accounts are often expressed as percentages. A quarter of a percentage point (0.25%) might seem small, but it can significantly affect the total interest paid or earned over time.
- Tax Calculations: Sales tax or other taxes are frequently calculated as a percentage of the total cost. If the tax rate is 25%, you would pay an additional 25% on the price of the goods or services.
- Profit Margins: Businesses use percentages to determine profit margins. A 25% profit margin means that 25% of the revenue is profit.
2. Data Analysis and Statistics:
- Representing Proportions: Percentages are vital for representing proportions in statistical data. If 25% of respondents in a survey chose a particular option, it clearly shows the relative popularity of that option.
- Charts and Graphs: Pie charts and bar graphs frequently use percentages to visually represent parts of a whole. A quarter-segment in a pie chart immediately communicates a 25% proportion.
- Probability: In probability calculations, a 25% chance of an event occurring means that there's a one in four probability of that event happening.
3. Everyday Life Applications:
- Recipe Scaling: If a recipe calls for a quarter cup of an ingredient, you know that it represents 25% of a full cup.
- Time Management: A quarter of an hour is 15 minutes (25% of 60 minutes).
- Distance Measurement: If you need to travel a quarter of a mile, you know it's 25% of a mile.
Beyond One-Quarter: Expanding the Concept
While this article focuses on "a quarter," understanding this concept empowers you to work with other fractions and their percentage equivalents. By applying the same principles of converting fractions to decimals and then to percentages, you can easily calculate the percentage representation of any fraction.
For instance:
- One-half (1/2): 1 ÷ 2 = 0.5; 0.5 x 100% = 50%
- Three-quarters (3/4): 3 ÷ 4 = 0.75; 0.75 x 100% = 75%
- One-fifth (1/5): 1 ÷ 5 = 0.2; 0.2 x 100% = 20%
Mastering Percentage Calculations: Tips and Tricks
Efficiently handling percentage calculations is vital. Here are some helpful tips:
- Memorize key percentage equivalents: Familiarizing yourself with common fraction-percentage equivalents (e.g., 1/2 = 50%, 1/4 = 25%, 1/5 = 20%, etc.) significantly speeds up calculations.
- Use calculators strategically: For complex calculations, utilizing a calculator enhances accuracy and efficiency.
- Practice regularly: Consistent practice is key to mastering percentage calculations. Work through various examples to build your confidence and understanding.
- Break down complex problems: When faced with challenging percentage problems, break them down into smaller, manageable steps.
Conclusion: The Significance of Understanding Percentages
Understanding the relationship between fractions and percentages, particularly the equivalence of a quarter to 25%, is a crucial skill with wide-ranging applications. From handling personal finances to analyzing data and comprehending statistical information, this knowledge empowers informed decision-making and enhances problem-solving abilities across various aspects of life. By mastering these concepts, you equip yourself with a valuable tool for navigating the numerical world with confidence and clarity. Remember to practice regularly and utilize the tips provided to further solidify your understanding and enhance your calculation skills. The more you practice, the easier and more intuitive these conversions will become.
Latest Posts
Latest Posts
-
What Does A Positive Delta H Mean
Mar 31, 2025
-
What Holds An Ionic Bond Together
Mar 31, 2025
-
Genotype And Phenotype Punnett Square Examples
Mar 31, 2025
-
Equation Relating Electric Field And Voltage
Mar 31, 2025
-
Does A Plant Cell Have Dna
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is A Quarter In Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
