Why Is The Electric Field Inside A Conductor Zero
Muz Play
Mar 29, 2025 · 5 min read
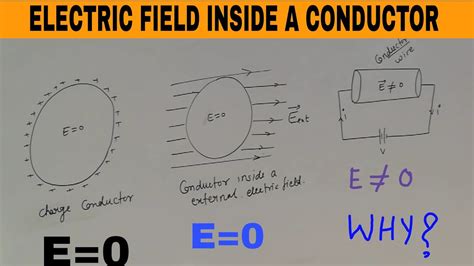
Table of Contents
Why is the Electric Field Inside a Conductor Zero?
Understanding why the electric field inside a conductor is zero is fundamental to comprehending electrostatics and the behavior of materials in the presence of electric fields. This principle underpins many crucial applications in electronics, electromagnetism, and physics. This article will delve deep into the reasons behind this phenomenon, exploring the underlying concepts and providing a comprehensive explanation.
The Nature of Conductors
Before diving into the core question, let's establish a clear understanding of what constitutes a conductor. A conductor is a material that allows the free movement of electric charge. This free movement is facilitated by the presence of loosely bound electrons, often referred to as free electrons, within the material's atomic structure. Metals, like copper, silver, and gold, are excellent examples of conductors due to their abundance of these mobile electrons.
The Role of Free Electrons
These free electrons are not bound to any particular atom and can easily drift throughout the material. This mobility is crucial in understanding the electric field behavior within a conductor. When an external electric field is applied, these free electrons experience a force and begin to move, creating an electric current.
The Static Equilibrium Condition
The key to understanding why the electric field inside a conductor is zero lies in the concept of static equilibrium. In a static situation, meaning no net flow of charge, the charges within the conductor redistribute themselves until the net electric force on each electron is zero. This is a state of equilibrium, and it's essential for the electric field inside to vanish.
Charge Redistribution and its effects:
When a conductor is placed in an external electric field, the free electrons initially experience a force pushing them in one direction. This movement of charges creates an internal electric field that opposes the external field. This opposing field is generated by the accumulation of excess charge on the surface of the conductor. The process continues until the internal field precisely cancels the external field within the conductor, resulting in a net electric field of zero.
This redistribution of charges is a crucial self-regulating mechanism. If there were a net electric field inside the conductor, the free electrons would continue to move until this field is neutralized. This dynamic equilibrium is what guarantees the absence of an electric field in the conductor's interior under static conditions.
Mathematical Proof: Gauss's Law
Gauss's law provides a powerful mathematical framework to demonstrate the absence of an electric field inside a conductor in electrostatic equilibrium. Gauss's law states that the flux of the electric field through any closed surface is proportional to the enclosed charge.
Mathematically, it's expressed as:
∮ E • dA = Q<sub>enc</sub> / ε₀
where:
- E is the electric field vector
- dA is a differential area vector
- Q<sub>enc</sub> is the enclosed charge
- ε₀ is the permittivity of free space
Applying Gauss's Law to a Conductor:
Consider a Gaussian surface entirely within a conductor. Because the conductor is in electrostatic equilibrium, there is no net flow of charge, and therefore no net current. This implies that there is no charge within the Gaussian surface. Consequently, Q<sub>enc</sub> = 0. Therefore, Gauss's law simplifies to:
∮ E • dA = 0
This equation implies that the electric field inside the conductor must be zero. If there were any electric field, the integral would be non-zero, contradicting the law. This mathematical proof rigorously supports the assertion that the electric field within a conductor in electrostatic equilibrium is indeed zero.
Implications and Exceptions
The zero electric field inside a conductor has profound implications for various aspects of physics and engineering:
Shielding Effect (Faraday Cage):
This principle is the basis behind the Faraday cage, a structure of conductive material that shields its interior from external electric fields. This is because the charges in the conductor redistribute themselves to cancel out any external field, creating a region of zero electric field within. This has applications in protecting sensitive electronics from electromagnetic interference (EMI) and lightning strikes.
Electrostatic Potential:
Inside a conductor in electrostatic equilibrium, the electric potential (V) is constant. Since the electric field is the negative gradient of the potential (E = -∇V), a zero electric field implies a constant potential. This means all points within the conductor are at the same electric potential.
Charge Distribution on the Surface:
All excess charge in a conductor resides on its surface. This is a direct consequence of the zero electric field inside. If charge existed within the conductor, it would create an internal electric field, contradicting the equilibrium condition.
Cases Where the Electric Field Might Not Be Zero:
While the zero electric field inside a conductor is a crucial principle, there are situations where this might not strictly hold true:
Non-static Conditions:
If the charge distribution is changing (e.g., an alternating current is flowing), then the electric field inside the conductor is no longer zero. The movement of charges creates a time-varying magnetic field, which, according to Faraday's law of induction, induces an electric field.
High-Frequency Fields:
At very high frequencies, the skin effect becomes significant. The skin effect is the phenomenon where alternating currents tend to flow near the surface of a conductor, reducing the effective cross-sectional area for current flow. This means that the electric field is not strictly zero throughout the conductor's entire volume, though it remains significantly reduced in the inner regions.
Superconductors:
Superconductors exhibit unique properties at extremely low temperatures. While the electric field is typically zero inside a conductor in equilibrium, the behavior of a superconductor is different due to the absence of electrical resistance.
Non-uniform conductors:
If the conductor has a non-uniform shape or composition, the electric field might not be exactly zero everywhere inside, although it remains significantly diminished compared to the external field.
Conclusion:
The principle that the electric field inside a conductor is zero under static conditions is a cornerstone of electrostatics. It is derived from the concept of static equilibrium, confirmed by Gauss's law, and manifests in various practical applications, including Faraday cages. Understanding this principle is essential for grasping the behavior of conductors in electric fields and its wider implications across various fields of science and engineering. While exceptions exist under non-static or high-frequency conditions, the fundamental principle remains crucial for analyzing and predicting the behavior of conductors in most everyday scenarios.
Latest Posts
Latest Posts
-
Clusters Of Neuron Cell Bodies In The Pns Are Called
Mar 31, 2025
-
Solving Square Root And Other Radical Equations
Mar 31, 2025
-
What Are Characteristics Of A Virus
Mar 31, 2025
-
The Adrenal Glands Are Attached Superiorly To Which Organ
Mar 31, 2025
-
The Set Of Ordered Pairs That Defines The Relation
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Why Is The Electric Field Inside A Conductor Zero . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
