How Do You Solve Linear Equations With Fractions
Muz Play
Mar 28, 2025 · 6 min read
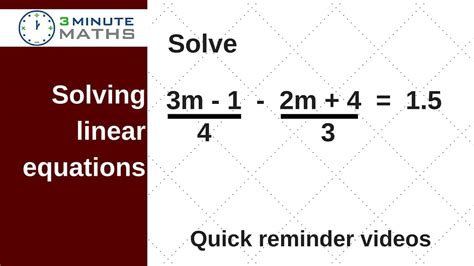
Table of Contents
How to Solve Linear Equations with Fractions: A Comprehensive Guide
Linear equations are fundamental to algebra, and mastering them is crucial for success in mathematics. While solving linear equations without fractions is relatively straightforward, the presence of fractions can often introduce a layer of complexity that can be daunting for many students. This comprehensive guide will demystify the process, providing you with a step-by-step approach to confidently solve linear equations containing fractions. We'll cover various techniques, examples, and helpful tips to solidify your understanding.
Understanding the Basics: What are Linear Equations?
Before delving into equations with fractions, let's briefly review the concept of linear equations. A linear equation is an algebraic equation in which the highest power of the variable (usually 'x') is 1. These equations represent a straight line when graphed. They typically involve constants, variables, and arithmetic operations like addition, subtraction, multiplication, and division. A simple example is: 2x + 3 = 7.
Why Fractions Appear in Linear Equations
Fractions in linear equations often arise from real-world problems and scenarios. They might represent parts of a whole, rates, ratios, or proportions. For instance, if a problem involves dividing a quantity, the result could be a fraction that needs to be incorporated into the equation.
Methods for Solving Linear Equations with Fractions
There are two primary methods for solving linear equations containing fractions:
1. The Least Common Multiple (LCM) Method
This method involves eliminating fractions by multiplying the entire equation by the least common multiple (LCM) of all the denominators present. This step clears the fractions, leaving you with a simpler equation to solve.
Steps:
- Identify the denominators: Determine all the denominators present in the equation.
- Find the LCM: Calculate the least common multiple of these denominators. This is the smallest number that is a multiple of all the denominators.
- Multiply the entire equation by the LCM: Multiply every term in the equation (on both sides of the equals sign) by the LCM. This will eliminate the fractions.
- Solve the resulting equation: Solve the simplified equation using standard techniques for solving linear equations (like combining like terms, isolating the variable, etc.).
- Check your solution: Substitute your solution back into the original equation to verify its accuracy.
Example:
Solve the equation: (1/2)x + (2/3) = (5/6)x - 1
-
Denominators: 2, 3, 6
-
LCM: The LCM of 2, 3, and 6 is 6.
-
Multiply by LCM: Multiply the entire equation by 6:
6 * [(1/2)x + (2/3)] = 6 * [(5/6)x - 1]
This simplifies to: 3x + 4 = 5x - 6
-
Solve: Subtract 3x from both sides: 4 = 2x - 6. Add 6 to both sides: 10 = 2x. Divide by 2: x = 5.
-
Check: Substitute x = 5 into the original equation: (1/2)(5) + (2/3) = (5/6)(5) - 1. This simplifies to (5/2) + (2/3) = (25/6) - 1, which further simplifies to (19/6) = (19/6). The solution is correct.
2. The Fraction Manipulation Method
This method involves manipulating the fractions directly within the equation without initially clearing them. While often less efficient than the LCM method, it can be useful for building a stronger understanding of fraction arithmetic.
Steps:
- Combine like terms: Group similar terms (those with 'x' and those without 'x') on either side of the equation. This might involve adding or subtracting fractions.
- Isolate the variable term: Move all terms containing the variable ('x') to one side of the equation and all constant terms to the other side. Remember to perform the necessary addition, subtraction, multiplication, or division with fractions.
- Solve for x: Perform the necessary operations (addition, subtraction, multiplication, or division of fractions) to isolate 'x' and find its value.
- Check your solution: Substitute your solution back into the original equation to ensure its correctness.
Example:
Solve the equation: (1/4)x - (1/2) = (3/8)x + 1
-
Combine Like Terms (Partially): We'll subtract (3/8)x from both sides:
(1/4)x - (3/8)x - (1/2) = 1
-
Simplify Fraction with 'x': Find a common denominator for (1/4) and (3/8):
(2/8)x - (3/8)x - (1/2) = 1 (-1/8)x - (1/2) = 1
-
Isolate 'x': Add (1/2) to both sides:
(-1/8)x = 1 + (1/2) = (3/2)
-
Solve for x: Multiply both sides by -8:
x = (3/2) * (-8) = -12
-
Check: Substitute x = -12 into the original equation: (1/4)(-12) - (1/2) = (3/8)(-12) + 1. This simplifies to -3 - (1/2) = -9/2 + 1, which further simplifies to -7/2 = -7/2. The solution is correct.
Advanced Techniques and Complex Scenarios
While the LCM and fraction manipulation methods cover most cases, some more complex scenarios might require additional strategies:
Equations with nested fractions:
These equations contain fractions within fractions. The best approach is often to simplify the nested fractions first by using fraction division or multiplication rules before applying the LCM or fraction manipulation method.
Example: x / (1 + (1/x)) = 2
Equations with variables in denominators:
In equations like (2/x) + 1 = (3/2x), you must restrict the possible solutions to exclude values of x that would make any denominator zero (in this case, x ≠ 0). Then, you can apply the LCM method or other methods to solve for x. After finding a solution, verify it's not a restricted value.
Systems of linear equations with fractions:
If you have multiple equations with fractions, you can use either substitution or elimination methods. The LCM method is extremely helpful to simplify the equations before applying these methods.
Tips for Success
- Practice regularly: Consistent practice is key to mastering these techniques. Work through various examples to solidify your understanding.
- Check your work: Always substitute your solution back into the original equation to verify its correctness. This prevents careless errors.
- Use a calculator strategically: A calculator can assist with the arithmetic, but try to understand the underlying mathematical concepts first. Over-reliance on a calculator can hinder your understanding.
- Break down complex problems: If an equation seems overwhelming, break it down into smaller, manageable steps.
- Focus on understanding: Focus on understanding the why behind each step, not just the how. This will enhance your problem-solving skills and make you a more confident mathematician.
Conclusion
Solving linear equations with fractions might seem intimidating at first, but with consistent practice and a solid understanding of the LCM method and fraction manipulation, you can confidently tackle these problems. Remember to always check your solutions and break down complex problems into smaller, manageable steps. By mastering these techniques, you'll build a strong foundation for more advanced algebraic concepts. The ability to handle fractions seamlessly is a cornerstone of algebraic proficiency, opening doors to more complex mathematical explorations.
Latest Posts
Latest Posts
-
Why Do Fats Store More Energy Than Carbohydrates
Mar 31, 2025
-
What Is The Magnification Of The Ocular Lenses
Mar 31, 2025
-
The Ballard Scoring System Evaluates Newborns On Which Two Factors
Mar 31, 2025
-
Moment Of Inertia Of A Circle Formula
Mar 31, 2025
-
What Is End Point In Titration
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How Do You Solve Linear Equations With Fractions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
