How To Calculate Voltage Of A Cell
Muz Play
Apr 01, 2025 · 6 min read
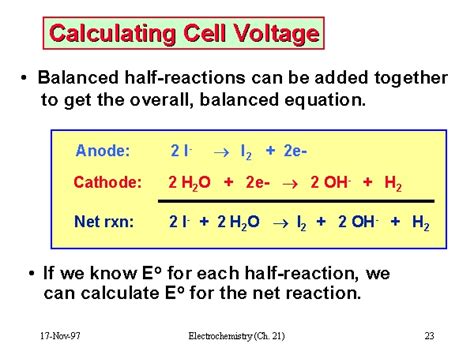
Table of Contents
How to Calculate the Voltage of a Cell: A Comprehensive Guide
Understanding how to calculate the voltage of a cell is fundamental to comprehending basic electricity and electronics. This guide will delve into the various methods and factors influencing the voltage of different types of cells, from simple electrochemical cells to more complex batteries. We’ll cover everything from the Nernst equation to practical applications and troubleshooting.
Understanding Cell Voltage: The Basics
Before diving into calculations, it's crucial to grasp the underlying principles. The voltage of a cell, also known as its electromotive force (EMF), is the potential difference between its two electrodes (anode and cathode). This potential difference arises from the difference in the electrochemical potential of the two half-cells. This difference drives the flow of electrons, creating an electric current when a circuit is completed.
The voltage of a cell is determined by several factors, including:
- The type of electrode materials: Different materials have different standard reduction potentials. The greater the difference in these potentials, the higher the cell voltage. This is a key concept explained in more detail below.
- The concentration of electrolytes: The concentration of ions in the electrolyte solution directly impacts the cell's voltage. This is described by the Nernst equation.
- Temperature: Temperature affects the kinetics of the electrochemical reactions, influencing the cell's voltage. Higher temperatures generally lead to increased voltage, but this is dependent on the specific cell chemistry.
- Internal resistance: The internal resistance of the cell causes a voltage drop when current flows. This is a significant factor when considering the voltage available at the cell's terminals under load.
Calculating Voltage using Standard Reduction Potentials
The most straightforward method to calculate the theoretical voltage of a cell involves using standard reduction potentials. These potentials represent the tendency of a species to gain electrons and are measured relative to a standard hydrogen electrode (SHE), which is assigned a potential of 0 volts. Standard reduction potentials are usually tabulated and readily available in chemistry textbooks and online resources.
The calculation is based on the following equation:
E°<sub>cell</sub> = E°<sub>cathode</sub> - E°<sub>anode</sub>
Where:
- E°<sub>cell</sub> is the standard cell potential (voltage)
- E°<sub>cathode</sub> is the standard reduction potential of the cathode (the electrode where reduction occurs)
- E°<sub>anode</sub> is the standard reduction potential of the anode (the electrode where oxidation occurs)
Important Note: Remember to use the reduction potentials as they are listed in the table. Do not change the sign if the reaction is an oxidation reaction (occurring at the anode). The formula subtracts the anode potential to account for this.
Example:
Consider a galvanic cell consisting of a zinc anode (Zn) and a copper cathode (Cu) in their respective sulfate solutions. The standard reduction potentials are:
- Zn<sup>2+</sup> + 2e<sup>-</sup> → Zn(s) E° = -0.76 V
- Cu<sup>2+</sup> + 2e<sup>-</sup> → Cu(s) E° = +0.34 V
In this cell, zinc undergoes oxidation (anode), and copper undergoes reduction (cathode). Therefore, the cell potential is:
E°<sub>cell</sub> = E°<sub>cathode</sub> - E°<sub>anode</sub> = (+0.34 V) - (-0.76 V) = 1.10 V
This calculation provides the theoretical maximum voltage the cell can produce under standard conditions (25°C, 1 atm pressure, 1 M concentration of ions).
The Nernst Equation: Accounting for Non-Standard Conditions
The standard reduction potential calculations only apply under ideal, standard conditions. In real-world scenarios, the concentrations of ions and the temperature may deviate, significantly impacting the cell's voltage. The Nernst equation addresses this by incorporating these variations:
E<sub>cell</sub> = E°<sub>cell</sub> - (RT/nF)lnQ
Where:
- E<sub>cell</sub> is the cell potential under non-standard conditions
- E°<sub>cell</sub> is the standard cell potential
- R is the ideal gas constant (8.314 J/mol·K)
- T is the temperature in Kelvin
- n is the number of moles of electrons transferred in the balanced redox reaction
- F is the Faraday constant (96485 C/mol)
- Q is the reaction quotient
The reaction quotient (Q) is calculated similarly to the equilibrium constant (K) but uses the current concentrations of reactants and products, not the equilibrium concentrations.
Example:
Let's consider the same Zn-Cu cell but with non-standard concentrations. Suppose the concentration of Zn<sup>2+</sup> is 0.1 M and the concentration of Cu<sup>2+</sup> is 1 M. Assume the temperature is 298 K (25°C). The reaction quotient Q is:
Q = [Zn<sup>2+</sup>]/[Cu<sup>2+</sup>] = 0.1/1 = 0.1
Applying the Nernst equation:
E<sub>cell</sub> = 1.10 V - (8.314 J/mol·K * 298 K / (2 * 96485 C/mol)) * ln(0.1) ≈ 1.13 V
Notice that the non-standard conditions result in a slightly higher cell voltage than the standard potential.
Different Types of Cells and their Voltage Calculations
The methods described above apply primarily to simple galvanic cells. However, the voltage calculation can become more complex for other cell types:
1. Batteries: Series and Parallel Connections
Batteries are composed of multiple cells connected either in series or parallel.
- Series Connection: The voltage of cells connected in series adds up. If you have 'x' number of identical cells each with voltage 'V', the total voltage is xV.
- Parallel Connection: The voltage remains the same as a single cell, but the current capacity increases.
2. Fuel Cells: A Dynamic Voltage
Fuel cells generate electricity through the electrochemical reaction of fuel and an oxidant. The voltage depends on the type of fuel and oxidant, their flow rates, and operating conditions. A precise calculation often involves complex thermodynamic modeling beyond the scope of this basic guide.
3. Solar Cells: Light-Dependent Voltage
Solar cells generate voltage through the photovoltaic effect when photons strike a semiconductor material. The voltage depends on the material's bandgap, light intensity, and temperature. Precise calculation is again more complex and involves detailed semiconductor physics.
Practical Considerations and Troubleshooting
Calculating the theoretical voltage is only one part of the equation. In practice, several factors can affect the actual voltage measured across the terminals of a cell or battery:
- Internal Resistance: As mentioned earlier, internal resistance causes voltage drop under load. The higher the current drawn, the greater the voltage drop. This is given by Ohm's Law: V<sub>drop</sub> = I * R<sub>internal</sub>
- Age and Degradation: Over time, cells and batteries degrade, reducing their capacity and voltage output.
- Temperature Effects: Extreme temperatures can affect the chemical reactions within a cell, impacting its voltage.
- Self-Discharge: Even when not in use, cells and batteries slowly lose charge due to self-discharge.
To measure the actual voltage of a cell, you should use a voltmeter, ideally a high-impedance voltmeter to minimize current draw and ensure accurate reading. If the measured voltage is significantly lower than the expected theoretical value, consider the above factors as potential causes.
Conclusion: A Practical Approach to Cell Voltage
Calculating the voltage of a cell involves understanding basic electrochemical principles, utilizing standard reduction potentials, and, where necessary, applying the Nernst equation to account for non-standard conditions. While calculating the theoretical voltage provides a valuable starting point, it's crucial to remember that practical considerations such as internal resistance, age, temperature, and self-discharge can significantly affect the actual measured voltage. By considering both theoretical calculations and practical factors, you can gain a comprehensive understanding of cell voltage and its implications in various applications. Remember to always prioritize safety when working with electrical components and cells.
Latest Posts
Latest Posts
-
Liquids Have A Definite Shape And Volume
Apr 02, 2025
-
Difference Between Molar Mass And Atomic Mass
Apr 02, 2025
-
Write In Standard Form The Equation Of Each Line
Apr 02, 2025
-
What Does Fad Stand For In Biology
Apr 02, 2025
-
The Outermost Layer Of The Heart Is Called The
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How To Calculate Voltage Of A Cell . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
