How To Do Mass Mass Stoichiometry
Muz Play
Apr 04, 2025 · 6 min read
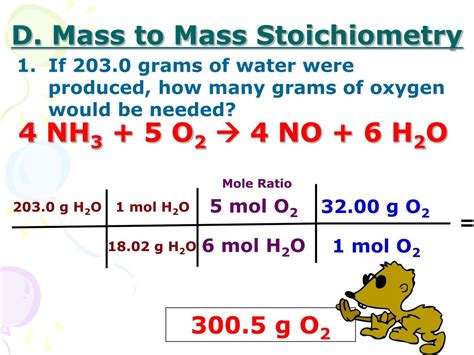
Table of Contents
Mastering Mass-Mass Stoichiometry: A Comprehensive Guide
Mass-mass stoichiometry problems are a cornerstone of chemistry, allowing us to predict the amounts of reactants needed or products formed in a chemical reaction based on mass. While seemingly daunting at first, mastering this skill involves a systematic approach combining balanced chemical equations and molar masses. This comprehensive guide will break down the process step-by-step, equipping you with the tools and understanding to confidently tackle any mass-mass stoichiometry problem.
Understanding the Fundamentals
Before diving into complex calculations, let's solidify our understanding of the underlying concepts:
1. Balanced Chemical Equations: The Roadmap
A balanced chemical equation is the foundation of any stoichiometry problem. It provides the crucial mole ratios between reactants and products. A balanced equation ensures the Law of Conservation of Mass is upheld, meaning the number of atoms of each element remains constant throughout the reaction. For example:
2H₂ + O₂ → 2H₂O
This equation tells us that two moles of hydrogen gas (H₂) react with one mole of oxygen gas (O₂) to produce two moles of water (H₂O). Without a balanced equation, your calculations will be inherently flawed.
2. Molar Mass: The Bridge Between Grams and Moles
Molar mass is the mass of one mole of a substance, expressed in grams per mole (g/mol). It's the crucial link connecting the macroscopic world of grams to the microscopic world of moles, allowing us to convert between mass and the number of particles. You can find molar masses on the periodic table or calculate them by adding the atomic masses of all atoms in a molecule. For example, the molar mass of water (H₂O) is approximately 18.02 g/mol (2 x 1.01 g/mol for H + 16.00 g/mol for O).
3. Mole Ratios: The Key to Conversion
The coefficients in a balanced chemical equation represent the mole ratios of reactants and products. These ratios are essential for converting between the moles of one substance and the moles of another in the reaction. In the example above, the mole ratio of H₂ to O₂ is 2:1, and the mole ratio of H₂ to H₂O is 2:2 (or 1:1).
The Step-by-Step Process: Solving Mass-Mass Stoichiometry Problems
Let's outline the systematic approach for solving mass-mass stoichiometry problems. We'll use the following example:
Problem: How many grams of water (H₂O) are produced when 4.0 grams of hydrogen gas (H₂) react completely with excess oxygen gas (O₂)?
Steps:
-
Write and balance the chemical equation:
2H₂ + O₂ → 2H₂O
-
Convert grams of the given substance to moles:
-
We start with 4.0 grams of H₂. Using the molar mass of H₂ (approximately 2.02 g/mol), we convert grams to moles:
(4.0 g H₂) x (1 mol H₂ / 2.02 g H₂) ≈ 1.98 mol H₂
-
-
Use the mole ratio from the balanced equation to convert moles of the given substance to moles of the desired substance:
-
From the balanced equation, the mole ratio of H₂ to H₂O is 1:1. Therefore:
(1.98 mol H₂) x (2 mol H₂O / 2 mol H₂) = 1.98 mol H₂O
-
-
Convert moles of the desired substance to grams:
-
Using the molar mass of H₂O (approximately 18.02 g/mol), we convert moles to grams:
(1.98 mol H₂O) x (18.02 g H₂O / 1 mol H₂O) ≈ 35.7 g H₂O
-
Therefore, approximately 35.7 grams of water are produced.
Advanced Techniques and Considerations
1. Limiting Reactants: Identifying the Bottleneck
In many real-world scenarios, reactants are not present in the stoichiometrically ideal ratios indicated by the balanced equation. One reactant will be completely consumed before others, limiting the amount of product formed. This reactant is called the limiting reactant.
To determine the limiting reactant, you'll perform mass-mass stoichiometry calculations for each reactant. The reactant producing the least amount of product is the limiting reactant, and its calculated product amount represents the actual yield of the reaction.
2. Percent Yield: Accounting for Reality
The theoretical yield is the amount of product predicted by stoichiometry calculations, assuming 100% reaction efficiency. However, in practice, reactions rarely achieve 100% yield due to various factors like incomplete reactions, side reactions, or loss during product purification. The percent yield accounts for this discrepancy:
Percent Yield = (Actual Yield / Theoretical Yield) x 100%
The actual yield is the experimentally measured amount of product obtained.
3. Dealing with More Complex Reactions
The principles remain the same for more complex reactions involving multiple steps or more than two reactants. You'll simply need to perform multiple stoichiometry calculations sequentially, always carefully considering mole ratios and limiting reactants.
4. Importance of Significant Figures
Pay close attention to significant figures throughout your calculations. Your final answer should reflect the precision of your measurements and calculations.
Practical Applications and Real-World Examples
Mass-mass stoichiometry is not just a theoretical exercise; it's a crucial tool in numerous fields:
- Industrial Chemistry: Optimizing production processes, determining the required amounts of raw materials, and predicting product yields in large-scale manufacturing.
- Environmental Science: Assessing pollutant levels, determining the amount of pollutants needed to neutralize a spill, and predicting the environmental impact of chemical reactions.
- Pharmaceutical Industry: Calculating drug dosages, formulating medications, and ensuring the purity and efficacy of pharmaceutical products.
- Food Science: Determining ingredient ratios in food processing, optimizing nutritional value, and ensuring food safety.
Troubleshooting Common Mistakes
- Forgetting to balance the chemical equation: This is the most common and critical error. Always ensure your chemical equation is balanced before beginning any calculations.
- Incorrectly using mole ratios: Double-check that you are using the correct mole ratios from the balanced equation to convert between moles of different substances.
- Errors in unit conversions: Carefully convert grams to moles and vice versa using the correct molar masses.
- Neglecting significant figures: Pay attention to significant figures throughout the calculation to ensure the final answer reflects the appropriate precision.
- Ignoring limiting reactants: When dealing with multiple reactants, always identify the limiting reactant to determine the actual yield.
Conclusion: Mastering the Art of Mass-Mass Stoichiometry
Mass-mass stoichiometry is a fundamental skill in chemistry with wide-ranging applications. By understanding the underlying concepts – balanced equations, molar masses, and mole ratios – and following the systematic step-by-step process, you can confidently solve even the most challenging stoichiometry problems. Remember to practice regularly, pay close attention to detail, and always double-check your work. With consistent effort, you'll master this essential skill and gain a deeper understanding of chemical reactions and their quantitative relationships.
Latest Posts
Latest Posts
-
Based On The Frequency Distribution Above Is 22 5 A
Apr 04, 2025
-
Dna Coloring Transcription And Translation Answer Key
Apr 04, 2025
-
What Organelle Is Found Only In Animal Cells
Apr 04, 2025
-
Family Developmental And Life Cycle Theory
Apr 04, 2025
-
What Are The Reactants Of A Neutralization Reaction
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about How To Do Mass Mass Stoichiometry . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
