Is Delta H Products Minus Reactants
Muz Play
Mar 30, 2025 · 6 min read
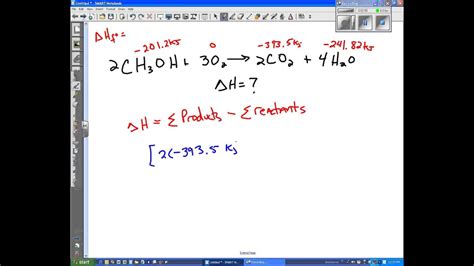
Table of Contents
Is ΔH = Products – Reactants? Understanding Enthalpy Change in Chemical Reactions
The statement "ΔH = Products – Reactants" is a concise and often-used summary of how to calculate the enthalpy change (ΔH) in a chemical reaction. While fundamentally correct, a deeper understanding requires exploring the nuances of this equation and the concepts surrounding enthalpy. This article will delve into the intricacies of enthalpy change, clarifying its meaning, calculation, and application in various chemical processes. We'll explore standard enthalpy changes of formation and combustion, and how these contribute to our understanding of reaction energetics.
Understanding Enthalpy and Enthalpy Change (ΔH)
Enthalpy (H) is a thermodynamic state function representing the total heat content of a system at constant pressure. It's a crucial concept in chemistry because it directly relates to the heat absorbed or released during a chemical reaction. Enthalpy change (ΔH), often simply called the heat of reaction, quantifies this energy transfer. A positive ΔH indicates an endothermic reaction (heat is absorbed from the surroundings), while a negative ΔH signifies an exothermic reaction (heat is released to the surroundings).
The equation ΔH = Products – Reactants refers to the difference in enthalpy between the products and reactants of a chemical reaction. More accurately, it represents the difference in the standard enthalpy of formation of the products and the reactants:
ΔH°<sub>rxn</sub> = Σ [ΔH°<sub>f</sub>(products)] – Σ [ΔH°<sub>f</sub>(reactants)]
where:
- ΔH°<sub>rxn</sub> is the standard enthalpy change of reaction. The "°" symbol denotes standard conditions (usually 298 K and 1 atm pressure).
- Σ represents the sum of the enthalpies of formation of all the products and reactants.
- ΔH°<sub>f</sub> represents the standard enthalpy of formation.
Standard Enthalpy of Formation (ΔH°<sub>f</sub>)
The standard enthalpy of formation (ΔH°<sub>f</sub>) is the enthalpy change when one mole of a substance is formed from its constituent elements in their standard states under standard conditions. It's a crucial value used in calculating the enthalpy change of a reaction. The standard enthalpy of formation for elements in their standard states is defined as zero.
For example, the standard enthalpy of formation of water (H₂O) is the enthalpy change for the reaction:
H₂(g) + ½O₂(g) → H₂O(l)
The standard enthalpy of formation for many compounds is readily available in thermodynamic data tables. These tables are essential for calculating the enthalpy changes of reactions.
Calculating ΔH using Standard Enthalpies of Formation
Let's illustrate the calculation of ΔH using the combustion of methane:
CH₄(g) + 2O₂(g) → CO₂(g) + 2H₂O(l)
To calculate ΔH°<sub>rxn</sub>, we need the standard enthalpies of formation for each compound:
- ΔH°<sub>f</sub>(CH₄(g)) = -74.8 kJ/mol
- ΔH°<sub>f</sub>(O₂(g)) = 0 kJ/mol (element in standard state)
- ΔH°<sub>f</sub>(CO₂(g)) = -393.5 kJ/mol
- ΔH°<sub>f</sub>(H₂O(l)) = -285.8 kJ/mol
Now, we apply the equation:
ΔH°<sub>rxn</sub> = [ΔH°<sub>f</sub>(CO₂(g)) + 2ΔH°<sub>f</sub>(H₂O(l))] – [ΔH°<sub>f</sub>(CH₄(g)) + 2ΔH°<sub>f</sub>(O₂(g))]
ΔH°<sub>rxn</sub> = [(-393.5 kJ/mol) + 2(-285.8 kJ/mol)] – [(-74.8 kJ/mol) + 2(0 kJ/mol)]
ΔH°<sub>rxn</sub> = -890.1 kJ/mol
This indicates that the combustion of one mole of methane releases 890.1 kJ of heat, making it a highly exothermic reaction.
Standard Enthalpy of Combustion (ΔH°<sub>c</sub>)
The standard enthalpy of combustion (ΔH°<sub>c</sub>) is a specific type of enthalpy change representing the heat released when one mole of a substance undergoes complete combustion in excess oxygen under standard conditions. It's particularly useful for determining the energy content of fuels.
For example, the combustion of ethane (C₂H₆):
2C₂H₆(g) + 7O₂(g) → 4CO₂(g) + 6H₂O(l)
The standard enthalpy of combustion of ethane can be calculated using the standard enthalpies of formation of the reactants and products in a manner similar to the example above. However, ΔH°<sub>c</sub> values are often directly available in thermodynamic data tables, making calculations easier.
Hess's Law and Enthalpy Changes
Hess's Law states that the total enthalpy change for a reaction is independent of the pathway taken. This is incredibly useful because it allows us to calculate the enthalpy change of reactions that are difficult or impossible to measure directly. By combining known enthalpy changes of other reactions, we can determine the enthalpy change of the target reaction. This is particularly valuable for reactions that occur in multiple steps.
Example: Consider a reaction that can be broken down into several steps. By summing the enthalpy changes of these individual steps, one can find the overall enthalpy change of the main reaction.
Limitations and Considerations
While the equation ΔH = Products – Reactants provides a straightforward method for calculating enthalpy changes, it's essential to acknowledge some limitations:
- Standard Conditions: The equation, as presented above, is strictly applicable under standard conditions (298 K and 1 atm). Deviations from standard conditions will affect the enthalpy change.
- Accuracy of Data: The accuracy of the calculated ΔH depends heavily on the accuracy of the standard enthalpies of formation used. Slight variations in these values from different sources can lead to minor discrepancies in the final result.
- Phase Changes: The physical state (solid, liquid, gas) of reactants and products must be specified, as enthalpy changes vary significantly between phases.
- Complex Reactions: For complex reactions involving multiple steps or intermediate species, the simple subtraction method might not be directly applicable. More advanced techniques, such as Hess's Law, might be necessary.
- Temperature Dependence: Enthalpy changes are temperature-dependent. The values used in calculations are usually specific to a particular temperature, often 298 K.
Applications of Enthalpy Change Calculations
The ability to calculate and understand enthalpy changes has widespread applications in various fields:
- Chemical Engineering: Designing and optimizing industrial processes, particularly those involving exothermic or endothermic reactions, requires precise enthalpy calculations.
- Materials Science: Determining the stability and reactivity of materials often involves analyzing their enthalpy of formation and other related thermodynamic properties.
- Environmental Science: Assessing the energy released or absorbed in environmental processes, such as combustion and biological reactions, provides valuable insights into global climate change and environmental impact assessments.
- Biochemistry: Understanding the energetics of biological reactions is fundamental to studying metabolism, enzyme kinetics, and other aspects of cellular processes.
Conclusion
The equation ΔH = Products – Reactants (more accurately, the equation using standard enthalpies of formation) is a powerful tool for calculating enthalpy changes in chemical reactions. While relatively straightforward, a comprehensive understanding of enthalpy, standard enthalpies of formation and combustion, and Hess's Law is crucial for accurate and meaningful applications. Remember that standard conditions, data accuracy, and phase considerations are essential factors to consider when performing these calculations. Mastering these principles provides a strong foundation for tackling more complex thermodynamic problems and gaining a deeper appreciation for the energetics governing chemical transformations.
Latest Posts
Latest Posts
-
Integration By Parts How To Choose U And Dv
Apr 01, 2025
-
Protein Synthesis Takes Place In The
Apr 01, 2025
-
Microscopic Anatomy Of A Muscle Fiber
Apr 01, 2025
-
What Is The General Equation For Cellular Respiration
Apr 01, 2025
-
Are Influence Lines In The Fe Exam
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Is Delta H Products Minus Reactants . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
