Packing Efficiency Of Body Centered Cubic
Muz Play
Mar 31, 2025 · 6 min read
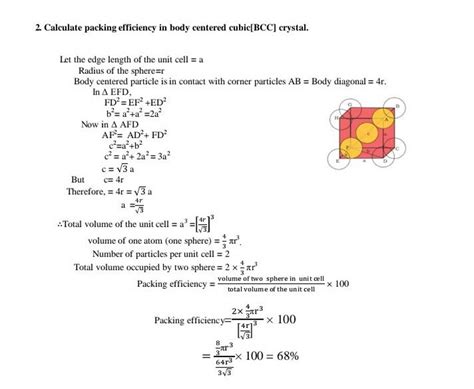
Table of Contents
Packing Efficiency of Body-Centered Cubic (BCC) Structures
The arrangement of atoms in a crystal lattice significantly impacts its physical properties, such as density, strength, and conductivity. One common crystal structure is the body-centered cubic (BCC) structure, found in many metals like iron, chromium, and tungsten. Understanding the packing efficiency of this structure is crucial for comprehending its macroscopic behavior. This article delves into the detailed calculation and implications of BCC packing efficiency.
What is a Body-Centered Cubic (BCC) Structure?
A body-centered cubic structure is a type of cubic crystal structure where atoms are located at the corners of a cube and one atom is situated at the center of the cube. This central atom is equidistant from all eight corner atoms. Each corner atom is shared by eight adjacent unit cells, while the central atom belongs exclusively to the unit cell.
Key Characteristics of BCC:
- Coordination Number: Each atom in a BCC structure has eight nearest neighbors (the eight corner atoms of the cube). This is its coordination number.
- Number of Atoms per Unit Cell: There are a total of two atoms per unit cell in a BCC structure: ⅛ atom at each of the eight corners (⅛ x 8 = 1 atom) and one atom at the center.
- Atomic Packing Factor (APF): This is the fraction of the unit cell volume that is occupied by atoms. It's a key indicator of the efficiency of atomic packing. We will calculate this for BCC in detail below.
- Space Lattice: The space lattice for BCC is cubic.
Calculating the Atomic Packing Factor (APF) for BCC
The Atomic Packing Factor (APF) is a crucial metric that quantifies the efficiency of atomic packing in a crystal structure. It represents the percentage of the unit cell volume occupied by atoms, assuming atoms are hard spheres. A higher APF indicates a more densely packed structure.
For BCC, the calculation involves several steps:
-
Volume of Atoms: Assuming each atom is a sphere with radius 'r', the volume of a single atom is (4/3)πr³. Since there are two atoms per unit cell in BCC, the total volume of atoms in the unit cell is 2 x (4/3)πr³ = (8/3)πr³.
-
Relationship Between Atomic Radius and Unit Cell Length: In a BCC structure, the body diagonal of the cube connects two opposite corner atoms and passes through the central atom. The length of the body diagonal can be expressed in terms of the unit cell length 'a' and the atomic radius 'r' using the Pythagorean theorem in three dimensions:
a² + a² + a² = (4r)² => 3a² = 16r² => a = (4r) / √3
-
Volume of the Unit Cell: The volume of the cubic unit cell is simply a³. Substituting the expression for 'a' from step 2, we get:
V<sub>cell</sub> = a³ = [(4r) / √3]³ = (64r³) / (3√3)
-
Calculating APF: The Atomic Packing Factor is the ratio of the total volume of atoms in the unit cell to the volume of the unit cell:
APF = (Volume of atoms) / (Volume of unit cell) = [(8/3)πr³] / [(64r³) / (3√3)] = (π√3) / 8
-
Numerical Value: Substituting the value of π (approximately 3.1416), we obtain:
APF ≈ (3.1416 x 1.732) / 8 ≈ 0.6802
Therefore, the Atomic Packing Factor for a body-centered cubic structure is approximately 68.02%. This means that about 68% of the unit cell volume is occupied by atoms, leaving the remaining 32% as empty space.
Comparison with Other Crystal Structures
It's insightful to compare the packing efficiency of BCC with other common crystal structures:
- Simple Cubic (SC): The APF for a simple cubic structure is only 52.4%. This is the least efficient of the common crystal structures.
- Face-Centered Cubic (FCC): The FCC structure has a much higher APF of 74.05%, making it a more efficient packing arrangement than BCC.
- Hexagonal Close-Packed (HCP): Similar to FCC, HCP also has an APF of 74.05%, indicating equally efficient packing.
This comparison highlights that BCC, while not the most efficient packing arrangement, still provides a reasonably dense atomic structure.
Factors Affecting Packing Efficiency
While the theoretical APF for BCC is 68.02%, several factors can influence the actual packing efficiency in real-world materials:
- Atomic Size and Shape: The assumption of perfectly spherical atoms is an idealization. In reality, atoms may have slightly different sizes and shapes, affecting the packing.
- Temperature and Pressure: Variations in temperature and pressure can alter interatomic distances and consequently affect the packing efficiency.
- Presence of Defects: Crystal defects, such as vacancies, dislocations, and grain boundaries, disrupt the regular atomic arrangement and reduce the effective packing efficiency.
- Alloying: The addition of alloying elements can change the lattice parameters and the atomic arrangement, influencing the overall packing density.
Implications of BCC Packing Efficiency
The relatively high packing efficiency of the BCC structure contributes to several important properties of materials exhibiting this structure:
- Mechanical Strength: The dense packing of atoms in BCC contributes to its moderate to high strength, especially at lower temperatures. The high coordination number also leads to strong interatomic bonding.
- Ductility: BCC metals generally show a degree of ductility, which is influenced by factors like temperature and the presence of defects. The ability of the atoms to slide past each other is affected by the atomic arrangement and the interatomic forces.
- Density: The high packing efficiency results in relatively high density compared to materials with lower APF structures.
- Magnetic Properties: Several BCC metals exhibit notable magnetic properties, such as ferromagnetism (e.g., iron), influenced by the atomic arrangement and electron configuration.
Applications of BCC Metals
The unique properties stemming from the BCC structure make these materials useful in various applications:
- Steel: Iron, a BCC metal, is the primary component of steel, and its properties are significantly influenced by its BCC structure at lower temperatures.
- Tool Steels: Alloying additions to iron, in tool steels, further enhance the strength and hardness of the material.
- Refractory Metals: Metals like tungsten and molybdenum, which possess BCC structures at room temperature, find applications in high-temperature applications due to their high melting points and strength at elevated temperatures.
- Nuclear Reactors: Some BCC metals possess excellent neutron absorption capabilities and are employed in nuclear reactor components.
Conclusion
The body-centered cubic structure, with its characteristic packing efficiency of approximately 68.02%, plays a crucial role in determining the properties of a wide range of metals and alloys. Understanding the details of this structure, its packing efficiency, and its relationship to material properties is essential for materials science and engineering applications. While not the most densely packed structure, the balance of density, strength, and ductility makes BCC materials versatile and vital across diverse technological domains. Further research and development continue to explore and exploit the unique characteristics of BCC materials, leading to ongoing advancements in material science and engineering. Future studies may focus on manipulating the defects and alloying to fine-tune the properties further, maximizing the potential of BCC metals in various high-performance applications.
Latest Posts
Latest Posts
-
Formula Para Sacar Diametro De Un Circulo
Apr 01, 2025
-
How To Make A Frequency Histogram In Excel
Apr 01, 2025
-
What Is The Monomer Of A Dna Molecule
Apr 01, 2025
-
When Does The Segregation Of Alleles Occur
Apr 01, 2025
-
Cardiac Muscles Differ From Skeletal Muscles In That They
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Packing Efficiency Of Body Centered Cubic . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
