Ray Tracing For A Converging Lens
Muz Play
Apr 05, 2025 · 6 min read
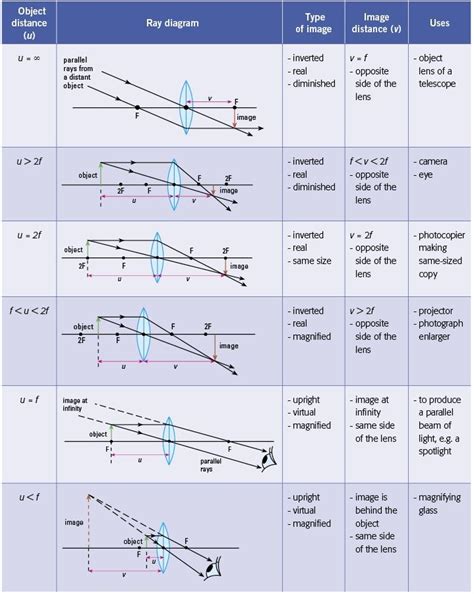
Table of Contents
Ray Tracing for a Converging Lens: A Comprehensive Guide
Ray tracing is a fundamental technique in geometrical optics used to predict the path of light rays as they interact with optical components. For converging lenses (also known as convex lenses), ray tracing allows us to determine the location and characteristics of the image formed. This guide provides a comprehensive understanding of ray tracing for converging lenses, covering various aspects from basic principles to advanced applications.
Understanding Converging Lenses
A converging lens is a lens that converges parallel rays of light to a single point called the focal point (F). This convergence is due to the lens's curved surface, which refracts (bends) the light rays. The distance between the lens and the focal point is called the focal length (f). The focal length is a crucial parameter determining the lens's focusing power. A shorter focal length indicates a stronger converging power. Converging lenses are thicker in the middle than at the edges.
Key Terms and Definitions:
- Principal Axis: The imaginary line passing through the center of the lens and perpendicular to its surface.
- Optical Center: The central point of the lens where a ray of light passes through undeviated.
- Focal Point (F): The point where parallel rays of light converge after passing through the lens.
- Focal Length (f): The distance between the lens and the focal point.
- Object Distance (u): The distance between the object and the lens.
- Image Distance (v): The distance between the image and the lens.
- Real Image: An image formed by the actual convergence of light rays. It can be projected onto a screen.
- Virtual Image: An image formed where the light rays appear to converge, but they don't actually meet. It cannot be projected onto a screen.
- Magnification (M): The ratio of the image height to the object height. It can be positive (upright image) or negative (inverted image).
The Three Principal Rays in Ray Tracing
To accurately trace the path of light rays through a converging lens, three principal rays are typically used:
1. The Parallel Ray:
This ray travels parallel to the principal axis before striking the lens. After refraction, it passes through the focal point on the opposite side of the lens.
2. The Focal Ray:
This ray passes through the focal point on the same side of the lens as the object. After refraction, it emerges from the lens parallel to the principal axis.
3. The Central Ray:
This ray passes through the optical center of the lens. It travels undeviated through the lens.
By constructing these three rays, the intersection of any two of them determines the location of the image. Using all three rays provides a consistency check and improves accuracy.
Ray Tracing for Different Object Positions
The position and characteristics of the image formed by a converging lens depend significantly on the object's position relative to the lens. Let's explore different scenarios:
1. Object at Infinity:
When the object is placed at infinity (u = ∞), the incoming rays are essentially parallel. After refraction, all rays converge at the focal point (F) on the opposite side of the lens. The image formed is real, inverted, point-sized, and located at the focal point.
2. Object Beyond 2f:
If the object is placed beyond twice the focal length (u > 2f), the image formed is real, inverted, and diminished. The image is located between f and 2f on the opposite side of the lens.
3. Object at 2f:
When the object is placed at twice the focal length (u = 2f), the image formed is real, inverted, and the same size as the object. The image is located at 2f on the opposite side of the lens.
4. Object Between f and 2f:
If the object is placed between the focal length and twice the focal length (f < u < 2f), the image formed is real, inverted, and magnified. The image is located beyond 2f on the opposite side of the lens.
5. Object at f:
When the object is placed at the focal point (u = f), the refracted rays emerge parallel to the principal axis and never converge. Therefore, no image is formed.
6. Object Inside f:
If the object is placed closer to the lens than the focal point (u < f), the image formed is virtual, upright, and magnified. The image is located on the same side of the lens as the object. This is how a magnifying glass works.
The Lens Equation and Magnification Formula
The relationship between object distance (u), image distance (v), and focal length (f) is described by the lens equation:
1/u + 1/v = 1/f
The magnification (M) is given by:
M = -v/u
Where:
- A positive magnification indicates an upright image.
- A negative magnification indicates an inverted image.
- A magnification greater than 1 indicates a magnified image.
- A magnification less than 1 indicates a diminished image.
Advanced Applications and Considerations
Ray tracing provides a foundational understanding of image formation in converging lenses. However, more advanced techniques and considerations are necessary for a complete analysis in practical scenarios:
1. Aberrations:
Real lenses exhibit imperfections known as aberrations, which distort the image. Common aberrations include spherical aberration (due to the lens's spherical shape), chromatic aberration (due to the lens's different refractive indices for different wavelengths of light), and coma (off-axis image distortion). These aberrations limit the quality of the image formed.
2. Multiple Lenses:
Many optical systems utilize multiple lenses to correct aberrations and achieve specific optical properties. Ray tracing becomes more complex in these systems, often requiring computer-aided design (CAD) software.
3. Thick Lenses:
The simple lens equation and magnification formula assume a thin lens approximation, where the lens thickness is negligible compared to the object and image distances. For thick lenses, more sophisticated calculations are required.
4. Non-Paraxial Rays:
The thin lens equations assume paraxial rays—rays that make small angles with the principal axis. For larger angles (non-paraxial rays), more accurate ray tracing methods are needed, often involving Snell's law directly and considering the lens's curvature and refractive index precisely.
5. Diffraction:
At very small scales, the wave nature of light becomes significant, and diffraction effects must be considered. These effects are not captured by geometrical ray tracing.
Conclusion
Ray tracing is an invaluable tool for understanding the behaviour of light as it passes through a converging lens. While the basic principles are relatively straightforward, understanding the nuances of image formation, considering lens aberrations, and dealing with more complex scenarios requires a deeper dive into geometrical and physical optics. Mastering ray tracing is essential for anyone working in optics, photography, or related fields. This comprehensive guide provides a solid foundation for further exploration of this critical concept. Remember to practice ray tracing with different object positions and lens configurations to solidify your understanding. The more you practice, the better you will become at predicting image characteristics quickly and efficiently.
Latest Posts
Latest Posts
-
Number Of Atoms In Face Centered Cubic
Apr 06, 2025
-
Two Reactions Between A Grignard Reagent And A Carbonyl Compound
Apr 06, 2025
-
The Hawthorne Studies Concluded That Worker Motivation
Apr 06, 2025
-
Formula For Kinetic Energy Of A Spring
Apr 06, 2025
-
What Are The Three Types Of Asexual Reproduction
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Ray Tracing For A Converging Lens . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
