Time Rate Of Change Of Momentum
Muz Play
Mar 28, 2025 · 6 min read
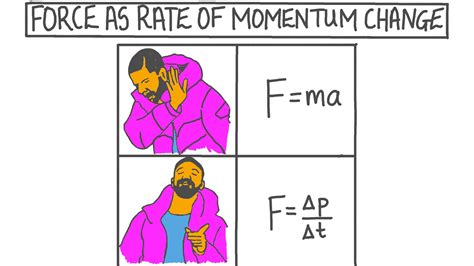
Table of Contents
Time Rate of Change of Momentum: Understanding Newton's Second Law
The concept of the time rate of change of momentum is fundamental to classical mechanics and forms the bedrock of Newton's second law of motion. Understanding this concept unlocks a deeper appreciation of how forces affect objects and their motion. This article will explore this crucial principle in detail, examining its implications, applications, and relevance across various physics scenarios.
What is Momentum?
Before delving into the time rate of change, let's establish a clear understanding of momentum itself. Momentum (p) is a vector quantity representing the mass in motion. It's defined as the product of an object's mass (m) and its velocity (v):
p = mv
The unit of momentum is typically kilogram-meters per second (kg⋅m/s). A heavier object moving at the same velocity as a lighter object will have a greater momentum. Similarly, an object moving at a higher velocity will have greater momentum than an object of the same mass moving at a lower velocity. This simple equation encapsulates a significant physical property.
Vector Nature of Momentum
The vector nature of momentum is crucial. It means momentum has both magnitude (size) and direction. If an object's velocity changes, either in magnitude or direction, its momentum also changes. This is paramount when considering changes in momentum over time.
The Time Rate of Change of Momentum
The time rate of change of momentum describes how rapidly the momentum of an object is altering over a specific time interval. It is calculated by finding the derivative of momentum with respect to time:
Δp/Δt = (p₂ - p₁)/(t₂ - t₁) where p₁ and p₂ represent momentum at times t₁ and t₂ respectively.
For infinitesimally small time intervals, this becomes:
dp/dt = lim (Δt→0) Δp/Δt
This represents the instantaneous time rate of change of momentum.
Newton's Second Law: The Force-Momentum Connection
Newton's second law of motion provides a powerful link between force and the time rate of change of momentum. It states that the net force (F<sub>net</sub>) acting on an object is directly proportional to the time rate of change of its momentum:
F<sub>net</sub> = dp/dt
This is a more fundamental and general statement of Newton's second law than the commonly known F = ma. While F = ma is valid for situations where mass remains constant, F<sub>net</sub> = dp/dt holds true even when the mass of the object is changing (e.g., a rocket expelling fuel).
Constant Mass Scenario
When the mass (m) of an object remains constant, we can derive the familiar equation F = ma from Newton's second law:
Since p = mv, then dp/dt = d(mv)/dt = m(dv/dt) (assuming constant mass)
As dv/dt is the acceleration (a), we arrive at:
F<sub>net</sub> = ma
This equation is widely used in many physics problems involving constant mass systems.
Variable Mass Scenario
The true power of F<sub>net</sub> = dp/dt lies in its ability to handle scenarios where mass is not constant. Consider a rocket launching into space. As it expels fuel, its mass decreases continuously. The force from the expelled fuel is crucial in accelerating the rocket. The equation F = ma doesn't directly account for this changing mass. However, F<sub>net</sub> = dp/dt accurately describes the system's dynamics by incorporating the changing momentum.
Examples of Variable Mass Systems
Several real-world scenarios exemplify the importance of considering the changing mass in momentum calculations:
- Rocket Propulsion: As mentioned, rockets continuously lose mass as they burn fuel. The thrust produced by the expelled gases changes the rocket's momentum, leading to acceleration.
- Chain Falling from a Table: Imagine a chain hanging over the edge of a table. As it falls, its mass increases below the edge, and the momentum increases proportionally.
- Rain Falling on a Moving Vehicle: The impact of raindrops on a moving vehicle leads to a change in the vehicle's momentum. This involves a variable mass system since each raindrop adds to the vehicle's total mass.
- Conveyor Belt Loading: Consider items being placed on a moving conveyor belt. The belt's momentum is affected as the mass it carries increases.
Applications of Time Rate of Change of Momentum
The time rate of change of momentum is pivotal in numerous physics applications:
Impulse and Momentum Change
Impulse (J) is defined as the change in momentum of an object:
J = Δp = p₂ - p₁ = ∫F dt
The impulse-momentum theorem states that the impulse acting on an object is equal to the change in its momentum. This is incredibly useful in collision problems, where the force might be unknown but the change in momentum is measurable.
Collisions
In collisions, whether elastic (kinetic energy is conserved) or inelastic (kinetic energy is not conserved), the time rate of change of momentum plays a crucial role in analyzing the outcome. The principle of conservation of momentum asserts that the total momentum of an isolated system remains constant if no external forces act on it. This is especially valuable in analyzing collisions, where the forces during impact can be difficult to determine directly.
Calculating Forces from Momentum Changes
Knowing the time rate of change of momentum enables us to calculate the net force acting on an object, even in complex situations where forces might be difficult to measure directly. This is particularly useful in situations with variable forces or short durations of interaction.
Advanced Considerations
Relativistic Momentum
In situations involving speeds approaching the speed of light, classical mechanics breaks down. Einstein's theory of special relativity introduces the concept of relativistic momentum, which modifies the classical formula to account for the effects of high speeds:
p = γmv, where γ = 1/√(1 - v²/c²) and c represents the speed of light.
At low speeds (v << c), relativistic momentum approximates classical momentum. However, at speeds approaching the speed of light, the relativistic correction becomes significant.
Angular Momentum
While this article primarily focuses on linear momentum, the concept of the time rate of change extends to angular momentum (L). Angular momentum is the rotational equivalent of linear momentum. The time rate of change of angular momentum is related to torque (τ), the rotational equivalent of force:
d L/dt = τ
This relationship is vital in understanding rotational motion and the effects of torques on rotating objects.
Conclusion
The time rate of change of momentum is a fundamental concept in classical and relativistic mechanics. Its importance extends beyond the simple F = ma equation, encompassing situations with variable mass and high speeds. By grasping the implications of Newton's second law in its complete form (F<sub>net</sub> = dp/dt), one can analyze complex dynamical systems and gain a deeper understanding of how forces interact with objects in motion. From rocket propulsion to collision analysis, this concept serves as a cornerstone of physics, enabling scientists and engineers to model and predict the behavior of various systems. The versatility and applicability of this concept solidify its place as a crucial component of the study of motion and dynamics. It's a principle that remains relevant across diverse fields, highlighting its enduring power and relevance in understanding the physical world.
Latest Posts
Latest Posts
-
5 Blind Man And The Elephant
Mar 31, 2025
-
Which Statement Describes The Citric Acid Cycle
Mar 31, 2025
-
Why Do Plants Love Water In Bio Terms
Mar 31, 2025
-
Identifying The Important Intermolecular Forces In Pure Compounds
Mar 31, 2025
-
Why Does Km Increase In Competitive Inhibition
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Time Rate Of Change Of Momentum . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
