Which Best Represents The Center Of The Data Set Below
Muz Play
Mar 30, 2025 · 6 min read
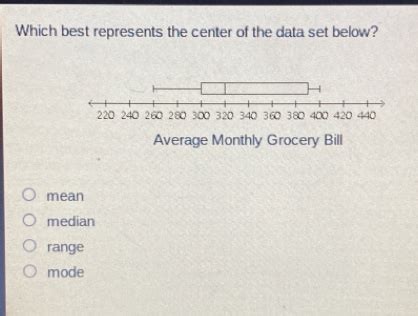
Table of Contents
Which Best Represents the Center of the Data Set? A Deep Dive into Measures of Central Tendency
Understanding the center of a dataset is fundamental in statistics. It allows us to summarize and interpret large amounts of data efficiently, providing a single value that represents the typical or average value within the dataset. However, there isn't one single "best" measure; the optimal choice depends heavily on the nature of the data and the research question. This article explores the most common measures of central tendency – mean, median, and mode – examining their strengths, weaknesses, and appropriate applications, ultimately guiding you to choose the most representative measure for your specific dataset.
The Mean: The Arithmetic Average
The mean, often referred to as the average, is the most commonly used measure of central tendency. It's calculated by summing all the values in a dataset and then dividing by the total number of values. The formula is straightforward:
Mean = Σx / n
where:
- Σx represents the sum of all values in the dataset.
- n represents the total number of values.
Strengths of the Mean:
- Simplicity and Familiarity: The mean is easy to understand and calculate, making it a widely accessible measure.
- Sensitivity to all data points: The mean considers every data point in the calculation, providing a comprehensive representation of the entire dataset.
- Mathematical Properties: The mean possesses useful mathematical properties that make it valuable in statistical analyses and further calculations. For instance, the sum of deviations from the mean always equals zero.
Weaknesses of the Mean:
- Susceptibility to Outliers: The mean is highly sensitive to outliers (extremely high or low values). A single outlier can significantly skew the mean, making it a poor representation of the central tendency when dealing with data containing extreme values. Consider a dataset representing the salaries of employees in a company. If the CEO's exceptionally high salary is included, the mean salary will be inflated and not accurately reflect the typical employee's salary.
- Not suitable for skewed data: In datasets with skewed distributions (where data is heavily concentrated on one side), the mean can be misleading as it's pulled towards the tail of the distribution.
- Not applicable to categorical data: The mean is only applicable to numerical data and cannot be used for categorical data (e.g., colors, types of cars).
The Median: The Middle Value
The median is the middle value in a dataset when the data is arranged in ascending order. If the dataset contains an even number of values, the median is the average of the two middle values.
Strengths of the Median:
- Robustness to Outliers: The median is resistant to outliers. Extreme values don't influence the median as significantly as they do the mean. This makes it a more reliable measure of central tendency for datasets containing outliers. Using the salary example again, the median salary would provide a more accurate representation of the typical employee's earnings, unaffected by the CEO's high salary.
- Suitable for skewed data: The median provides a better representation of the center for skewed data as it's less affected by the extreme values that skew the distribution.
- Applicable to ordinal data: Unlike the mean, the median can be used for ordinal data (data that has a natural order, such as rankings).
Weaknesses of the Median:
- Less sensitive to all data points: The median only considers the middle value(s) and ignores the magnitude of other data points. This can be a limitation if you need a measure that incorporates all the data's information.
- Less intuitive for some: While conceptually simple, understanding the median might be slightly less intuitive than understanding the mean for some individuals.
- Less useful for further statistical calculations: Compared to the mean, the median has fewer useful mathematical properties, making it less suitable for complex statistical analyses.
The Mode: The Most Frequent Value
The mode is the value that appears most frequently in a dataset. A dataset can have one mode (unimodal), two modes (bimodal), or multiple modes (multimodal). If all values appear with equal frequency, there's no mode.
Strengths of the Mode:
- Simple to understand and identify: The mode is the easiest measure of central tendency to understand and identify, especially in small datasets.
- Applicable to both numerical and categorical data: Unlike the mean and median, the mode can be used for both numerical and categorical data. For example, in a dataset of car colors, the mode would represent the most common car color.
- Useful for identifying clusters: The mode can highlight clusters or peaks in the data distribution, providing insights beyond a single central value.
Weaknesses of the Mode:
- Not always unique: A dataset can have multiple modes or no mode at all, making it less definitive compared to the mean or median.
- Sensitive to small changes in data: A slight change in the frequency of a value can change the mode significantly.
- Not suitable for all data types: The mode is less informative for continuous data, where values are unlikely to repeat exactly.
Choosing the Best Measure: A Practical Guide
Selecting the appropriate measure of central tendency depends on the specific characteristics of your data and the goals of your analysis. Here's a practical guide:
-
For symmetrical data with no outliers: The mean is generally the best choice, as it efficiently incorporates all data points.
-
For skewed data or data with outliers: The median is preferred as it's resistant to outliers and provides a more robust representation of the center.
-
For categorical data or to identify the most frequent value: The mode is the most suitable measure.
-
For a quick overview of a small dataset: The mode can be easily identified and provides immediate insights.
Example:
Let's consider two datasets:
Dataset A: 10, 12, 15, 15, 18, 20
- Mean: 15
- Median: 15
- Mode: 15
In this symmetrical dataset, all three measures are the same, providing a consistent representation of the center.
Dataset B: 10, 12, 15, 15, 18, 100
- Mean: 28.33
- Median: 15
- Mode: 15
Here, the outlier (100) significantly inflates the mean. The median and mode provide a more accurate representation of the typical value. The median is likely the best representation of the center in this case.
Beyond the Basics: Other Considerations
-
Weighted Mean: This is used when different data points have different weights or importance. For instance, calculating a weighted average grade where different assignments carry different weight percentages.
-
Geometric Mean: Used for data representing multiplicative relationships, such as growth rates or investment returns.
-
Harmonic Mean: Suitable for data representing rates or ratios, such as speeds or prices.
Choosing the best measure of central tendency involves careful consideration of the data's characteristics and the specific question you are trying to answer. By understanding the strengths and weaknesses of each measure, you can confidently select the most appropriate representation of the center for your dataset and draw accurate conclusions from your analysis. Remember, context is key, and a combination of measures may sometimes provide the most comprehensive understanding of your data.
Latest Posts
Latest Posts
-
Completa Estas Oraciones Con Las Preposiciones Por O Para
Apr 01, 2025
-
Cartilage Is Separated From Surrounding Tissues By A Fibrous
Apr 01, 2025
-
Archaea And Bacteria Are Most Similar In Terms Of Their
Apr 01, 2025
-
Elements Are Organized On The Periodic Table According To
Apr 01, 2025
-
The Envelope Of A Virus Is Derived From The Hosts
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Which Best Represents The Center Of The Data Set Below . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
