Calculate The Binding Energy Per Nucleon
Muz Play
Apr 02, 2025 · 5 min read
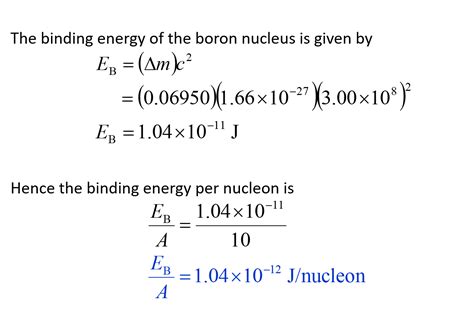
Table of Contents
Calculating the Binding Energy Per Nucleon: A Comprehensive Guide
Understanding the binding energy per nucleon is crucial for comprehending nuclear stability and the processes that govern nuclear reactions, from the power of the sun to the development of nuclear weapons. This comprehensive guide will walk you through the calculation, explaining the concepts involved and offering practical examples.
What is Binding Energy?
At the heart of nuclear physics lies the concept of binding energy. Atoms are made of protons and neutrons, collectively known as nucleons, held together in the nucleus by the strong nuclear force. This force is significantly stronger than the electromagnetic force repelling the positively charged protons, yet it operates only over extremely short distances. The energy required to completely disassemble a nucleus into its constituent protons and neutrons is its binding energy. This energy represents the difference between the mass of the nucleus and the sum of the masses of its individual protons and neutrons. This mass difference is a direct consequence of Einstein's famous equation, E=mc², where energy (E) and mass (m) are interchangeable, with 'c' representing the speed of light.
Mass Defect and Binding Energy
The difference between the mass of the nucleus and the sum of the masses of its individual nucleons is called the mass defect (Δm). This mass defect is converted into binding energy according to Einstein's equation:
Binding Energy (E) = Δm * c²
Where:
- Δm is the mass defect (in kilograms)
- c is the speed of light (approximately 3 x 10⁸ m/s)
- E is the binding energy (in Joules)
Calculating Binding Energy Per Nucleon
While the binding energy provides valuable insight into the stability of a nucleus, a more useful quantity for comparing the stability of different nuclei is the binding energy per nucleon. This value represents the average binding energy per nucleon in the nucleus. It's calculated by dividing the total binding energy by the total number of nucleons (protons + neutrons).
Binding Energy Per Nucleon = Total Binding Energy / Number of Nucleons
This value is expressed in mega-electronvolts (MeV) per nucleon. A higher binding energy per nucleon indicates a more stable nucleus. Nuclei with the highest binding energy per nucleon are the most stable, like iron-56.
Step-by-Step Calculation: An Example with Helium-4
Let's illustrate the calculation with Helium-4 (⁴He), which has 2 protons and 2 neutrons.
1. Determine the masses:
- Mass of a proton (mp) ≈ 1.007276 u (atomic mass units)
- Mass of a neutron (mn) ≈ 1.008665 u
- Mass of Helium-4 nucleus (mHe) ≈ 4.001506 u
2. Calculate the expected mass:
The expected mass of the Helium-4 nucleus if the nucleons were simply added together would be:
Expected Mass = (2 * mp) + (2 * mn) = (2 * 1.007276 u) + (2 * 1.008665 u) = 4.031882 u
3. Calculate the mass defect (Δm):
Mass defect (Δm) = Expected Mass - Actual Mass = 4.031882 u - 4.001506 u = 0.030376 u
4. Convert the mass defect to kilograms:
1 u ≈ 1.66054 x 10⁻²⁷ kg
Δm = 0.030376 u * (1.66054 x 10⁻²⁷ kg/u) ≈ 5.044 x 10⁻²⁹ kg
5. Calculate the binding energy (E):
Using Einstein's equation (E = Δm * c²):
E = (5.044 x 10⁻²⁹ kg) * (3 x 10⁸ m/s)² ≈ 4.54 x 10⁻¹² Joules
6. Convert binding energy to MeV:
1 Joule ≈ 6.242 x 10¹² MeV
E ≈ 4.54 x 10⁻¹² Joules * (6.242 x 10¹² MeV/Joule) ≈ 28.3 MeV
7. Calculate the binding energy per nucleon:
Binding Energy per Nucleon = Total Binding Energy / Number of Nucleons = 28.3 MeV / 4 nucleons ≈ 7.07 MeV/nucleon
Therefore, the binding energy per nucleon for Helium-4 is approximately 7.07 MeV/nucleon.
The Binding Energy Curve and Nuclear Stability
Plotting the binding energy per nucleon against the mass number (A = number of protons + neutrons) yields the binding energy curve. This curve reveals important insights into nuclear stability:
- Peak Stability: The curve peaks around iron-56 (⁵⁶Fe), indicating that iron-56 has the highest binding energy per nucleon and is therefore exceptionally stable.
- Fission and Fusion: Nuclei with mass numbers lighter than iron-56 can release energy through nuclear fusion (combining lighter nuclei to form heavier ones), while nuclei heavier than iron-56 can release energy through nuclear fission (splitting heavier nuclei into lighter ones). This is why fusion powers the sun and fission is utilized in nuclear power plants.
- Nuclear Stability and Isotopes: The curve also explains why certain isotopes are more stable than others. Isotopes with a higher binding energy per nucleon are more stable.
Factors Affecting Binding Energy
Several factors contribute to the binding energy of a nucleus:
- Strong Nuclear Force: The primary force responsible for binding nucleons together. Its short range and strong nature explain the high binding energies observed.
- Coulomb Repulsion: The electrostatic repulsion between protons destabilizes the nucleus. This effect becomes increasingly significant as the number of protons increases.
- Neutron-Proton Ratio: The optimal ratio of neutrons to protons varies depending on the nucleus size. A balanced ratio generally leads to greater stability. Light nuclei tend to have a neutron-to-proton ratio close to 1:1, while heavier nuclei require a higher neutron-to-proton ratio for stability due to the increased Coulomb repulsion between protons.
- Pairing Effect: Nuclei with even numbers of protons and neutrons tend to be more stable than those with odd numbers. This pairing effect arises from the quantum mechanical nature of nucleons.
- Shell Model: The nuclear shell model, similar to the electronic shell model in atoms, explains the stability of certain "magic numbers" of nucleons (2, 8, 20, 28, 50, 82, 126). Nuclei with these magic numbers exhibit enhanced stability.
Applications of Binding Energy Calculations
Understanding binding energy per nucleon has numerous applications in various fields:
- Nuclear Physics: Essential for understanding nuclear reactions, nuclear stability, and the properties of different isotopes.
- Nuclear Energy: Crucial for designing nuclear reactors and predicting energy release in fission and fusion processes.
- Astrophysics: Explains the energy generation processes in stars, including nuclear fusion reactions.
- Medical Physics: Used in medical imaging techniques and radiation therapy.
Conclusion
Calculating the binding energy per nucleon is a fundamental concept in nuclear physics with far-reaching implications. This guide provides a thorough understanding of the calculation process, the factors influencing binding energy, and its significance in various fields. By mastering this concept, you gain a deeper appreciation for the complexities of the atomic nucleus and the forces governing its behavior. Remember that precise calculations often require more advanced techniques and access to nuclear mass data tables, but this guide provides a solid foundation for understanding the underlying principles.
Latest Posts
Latest Posts
-
What Is A Wet Mount Slide
Apr 03, 2025
-
Examples Of Stoichiometry In Real Life
Apr 03, 2025
-
Why Is Water Necessary For Biological Organisms
Apr 03, 2025
-
How Is Probability Used In Genetics
Apr 03, 2025
-
Liquid To Gas Endothermic Or Exothermic
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Calculate The Binding Energy Per Nucleon . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
