Central Limit Theorem With Means Calculator
Muz Play
Apr 01, 2025 · 7 min read
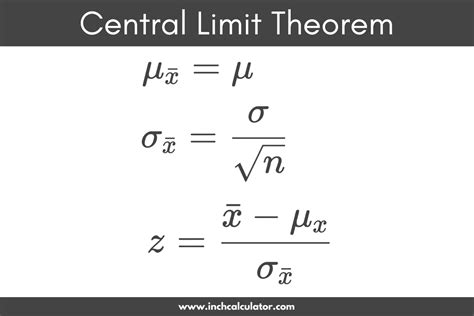
Table of Contents
Central Limit Theorem with Means Calculator: A Deep Dive
The Central Limit Theorem (CLT) is a cornerstone of statistics, providing a powerful bridge between theoretical probability distributions and real-world data analysis. It essentially states that the distribution of sample means approximates a normal distribution, regardless of the shape of the original population distribution, as the sample size increases. This remarkable property allows us to make inferences about population parameters even when we lack complete knowledge of the population distribution. This article will explore the CLT in detail, focusing on its practical applications and the utility of means calculators in understanding and leveraging its power.
Understanding the Central Limit Theorem
At its core, the CLT asserts that if you draw numerous random samples of a sufficiently large size from any population, the distribution of the sample means will resemble a normal distribution. This is true even if the original population isn't normally distributed. The larger the sample size, the closer the distribution of sample means will be to a perfect normal curve.
Key Aspects of the CLT:
-
Sample Size: The theorem's power hinges on the sample size (n). Generally, a sample size of 30 or more is considered sufficient for the CLT to hold reasonably well, although the required size might depend on the shape of the underlying population distribution. Highly skewed populations may require larger sample sizes.
-
Sample Means: The CLT focuses on the distribution of the means calculated from these random samples, not the individual data points themselves.
-
Approximation: It's crucial to remember that the CLT provides an approximation to a normal distribution. The larger the sample size, the more accurate this approximation becomes.
-
Independence: The samples must be independent. This means that the selection of one sample point shouldn't influence the selection of another.
-
Finite Variance: The population from which the samples are drawn must have a finite variance.
The Significance of the CLT
The implications of the CLT are far-reaching, underpinning many statistical procedures and analyses. Its significance lies in:
-
Hypothesis Testing: Many statistical tests rely on the assumption of normality. The CLT justifies this assumption even when the underlying population isn't normal, allowing us to use powerful techniques like t-tests and z-tests on data that might not strictly meet the normality criterion.
-
Confidence Intervals: Constructing confidence intervals for population means relies heavily on the CLT. These intervals provide a range within which the true population mean is likely to fall, with a specified level of confidence. The CLT ensures that the sampling distribution of the mean is approximately normal, allowing us to calculate these intervals accurately.
-
Statistical Inference: More broadly, the CLT is fundamental to the field of statistical inference, allowing us to draw conclusions about a population based on a sample. This is crucial in numerous fields, including medicine, engineering, social sciences, and finance.
Means Calculator: A Practical Tool
Understanding the CLT conceptually is crucial, but its application often involves complex calculations. A means calculator simplifies this process, automating many of the steps involved in determining the properties of the sampling distribution of the mean.
Functionality of a Means Calculator:
A well-designed means calculator should allow you to input the following information:
- Population Mean (μ): The average of the entire population.
- Population Standard Deviation (σ): A measure of the spread or dispersion of the population data.
- Sample Size (n): The number of data points in each sample.
Based on this input, a means calculator can calculate:
- Mean of the Sampling Distribution (μ<sub>x̄</sub>): This is equal to the population mean (μ).
- Standard Error of the Mean (SEM): This is the standard deviation of the sampling distribution and is calculated as σ/√n. The SEM measures the variability of sample means around the population mean.
- Probability Calculations: Many calculators allow you to calculate probabilities associated with specific ranges of sample means, utilizing the normal distribution's properties. For instance, you can determine the probability of obtaining a sample mean within a particular interval.
Illustrative Example: Using a Means Calculator
Let's consider a scenario where we are interested in the average height of adult women in a certain city. Suppose we know that the population mean height (μ) is 165 cm and the population standard deviation (σ) is 5 cm. We collect samples of 36 women each.
Using a means calculator, we would input:
- μ = 165 cm
- σ = 5 cm
- n = 36
The calculator would then provide:
- μ<sub>x̄</sub> = 165 cm (This is always equal to the population mean)
- SEM = σ/√n = 5/√36 = 0.83 cm
This indicates that the average of the sample means will be 165 cm, and the standard deviation of these sample means (the standard error) is 0.83 cm. We can now use this information to estimate probabilities related to sample means from our data. For instance, we could calculate the probability that a randomly selected sample would have a mean height between 164 cm and 166 cm.
Beyond Basic Calculations: Advanced Applications
While basic means calculators handle the core calculations of the CLT, more advanced tools can offer additional features:
-
Visualization: Some calculators offer graphical representations of the sampling distribution, allowing you to visualize how the distribution of sample means approaches normality as the sample size increases.
-
Different Population Distributions: Advanced calculators might handle scenarios where the underlying population distribution isn't specified, using simulation techniques to estimate the sampling distribution.
-
Confidence Interval Calculation: Some tools directly calculate confidence intervals based on the input parameters and the chosen confidence level.
Choosing the Right Means Calculator
Selecting the appropriate means calculator depends on your needs and technical expertise. Consider these factors:
- Functionality: Does the calculator offer all the features you require (probability calculations, visualization, etc.)?
- User-Friendliness: Is the interface intuitive and easy to use?
- Accuracy: Is the calculator known for its reliable calculations?
- Availability: Is the calculator freely accessible online or requires a paid subscription?
Limitations of the CLT
While incredibly powerful, the CLT has some limitations:
-
Sample Size: While a sample size of 30 is often sufficient, extremely skewed or heavy-tailed distributions might require much larger samples to achieve a reasonable approximation to normality.
-
Independence: The assumption of independence between samples is crucial. Violation of this assumption can lead to inaccurate results.
-
Finite Variance: The CLT assumes the population has a finite variance. Distributions with infinite variance (e.g., Cauchy distribution) don't satisfy this condition, and the CLT doesn't apply.
-
Approximation: It's crucial to remember that the CLT provides an approximation. The approximation improves with larger sample sizes, but it's never perfect, especially for small sample sizes or non-normal populations.
Practical Applications Across Disciplines
The CLT's reach extends far beyond theoretical statistics. Here are some examples of its use in different fields:
-
Quality Control: In manufacturing, the CLT is used to monitor product quality by analyzing the distribution of sample measurements.
-
Medical Research: Clinical trials rely on the CLT to analyze the effects of treatments by comparing the means of treatment and control groups.
-
Finance: Financial models often assume that asset returns are normally distributed (or at least approximately so), which is often justified by the CLT.
-
Environmental Science: The CLT is used to analyze environmental data, such as pollution levels or temperature measurements.
Conclusion
The Central Limit Theorem is a fundamental concept in statistics, providing a powerful tool for making inferences about populations based on sample data. Understanding the CLT, coupled with the use of means calculators, empowers researchers and analysts across numerous disciplines to conduct effective data analysis and draw meaningful conclusions. While the CLT has its limitations, its ability to simplify complex statistical problems makes it an indispensable tool in the realm of data science. Remember to always consider the assumptions of the CLT and the limitations of any means calculator you choose to use. A solid grasp of both the theoretical foundation and the practical application of this theorem is essential for anyone working with statistical data.
Latest Posts
Latest Posts
-
Is Flammability A Chemical Or Physical Property
Apr 02, 2025
-
Skewed Left Stem And Leaf Plot
Apr 02, 2025
-
Molecules Consisting Only Of Carbon And Hydrogen Are Called
Apr 02, 2025
-
How To Read A Solubility Curve
Apr 02, 2025
-
Difference Between Fractional And Simple Distillation
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Central Limit Theorem With Means Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
