Do Trailing Zeros Count As Sig Figs
Muz Play
Mar 30, 2025 · 5 min read
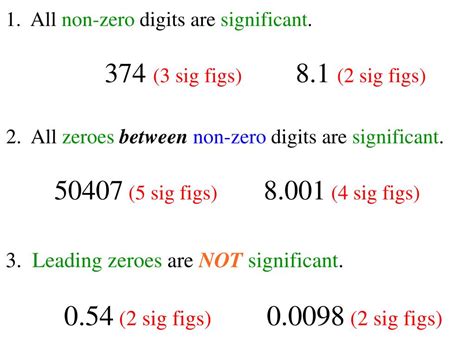
Table of Contents
Do Trailing Zeros Count as Significant Figures? A Comprehensive Guide
Determining the number of significant figures (sig figs) in a measurement is crucial for accurate scientific calculations and reporting. While the rules for most significant figures are relatively straightforward, trailing zeros—zeros that appear at the end of a number—present a unique challenge. This comprehensive guide will clarify when trailing zeros count as significant figures and when they don't, equipping you with the knowledge to handle them confidently in any scientific context.
Understanding Significant Figures
Before delving into the intricacies of trailing zeros, let's establish a solid foundation in understanding significant figures. Significant figures represent the digits in a number that carry meaning contributing to its precision. They reflect the uncertainty inherent in any measurement. A higher number of significant figures indicates greater precision.
Key Rules for Identifying Significant Figures:
- Non-zero digits are always significant. For example, in the number 123, all three digits are significant.
- Zeros between non-zero digits are always significant. In 102, the zero is significant.
- Leading zeros (zeros to the left of the first non-zero digit) are never significant. They only serve to indicate the magnitude of the number. For example, in 0.0012, only 1 and 2 are significant.
- Trailing zeros (zeros to the right of the last non-zero digit) can be significant or insignificant, depending on the context. This is where the complexities lie, and the focus of this article.
Trailing Zeros: The Ambiguity
The significance of trailing zeros hinges entirely on whether a decimal point is present. This is because the presence or absence of a decimal point strongly suggests the level of precision in the measurement.
Case 1: Trailing Zeros in Numbers Without a Decimal Point
Consider the number 100. Are all three zeros significant? The answer is no, unless explicitly stated otherwise. Without a decimal point, the trailing zeros might simply reflect the limitations of the measuring instrument rather than precise measurements. The number 100 could represent a measurement anywhere between 95 and 105. Therefore, in this case, it's often assumed that only one significant figure is present.
However, if the number 100 is written with an overbar on the last zero (100), this indicates that the zero is significant. Similarly, scientific notation clarifies ambiguity. Writing the number as 1.00 x 10² explicitly states that there are three significant figures. The use of scientific notation is strongly encouraged whenever there is any potential ambiguity in the number of significant figures.
Example: A measurement of 2500 meters, without further context, typically has only two significant figures (2 and 5). However, if the measurement is 2500. meters, the decimal point implies greater precision, indicating four significant figures.
Case 2: Trailing Zeros in Numbers With a Decimal Point
When a decimal point is present, trailing zeros become significant. This is because including the decimal point signals a deliberate effort to indicate precision. The inclusion of those zeros is intentional, indicating that the measurement has been made with a certain level of accuracy.
Example: The number 2500.0 has five significant figures. The trailing zeros after the decimal point are not placeholders; they are significant digits. The precision implied here is much higher than in the previous example of 2500 meters. Similarly, 1.000 has four significant figures.
Consider the difference:
- 2500 (Likely 2 significant figures)
-
- (Four significant figures)
- 2.500 x 10³ (Four significant figures – Scientific notation is clear)
The Role of Measurement Uncertainty
The inherent uncertainty in any measurement plays a critical role in determining the significance of trailing zeros. A measurement of 100g obtained using a scale with a resolution of 10g would only be precise to one significant figure. However, if obtained using a scale with a resolution of 1g, the uncertainty is much smaller, and it would have three significant figures.
Always consider the instrument's precision when deciding how many significant figures are appropriate in your measurements. This aspect is often neglected but significantly impacts the accuracy of scientific calculations and results.
Practical Applications and Examples
Let's solidify our understanding with more examples illustrating different scenarios:
Example 1: A student measures the length of a table to be 1.50 meters. This measurement has three significant figures. The trailing zero after the decimal point indicates a precision to the nearest centimeter.
Example 2: A chemist records the mass of a sample as 20.0 grams. This measurement has three significant figures. The trailing zero after the decimal point demonstrates a precision of 0.1 grams.
Example 3: The population of a city is reported as 1,200,000. The number of significant figures is ambiguous without additional context, likely between two and three. However, if reported as 1.20 x 10⁶, it explicitly shows three significant figures.
Example 4: A speed is recorded as 25.00 km/h. This measurement possesses four significant figures, demonstrating a level of precision to the nearest hundredth of a kilometer per hour.
Example 5: 0.00050 has two significant figures. The leading zeros are not significant, while the trailing zero after the decimal is significant.
Avoiding Ambiguity: Best Practices
To avoid confusion and ensure accurate communication of results, scientists often adhere to the following best practices:
-
Use Scientific Notation: Scientific notation removes any ambiguity associated with trailing zeros. For instance, expressing 1200 as 1.2 x 10³ clarifies that only two significant figures are present.
-
Specify Uncertainty: Explicitly state the uncertainty associated with a measurement. For example, "100 ± 5 meters" indicates that the measurement has only one significant figure.
-
Context is Key: Always consider the context of the measurement. The precision of the measuring instrument dictates the number of significant figures.
-
Round Carefully: When performing calculations, pay close attention to rounding rules to maintain the appropriate number of significant figures.
Conclusion
The treatment of trailing zeros in determining significant figures requires careful attention to detail. The presence or absence of a decimal point, along with the implied or explicitly stated uncertainty, significantly influences whether these zeros are considered significant. By consistently applying the rules and best practices outlined in this guide, you can ensure accuracy and clarity in scientific measurements and calculations, promoting better understanding and collaboration within the scientific community. Understanding these nuances is crucial for accurate scientific communication and reliable results. Remember to always consider the context of your measurement and prioritize clear communication through proper notation or explicit specification of uncertainty to avoid ambiguity.
Latest Posts
Latest Posts
-
Express The Inequality Using Interval Notation
Apr 01, 2025
-
Difference Between Hypothesis Law And Theory
Apr 01, 2025
-
Is An Atom The Smallest Particle
Apr 01, 2025
-
Central Limit Theorem With Means Calculator
Apr 01, 2025
-
What Is The Difference Between Lactic Acid And Alcoholic Fermentation
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Do Trailing Zeros Count As Sig Figs . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
