Equilibrium Solution Of A Differential Equation
Muz Play
Apr 01, 2025 · 6 min read
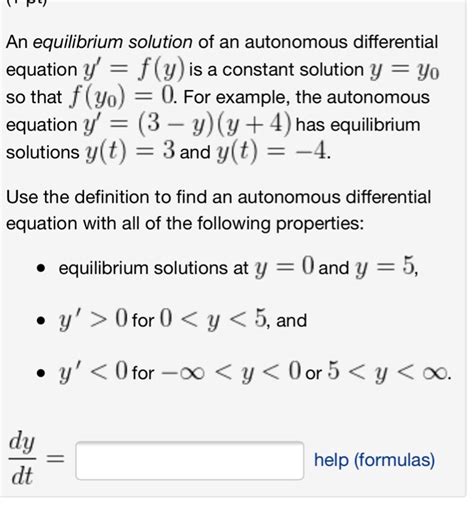
Table of Contents
Equilibrium Solutions of Differential Equations: A Comprehensive Guide
Understanding equilibrium solutions, also known as stationary points or critical points, is fundamental to analyzing differential equations. These solutions represent states where the system remains unchanged over time, offering crucial insights into the long-term behavior of dynamic systems. This comprehensive guide will delve into the theory and application of equilibrium solutions, covering various aspects from basic concepts to advanced techniques.
What are Equilibrium Solutions?
An equilibrium solution of a differential equation is a constant solution that satisfies the equation. In simpler terms, it's a value of the dependent variable that, once reached, will remain unchanged as the independent variable (often representing time) progresses. For a first-order differential equation of the form dy/dt = f(y), an equilibrium solution, y<sub>e</sub>, satisfies the condition f(y<sub>e</sub>) = 0. This means the rate of change is zero at the equilibrium point; the system is at rest.
Consider the example: dy/dt = y(1-y). Here, the equilibrium solutions are found by setting dy/dt = 0, leading to y(1-y) = 0. This gives us two equilibrium solutions: y = 0 and y = 1.
Finding Equilibrium Solutions
The process of finding equilibrium solutions depends on the type of differential equation.
First-Order Differential Equations
For a first-order ordinary differential equation (ODE) of the form dy/dt = f(t, y), equilibrium solutions are found by setting dy/dt = 0 and solving the resulting equation f(t, y) = 0 for y. If the equation is autonomous (meaning f(t, y) does not explicitly depend on t), then the equilibrium solutions are constant and independent of time.
Example: Let's consider the logistic growth model: dy/dt = ry(1 - y/K), where r and K are positive constants. Setting dy/dt = 0, we get ry(1 - y/K) = 0. This gives us two equilibrium solutions: y = 0 and y = K.
Higher-Order Differential Equations
Finding equilibrium solutions for higher-order ODEs is more involved. We need to convert the higher-order equation into a system of first-order equations. This is typically done by introducing new variables representing the derivatives of the original dependent variable. Then, we solve the system of equations simultaneously to find equilibrium points.
Example: Consider the second-order equation d²y/dt² + y = 0. We can rewrite this as a system of first-order equations: dy/dt = z and dz/dt = -y. Setting dy/dt = 0 and dz/dt = 0, we obtain z = 0 and y = 0. Therefore, the only equilibrium solution is y = 0, z = 0.
Systems of Differential Equations
For systems of differential equations, the equilibrium points are found by setting all derivatives equal to zero and solving the resulting system of algebraic equations. This can involve techniques like substitution, elimination, or numerical methods for complex systems.
Stability Analysis of Equilibrium Solutions
Equilibrium solutions aren't all created equal. Their stability dictates how the system behaves near the equilibrium point. There are three main types of stability:
1. Stable Equilibrium
A stable equilibrium solution is one where solutions starting near the equilibrium point converge towards it as time progresses. Small perturbations from the equilibrium will eventually decay, bringing the system back to its stable state. Think of a ball resting at the bottom of a bowl – if you nudge it slightly, it will roll back to the bottom.
2. Unstable Equilibrium
An unstable equilibrium solution is one where solutions starting near the equilibrium point diverge from it as time progresses. Even a tiny perturbation will cause the system to move away from the equilibrium. Imagine a ball balanced on top of a hill – any small disturbance will cause it to roll down.
3. Semi-stable Equilibrium
A semi-stable equilibrium exhibits a mix of stable and unstable behavior. Solutions approaching the equilibrium from one direction will converge towards it, while solutions approaching from another direction will diverge.
Methods for Determining Stability
Several methods can be used to determine the stability of equilibrium solutions:
1. Linearization
For systems described by differentiable equations, linearization around an equilibrium point provides a powerful tool for local stability analysis. This involves approximating the system's behavior near the equilibrium using a linear system obtained from the Jacobian matrix of the system's equations. The eigenvalues of this Jacobian matrix dictate the stability:
- All eigenvalues have negative real parts: The equilibrium is asymptotically stable (stable).
- At least one eigenvalue has a positive real part: The equilibrium is unstable.
- All eigenvalues have non-positive real parts, with at least one eigenvalue having a zero real part: Further analysis is needed (possibly using higher-order terms).
2. Phase Plane Analysis (for 2D systems)
For two-dimensional systems, phase plane analysis provides a graphical method for determining stability. By plotting the vector field of the system, we can visualize the trajectories of solutions and infer the stability of equilibrium points by observing the behavior of trajectories near those points.
3. Lyapunov Functions
Lyapunov functions provide a powerful and general approach to stability analysis. A Lyapunov function is a scalar function that decreases along the trajectories of the system, indicating stability. Finding an appropriate Lyapunov function can be challenging but is often a powerful tool when other methods are insufficient.
Applications of Equilibrium Solutions
Equilibrium solutions find wide-ranging applications across numerous scientific disciplines:
1. Population Dynamics
In population models, equilibrium solutions represent carrying capacities or extinction states. Stability analysis determines whether a population will stabilize at a certain size or go extinct.
2. Chemical Kinetics
Equilibrium solutions in chemical kinetics correspond to chemical equilibrium states where the rates of forward and reverse reactions are equal. Stability analysis indicates whether the equilibrium is stable or will shift due to external factors.
3. Mechanics
In mechanical systems, equilibrium solutions correspond to stable or unstable rest positions. Understanding their stability is crucial for designing stable structures and systems.
4. Economics
In economic models, equilibrium solutions represent market equilibrium points where supply equals demand. Stability analysis helps understand the response of the market to changes in supply or demand.
Advanced Concepts
The study of equilibrium solutions extends to more advanced concepts:
Bifurcation Theory
Bifurcation theory explores how changes in system parameters can lead to qualitative changes in the system's behavior, including the appearance or disappearance of equilibrium solutions, or changes in their stability.
Limit Cycles and Attractors
In nonlinear systems, limit cycles represent stable periodic orbits, acting as attractors for nearby trajectories. While not equilibrium solutions themselves, their existence significantly impacts the system's long-term behavior.
Numerical Methods
For complex systems lacking analytical solutions, numerical methods are employed to approximate equilibrium solutions and analyze their stability. These techniques involve iterative methods to find the roots of the system of equations defining the equilibrium points.
Conclusion
Equilibrium solutions are cornerstone concepts in the analysis of differential equations. Their identification and stability analysis provide profound insights into the long-term behavior of dynamic systems across diverse scientific and engineering fields. Understanding these concepts, along with the various methods for their analysis, is crucial for comprehending the dynamics of complex systems and making predictions about their future states. This comprehensive overview aims to equip readers with the foundational knowledge needed to effectively tackle problems involving equilibrium solutions in differential equations. Remember to always consider the specific context of your problem when analyzing equilibrium solutions and selecting appropriate methods for stability analysis.
Latest Posts
Latest Posts
-
Is Magnesium A Metal Nonmetal Or Metalloid
Apr 02, 2025
-
Number Of Atoms In A Simple Cubic Unit Cell
Apr 02, 2025
-
Inscribed Circle In A Right Triangle
Apr 02, 2025
-
Bacteria And Archaea Are Both Domains Consisting Of Prokaryotic Organisms
Apr 02, 2025
-
What Organelles Do Plants Have That Animals Dont
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Equilibrium Solution Of A Differential Equation . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
