Examples Of Instantaneous Rate Of Change
Muz Play
Apr 01, 2025 · 6 min read
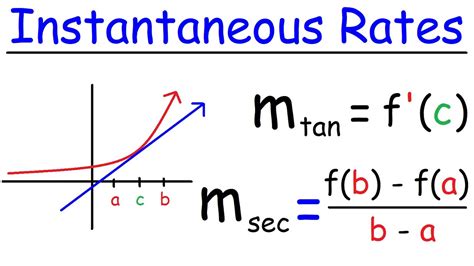
Table of Contents
Examples of Instantaneous Rate of Change: Understanding the Concept in Real-World Applications
The instantaneous rate of change represents a fundamental concept in calculus with far-reaching applications across numerous fields. Unlike the average rate of change, which considers the change over a period, the instantaneous rate of change focuses on the rate at a specific instant. This seemingly subtle difference unlocks the ability to analyze dynamic systems with incredible precision. This article will delve into a comprehensive exploration of the instantaneous rate of change, providing numerous real-world examples to solidify understanding.
Understanding the Concept
Before exploring examples, it's crucial to grasp the core idea. The instantaneous rate of change is essentially the slope of the tangent line to a curve at a particular point. Imagine a car's speed. The average speed over a journey is easily calculated (total distance / total time). However, the speed at any given moment – the instantaneous speed – requires a different approach. This is where calculus comes into play.
The instantaneous rate of change is formally defined using the concept of a derivative. The derivative of a function at a point represents the instantaneous rate of change of that function at that specific point. While the formal mathematical definition involves limits, intuitively, it's the slope of the tangent line, as mentioned earlier.
Real-World Examples of Instantaneous Rate of Change
Let's explore a range of examples where understanding instantaneous rate of change is crucial:
1. Speed and Velocity
This is perhaps the most intuitive example. The speedometer in your car displays the instantaneous speed. It doesn't calculate your average speed over the entire journey; instead, it measures your speed at that precise moment. Similarly, in physics, velocity is a vector quantity (including direction), and its instantaneous value provides crucial information about the motion of an object.
Example: A car accelerates from rest. Its speed isn't constant; it changes continuously. At any specific time (say, t=3 seconds), the speedometer gives the instantaneous speed. This speed is the instantaneous rate of change of the car's position with respect to time at t=3 seconds.
2. Population Growth
Modeling population growth often involves functions that describe the population size over time. The instantaneous rate of change of the population at a given time represents the population growth rate at that specific moment. This is vital for predicting future population sizes, understanding resource allocation, and making informed policy decisions.
Example: Consider a bacterial colony. Its population grows exponentially. The instantaneous rate of change at a particular time allows scientists to estimate how rapidly the colony is growing at that instant. This information is critical for understanding bacterial growth dynamics and developing effective control strategies.
3. Temperature Change
The rate at which temperature changes over time is another excellent illustration. The instantaneous rate of change of temperature indicates how rapidly the temperature is increasing or decreasing at a specific instant.
Example: Imagine a cup of hot coffee cooling down. The temperature doesn't drop uniformly. The instantaneous rate of change at any specific moment tells us how quickly the coffee is cooling at that precise instant, influenced by factors like room temperature and the coffee's initial temperature. Understanding this instantaneous rate allows for better prediction of the coffee's temperature at any given time.
4. Economic Growth
In economics, the instantaneous rate of change of economic indicators like Gross Domestic Product (GDP) helps economists understand the health of the economy. The instantaneous rate of change reflects the economy's growth rate at a specific point in time, rather than an average over a longer period.
Example: A country's GDP fluctuates over time. Economists use calculus to analyze the instantaneous rate of change of GDP to understand if the economy is accelerating, decelerating, or stagnating at any given moment. This information is crucial for making informed economic policy decisions.
5. Chemical Reactions
In chemistry, the rate of a chemical reaction often changes over time as reactants are consumed and products are formed. The instantaneous rate of change at a specific moment provides insight into the reaction's speed at that precise time.
Example: Consider the decomposition of a substance. The rate at which the substance breaks down changes as the reaction progresses. The instantaneous rate of change at any time 't' helps chemists model the reaction kinetics accurately and understand the reaction's mechanism.
6. Spread of Disease
Epidemiologists use models to predict the spread of infectious diseases. The instantaneous rate of change of the number of infected individuals at a given time represents the infection rate at that specific point.
Example: During a flu outbreak, the number of newly infected individuals changes over time. The instantaneous rate of change at different points in the outbreak helps epidemiologists understand the disease's transmission rate, predict future case numbers, and implement effective control measures.
7. Water Flow
The instantaneous rate of change of water flow in a river or pipe can be crucial for various applications. This is especially important for flood control and managing water resources.
Example: Imagine a dam releasing water. The rate of water flow isn't constant. Understanding the instantaneous rate of change at different times helps engineers manage water levels effectively, prevent flooding, and optimize water distribution.
8. Motion of a Projectile
When analyzing the trajectory of a projectile (like a ball thrown into the air), the instantaneous rate of change of its vertical velocity is the acceleration due to gravity. This constant acceleration influences the projectile's path.
Example: A ball thrown upwards experiences a constant downward acceleration due to gravity. The instantaneous rate of change of its vertical velocity at any point in its trajectory is this constant acceleration. This information is crucial for calculating the ball's maximum height and time of flight.
9. Current in an Electrical Circuit
In electrical circuits, the instantaneous rate of change of voltage across a capacitor is related to the current flowing through it.
Example: In a simple RC circuit (resistor-capacitor circuit), the instantaneous rate of change of the voltage across the capacitor determines the current flowing through the circuit. Understanding this relationship is vital for designing and analyzing electronic circuits.
10. Cardiac Output
In medicine, the instantaneous rate of change of blood volume in the heart chambers is related to cardiac output (the amount of blood pumped by the heart per minute).
Example: By monitoring the instantaneous rate of change of blood volume in the heart chambers, cardiologists can assess cardiac function and detect potential irregularities.
Calculating Instantaneous Rate of Change
While the intuitive understanding of the instantaneous rate of change as the slope of the tangent line is helpful, the actual calculation involves the concept of limits and derivatives.
For a function f(x), the instantaneous rate of change at a point x = a is given by the derivative:
f'(a) = lim (h→0) [f(a + h) - f(a)] / h
This formula represents the limit of the average rate of change as the interval 'h' approaches zero. In practice, for many common functions, derivative formulas are readily available, simplifying the calculation.
Conclusion
The instantaneous rate of change is a powerful concept with broad applications. From predicting population growth to monitoring the health of an economy or analyzing chemical reactions, understanding this concept is crucial across many disciplines. While the formal mathematical definition involves limits and derivatives, the intuitive understanding of it as the slope of the tangent line provides a solid foundation for grasping its significance and real-world applications. Mastering this concept opens doors to a deeper understanding of dynamic systems and their behavior.
Latest Posts
Latest Posts
-
Monomers Are Connected In What Type Of Reaction
Apr 02, 2025
-
Find The Equation Of The Vertical Line
Apr 02, 2025
-
Electron Configuration For Copper And Chromium
Apr 02, 2025
-
Confidence Interval Calculator With Two Samples
Apr 02, 2025
-
Lewis Base Vs Bronsted Lowry Base
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Examples Of Instantaneous Rate Of Change . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
