How Do I Multiply Rational Expressions
Muz Play
Apr 05, 2025 · 5 min read
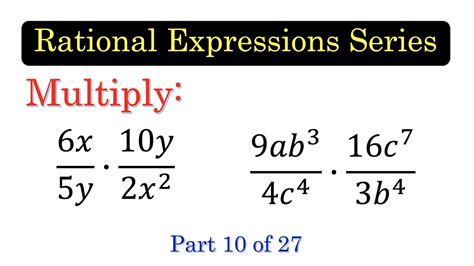
Table of Contents
How Do I Multiply Rational Expressions? A Comprehensive Guide
Multiplying rational expressions might seem daunting at first, but with a systematic approach and a solid understanding of the fundamentals, it becomes a manageable and even enjoyable algebraic process. This comprehensive guide will walk you through the steps, providing examples and tips to master this essential skill. We'll cover everything from simplifying individual expressions to tackling complex multiplications, ensuring you gain confidence in handling rational expressions.
Understanding Rational Expressions
Before diving into multiplication, let's solidify our understanding of what rational expressions are. A rational expression is simply a fraction where the numerator and/or the denominator are polynomials. Polynomials are algebraic expressions involving variables raised to non-negative integer powers, with coefficients that are constants.
For example:
(x² + 2x + 1) / (x + 1)is a rational expression.3x / (x² - 4)is a rational expression.5is also a rational expression (it can be written as 5/1).
The Fundamental Principle of Rational Expressions
The key to multiplying (and simplifying) rational expressions lies in the fundamental principle of fractions: you can multiply or divide both the numerator and the denominator of a fraction by the same non-zero value without changing the fraction's value. This principle is the backbone of simplifying rational expressions.
Step-by-Step Guide to Multiplying Rational Expressions
Multiplying rational expressions involves these key steps:
-
Factor Completely: This is arguably the most crucial step. Factor both the numerators and the denominators of all the rational expressions involved. Look for common factors, differences of squares (a² - b² = (a + b)(a - b)), perfect square trinomials (a² + 2ab + b² = (a + b)²), and other factoring techniques. The more completely you factor, the easier simplification will be.
-
Multiply Numerators and Denominators: Once everything is factored, multiply all the numerators together to form a new numerator and multiply all the denominators together to form a new denominator.
-
Cancel Common Factors: This is where the fundamental principle of fractions comes into play. Look for common factors in the numerator and the denominator of the resulting expression. Cancel these common factors – one factor from the numerator and one identical factor from the denominator. Remember, you can only cancel factors, not terms.
-
Write the Simplified Expression: After canceling all common factors, write the remaining expression. This is your simplified product of the rational expressions.
Examples: From Simple to Complex
Let's work through several examples to illustrate the process:
Example 1: Simple Multiplication
Multiply: (2x) / (x + 3) * (x + 3) / (4)
-
Factor: Both expressions are already factored.
-
Multiply:
(2x)(x + 3) / (x + 3)(4) -
Cancel: We can cancel the common factor
(x + 3)from the numerator and the denominator:(2x)(1) / (1)(4) -
Simplify:
2x / 4simplifies tox / 2
Example 2: Incorporating Factoring
Multiply: (x² - 4) / (x + 2) * (x + 1) / (x - 2)
-
Factor:
x² - 4is a difference of squares, so it factors to(x + 2)(x - 2). -
Multiply:
[(x + 2)(x - 2)] / (x + 2) * (x + 1) / (x - 2) -
Cancel: We can cancel
(x + 2)and(x - 2)from both the numerator and the denominator. Note that we're canceling factors, not terms. -
Simplify: The simplified expression is
x + 1.
Example 3: Dealing with More Complex Polynomials
Multiply: (x³ + 8) / (x² - 4) * (x² - x - 6) / (x² + 2x + 4)
-
Factor:
x³ + 8is a sum of cubes, factoring to(x + 2)(x² - 2x + 4)x² - 4is a difference of squares, factoring to(x + 2)(x - 2)x² - x - 6factors to(x - 3)(x + 2)
-
Multiply:
[(x + 2)(x² - 2x + 4)] / [(x + 2)(x - 2)] * [(x - 3)(x + 2)] / (x² + 2x + 4) -
Cancel: Cancel common factors:
(x + 2)from both numerator and denominator (twice). Note that(x² - 2x + 4)and(x² + 2x + 4)are not the same, so they cannot be canceled. -
Simplify: The simplified expression is
(x - 3) / (x - 2).
Example 4: Handling Expressions with Multiple Terms
Multiply: [(3x² + 6x) / (x² + x - 2)] * [(x² - 4x + 4) / (6x³)]
-
Factor:
- 3x² + 6x = 3x(x + 2)
- x² + x - 2 = (x + 2)(x - 1)
- x² - 4x + 4 = (x - 2)²
- 6x³ = 6x * x²
-
Multiply: [3x(x + 2) / ((x + 2)(x - 1))] * [(x - 2)² / (6x * x²)]
-
Cancel: Cancel the common factor (x + 2), x from both numerator and denominator.
-
Simplify: (x - 2)² / (2x(x - 1) ) = (x-2)²/ 2x(x-1)
Common Mistakes to Avoid
- Canceling Terms Instead of Factors: Remember, you can only cancel common factors, not terms. You cannot cancel x from (x + 2), even if it appears in each expression.
- Forgetting to Factor Completely: Incomplete factoring will lead to incorrect simplification. Always make sure you've factored each polynomial completely before canceling.
- Ignoring Restrictions: Rational expressions have restrictions on the values of the variables that make the denominator zero. These restrictions must be considered when simplifying and solving problems involving rational expressions. For example, in Example 2, x cannot be -2 or 2 because these values would make the denominator zero.
Mastering Rational Expressions: Practice Makes Perfect
The best way to master multiplying rational expressions is through consistent practice. Work through numerous examples, starting with simpler ones and gradually progressing to more challenging problems. Look for opportunities to apply these skills in other areas of algebra and precalculus. The more you practice, the more intuitive the process will become. Remember to always check your work, verifying your factoring and cancellation steps to avoid errors. With dedicated effort and attention to detail, you'll become proficient in multiplying and simplifying rational expressions.
Latest Posts
Latest Posts
-
What Is The Main Transformation That Occurs During Glycolysis
Apr 05, 2025
-
Why Does The Atomic Radii Increase Down A Group
Apr 05, 2025
-
Valves That Prevent Backflow Of Blood Into The Ventricles
Apr 05, 2025
-
What Is The Mass Of Neutron In Amu
Apr 05, 2025
-
What Causes Semilunar Valves To Open
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about How Do I Multiply Rational Expressions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
