How To Round Sig Figs When Adding
Muz Play
Mar 31, 2025 · 5 min read
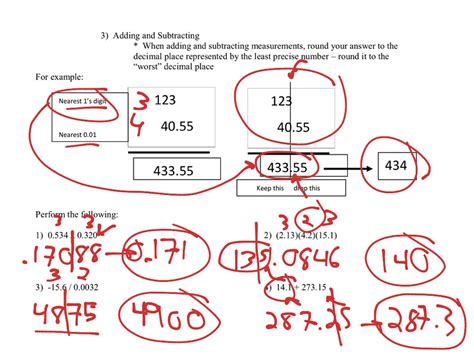
Table of Contents
How to Round Sig Figs When Adding: A Comprehensive Guide
Adding numbers and dealing with significant figures (sig figs) can be tricky. While multiplication and division have straightforward rules, addition and subtraction require a slightly different approach, focusing on the position of the last significant digit rather than the number of significant digits. This comprehensive guide will walk you through the process, explaining the rules, offering examples, and providing tips to avoid common mistakes. We’ll delve into the rationale behind these rules and equip you with the knowledge to confidently handle significant figure rounding in addition.
Understanding Significant Figures (Sig Figs)
Before diving into the rules for addition, let's quickly review what significant figures are. Significant figures represent the precision of a measurement. They include all the digits known with certainty plus one uncertain digit. For example:
- 25.3 cm: This measurement has three significant figures. The 2 and 5 are certain, while the 3 is the uncertain digit (estimated).
- 0.0045 g: This measurement has two significant figures (4 and 5). The leading zeros are not significant as they only serve to place the decimal point.
- 1200 kg: This is ambiguous. It could have two, three, or four significant figures depending on the precision of the measurement. Scientific notation clarifies this ambiguity (e.g., 1.2 x 10³ kg has two sig figs, 1.20 x 10³ kg has three).
The Rule for Adding with Significant Figures
The key to adding numbers and keeping track of sig figs lies in decimal place alignment:
The sum should be rounded to the same number of decimal places as the number with the fewest decimal places in the original numbers.
This means we don't count the total number of significant figures in each number. Instead, we focus on the position of the last significant digit relative to the decimal point.
Let's illustrate with examples:
Example 1: Simple Addition
Add the following measurements: 25.3 cm + 12.12 cm + 1.1 cm
-
Perform the addition: 25.3 cm + 12.12 cm + 1.1 cm = 38.52 cm
-
Identify the number with the fewest decimal places: 1.1 cm has only one decimal place.
-
Round the sum: The sum, 38.52 cm, needs to be rounded to one decimal place. Since the digit in the second decimal place (2) is less than 5, we round down.
-
Final answer: 38.5 cm
Example 2: Addition with Leading Zeros
Add: 0.0023 m + 0.125 m + 0.05 m
-
Perform the addition: 0.0023 m + 0.125 m + 0.05 m = 0.1773 m
-
Identify the number with the fewest decimal places: 0.05 m has two decimal places. Note: While 0.0023 only has two significant figures, it has four decimal places.
-
Round the sum: Round 0.1773 m to two decimal places. The digit in the third decimal place (7) is greater than or equal to 5, so we round up.
-
Final answer: 0.18 m
Example 3: Addition with Whole Numbers
Add: 125 g + 23 g + 1 g
-
Perform the addition: 125 g + 23 g + 1 g = 149 g
-
Identify the number with the fewest decimal places: All numbers have zero decimal places.
-
Round the sum: No rounding is needed as the sum already has zero decimal places.
-
Final answer: 149 g
Example 4: Handling Scientific Notation in Addition
Adding numbers in scientific notation requires careful attention to the exponents. Ensure that the numbers have the same exponent before performing the addition:
Add: 2.5 x 10² + 1.2 x 10¹
-
Convert to the same exponent: Rewrite 1.2 x 10¹ as 0.12 x 10².
-
Perform the addition: 2.5 x 10² + 0.12 x 10² = 2.62 x 10²
-
Identify the number with the fewest decimal places: Both have one decimal place after adjusting the exponent.
-
Round the sum: No further rounding is required.
-
Final answer: 2.62 x 10²
Common Mistakes to Avoid
- Focusing on the total number of sig figs: Remember, the rule for addition is about decimal places, not the total number of significant figures.
- Incorrectly rounding up or down: Follow the standard rounding rules (round up if the next digit is 5 or greater; round down if it's less than 5).
- Ignoring leading zeros: Leading zeros before a non-zero digit are not significant, but they still affect the number of decimal places in addition.
- Not adjusting exponents in scientific notation: Before adding numbers in scientific notation, ensure they have the same exponent.
The Importance of Precision in Calculations
The rules for significant figures in addition emphasize the importance of precision in scientific measurements and calculations. When we add measurements, the precision of the result is limited by the least precise measurement. Rounding according to the rules ensures that we don't falsely imply a higher level of precision than the data warrants.
For instance, if you are adding masses measured using a balance that only measures to the nearest gram (e.g. 1g, 2g, 3g) with masses measured using a more precise analytical balance that measures to the nearest milligram (e.g. 1.000g, 2.123g, 3.456g), the precision of the final sum is limited to the gram level. The more precise readings from the analytical balance contribute information but only to the extent allowed by the gram precision of the first instrument.
Advanced Considerations
While the decimal place rule generally suffices for most addition problems, there are nuances and exceptions, particularly in more complex calculations or when dealing with uncertainty analysis. These scenarios often require a deeper understanding of error propagation and statistical methods which goes beyond the scope of this basic introduction.
Conclusion
Mastering significant figure rounding in addition is a vital skill for anyone working with numerical data, especially in scientific fields. By understanding the principles outlined in this comprehensive guide and practicing the examples, you’ll enhance your ability to handle numerical data accurately and confidently communicate the precision of your results. Remember, the focus is on the decimal place, not the total number of significant figures, and always adhere strictly to the rules for rounding. Consistent application of these rules ensures that your calculations accurately reflect the precision of your measurements.
Latest Posts
Latest Posts
-
How Is The Use Of Symbols Related To Culture
Apr 01, 2025
-
As You Move Across The Periodic Table
Apr 01, 2025
-
What Is In The Atmosphere Of Jupiter
Apr 01, 2025
-
What Does A Negative Reduction Potential Mean
Apr 01, 2025
-
How To Calculate Standard Free Energy Change
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How To Round Sig Figs When Adding . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
