What Is The Coefficient Of Restitution
Muz Play
Mar 31, 2025 · 6 min read
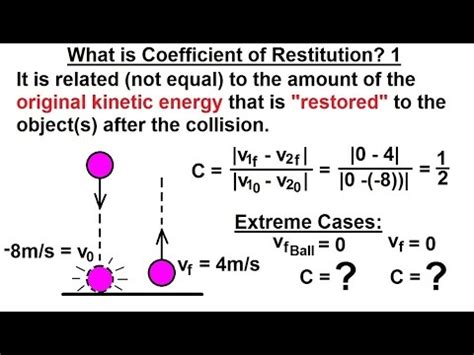
Table of Contents
What is the Coefficient of Restitution? A Deep Dive into Impact and Energy
The coefficient of restitution (COR), often denoted as 'e', is a crucial parameter in physics that quantifies the elasticity of a collision between two objects. It essentially measures how much kinetic energy is retained after an impact, providing insights into the nature of the collision and the properties of the colliding bodies. Understanding the COR is vital in diverse fields, from sports science and engineering to designing safety systems and analyzing impact events. This comprehensive guide will delve into the intricacies of the coefficient of restitution, exploring its definition, calculation, factors influencing it, and its applications across various disciplines.
Defining the Coefficient of Restitution
The coefficient of restitution is defined as the ratio of the relative velocity of separation to the relative velocity of approach between two objects after a collision. In simpler terms, it's a measure of how "bouncy" the collision is. A perfectly elastic collision, where no kinetic energy is lost, has a COR of 1 (e=1). A perfectly inelastic collision, where the objects stick together after impact, has a COR of 0 (e=0). Most real-world collisions fall somewhere in between these two extremes, with the COR representing the degree of energy loss during the collision.
Mathematically, the coefficient of restitution is expressed as:
e = -(v₂' - v₁') / (v₂ - v₁)
Where:
- v₁ and v₂ are the initial velocities of object 1 and object 2, respectively, before the collision.
- v₁' and v₂' are the final velocities of object 1 and object 2, respectively, after the collision.
Understanding Different Types of Collisions
The value of the coefficient of restitution directly reflects the type of collision:
1. Perfectly Elastic Collision (e = 1):
In a perfectly elastic collision, kinetic energy is conserved. The total kinetic energy of the system before the collision equals the total kinetic energy after the collision. This ideal scenario rarely occurs in the real world due to energy losses through heat, sound, and deformation. Examples that approach perfect elasticity include collisions between very hard, smooth objects like billiard balls (though even here, some energy loss occurs).
2. Perfectly Inelastic Collision (e = 0):
In a perfectly inelastic collision, the maximum possible kinetic energy is lost. The colliding objects stick together after impact, moving with a common final velocity. Examples include a clay ball hitting a wall or a car crash where the vehicles become entangled.
3. Inelastic Collision (0 < e < 1):
This is the most common type of collision in the real world. Some kinetic energy is lost during the collision, but the objects do not stick together. The amount of energy lost depends on the coefficient of restitution and the properties of the colliding objects. Examples include a basketball bouncing on the floor or a tennis ball hitting a racket.
Factors Affecting the Coefficient of Restitution
Several factors influence the coefficient of restitution, making it a complex parameter to predict precisely. These include:
-
Materials of the colliding objects: The material properties, such as hardness, elasticity, and internal structure, significantly impact how much energy is absorbed during the collision. Harder, more elastic materials tend to have higher COR values.
-
Temperature: Temperature affects the material properties, particularly elasticity. Higher temperatures can lead to slightly lower COR values in some materials.
-
Velocity of impact: The speed at which the objects collide can influence the COR. At very high impact velocities, plastic deformation may become more significant, resulting in a lower COR.
-
Surface conditions: The roughness and smoothness of the colliding surfaces affect the contact area and friction during the collision, thus influencing energy loss and the COR. Rougher surfaces typically have lower COR values.
-
Deformation: Permanent deformation of the colliding objects leads to significant energy loss and a lower COR.
-
Impact Angle: The angle of impact can also influence the COR. A glancing blow might result in a different COR than a direct, head-on collision.
Calculating the Coefficient of Restitution: Practical Applications
Calculating the COR experimentally involves measuring the velocities of the objects before and after the collision. High-speed cameras are frequently employed to accurately capture these velocities. Alternatively, various indirect methods can be used depending on the system being studied.
Examples of Applications:
-
Sports: The COR is a vital factor in sports equipment design and performance analysis. For example, the bounce height of a basketball depends on its COR and the floor's material. In golf, the COR of the golf ball influences its distance and trajectory. Similarly, in tennis, the COR between the ball and racket affects spin and velocity.
-
Automotive Engineering: The COR is essential in designing safer vehicles. Crash tests involve measuring the COR to assess the energy absorption capacity of different car parts and materials, improving vehicle safety designs.
-
Impact Mechanics: Understanding the COR is vital in impact mechanics, analyzing the effects of collisions on structures and materials. This is crucial in fields like aerospace engineering and structural analysis, designing for impacts from debris or other objects.
-
Robotics: In robotics, the COR plays a significant role in designing robot manipulators and grasping systems. The interactions between the robot and its environment often involve collisions, so understanding the COR helps in developing more efficient and robust systems.
-
Geophysics: The COR can be applied to study seismic waves. The reflection and refraction of waves depend on the properties of the materials involved, including their COR.
-
Manufacturing: In industrial processes involving impacts, the COR helps optimize the design and operation of machinery. For example, in forging or stamping, the COR will help in predicting energy transfer and material deformation.
Advanced Concepts and Considerations
The coefficient of restitution is a simplified model that assumes certain idealizations. In reality, collisions are complex phenomena influenced by factors beyond the simple relative velocity approach. These include:
-
Non-linear material behavior: Many materials do not exhibit linear elastic behavior, especially at high impact velocities. This complicates the COR calculation and makes it dependent on impact velocity.
-
Friction: Frictional forces during the collision dissipate energy, which is not always directly accounted for in the basic COR equation.
-
Energy dissipation mechanisms: Energy is lost during impact through various mechanisms, including heat generation, sound production, and plastic deformation. These losses are not always easily quantifiable.
-
Multiple impacts: In scenarios with multiple impacts, the COR becomes more intricate to determine, requiring complex simulations.
Conclusion
The coefficient of restitution is a fundamental concept in physics, crucial for understanding and predicting the outcome of collisions. Its application spans a wide range of fields, from sports science and engineering to geophysics and robotics. While the simple definition and equation provide a useful approximation, it's important to remember that the COR is influenced by numerous factors, and its precise determination often requires advanced techniques and considerations. By understanding these complexities and employing appropriate methodologies, we can accurately leverage the COR to solve numerous practical problems and design more efficient and robust systems. Continued research and refinement of the COR model will further enhance its accuracy and expand its applicability across diverse scientific and engineering domains.
Latest Posts
Latest Posts
-
How To Find Moles Of Naoh Used In Titration
Apr 02, 2025
-
Different Conformations Of The Same Compound
Apr 02, 2025
-
Induced Fit Model Vs Lock And Key
Apr 02, 2025
-
Colligative Properties Freezing Point Depression Lab
Apr 02, 2025
-
Matrix As A Product Of Elementary Matrices
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Coefficient Of Restitution . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
