What Is The Unit Of Measure For Kinetic Energy
Muz Play
Mar 28, 2025 · 5 min read
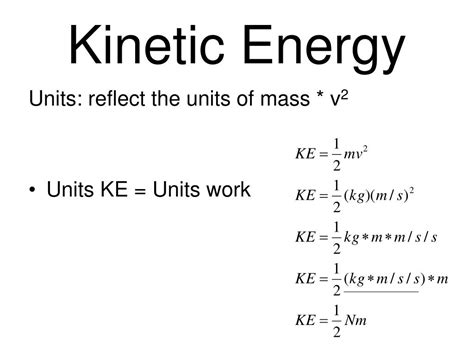
Table of Contents
What is the Unit of Measure for Kinetic Energy? A Deep Dive
Kinetic energy, the energy possessed by an object due to its motion, is a fundamental concept in physics. Understanding its unit of measure is crucial for accurately calculating and applying this important principle in various fields, from mechanics to thermodynamics. This comprehensive guide delves into the unit of kinetic energy, its derivation, and its applications across different systems of units. We'll also explore related concepts and address common misconceptions.
Understanding Kinetic Energy
Before diving into the unit of measure, let's refresh our understanding of kinetic energy itself. Kinetic energy is directly proportional to the mass of the object and the square of its velocity. This relationship is expressed by the following equation:
KE = ½mv²
Where:
- KE represents kinetic energy
- m represents the mass of the object
- v represents the velocity of the object
This equation tells us that a heavier object moving at the same speed as a lighter object will possess more kinetic energy. Similarly, an object moving at a higher speed will have significantly more kinetic energy than the same object moving at a lower speed (due to the velocity being squared).
The Unit of Measure for Kinetic Energy: Joules (J)
In the International System of Units (SI), the standard unit of kinetic energy, and energy in general, is the joule (J). The joule is a derived unit, meaning it's defined in terms of other fundamental SI units. To understand why the joule is the unit for kinetic energy, let's break down the units of mass and velocity in the kinetic energy equation:
- Mass (m): Measured in kilograms (kg) in the SI system.
- Velocity (v): Measured in meters per second (m/s) in the SI system.
Substituting these units into the kinetic energy equation:
KE = ½ * (kg) * (m/s)² = kg⋅m²/s²
Therefore, 1 joule is equivalent to 1 kilogram-meter squared per second squared (kg⋅m²/s²). This reflects the fundamental relationship between mass, distance, and time in defining energy.
Kinetic Energy in Different Unit Systems
While the joule is the standard and most widely used unit for kinetic energy, other unit systems exist, each with its own equivalent unit. Let's explore some of them:
1. CGS System (centimeter-gram-second)
In the CGS system, the unit of mass is the gram (g), and the unit of velocity is centimeters per second (cm/s). Therefore, the unit of kinetic energy in the CGS system becomes:
KE = ½ * (g) * (cm/s)² = g⋅cm²/s²
This unit is called an erg. One erg is equal to 10⁻⁷ joules.
2. British Imperial/US Customary Units
This system uses pounds (lb) for mass, feet (ft) for distance, and seconds (s) for time. The resulting unit of kinetic energy is:
KE = ½ * (lb) * (ft/s)² = lb⋅ft²/s²
This unit doesn't have a specific name but is often expressed as foot-pounds (ft⋅lb) which is also known as foot-pound-force (lbf⋅ft). Conversion to joules involves using conversion factors for pounds and feet to kilograms and meters, respectively.
3. Electronvolt (eV)
In atomic and nuclear physics, a much smaller unit of energy is often used: the electronvolt (eV). This represents the energy gained by a single electron when accelerated through a potential difference of one volt. It's a convenient unit for describing the kinetic energies of subatomic particles. Conversion to joules is done through the following relationship: 1 eV = 1.602 × 10⁻¹⁹ J.
Practical Applications and Examples
Understanding the unit of kinetic energy is crucial for various applications, including:
- Mechanical Engineering: Calculating the energy transferred during collisions, designing machines and structures that withstand impact forces, analyzing the efficiency of mechanical systems.
- Automotive Engineering: Determining the braking distances of vehicles, designing safety features like airbags, calculating the energy absorbed during crash tests.
- Aerospace Engineering: Analyzing the energy of projectiles, calculating the fuel efficiency of rockets, designing aircraft wings to withstand air pressure.
- Sports Science: Analyzing the impact forces in sports, optimizing athletes' performance through energy transfer analysis.
- Physics Research: In various branches of physics, from particle physics to astrophysics, kinetic energy is a crucial element in various calculations and analyses.
Example 1: A 1 kg ball is thrown at a speed of 10 m/s. Calculate its kinetic energy.
KE = ½ * (1 kg) * (10 m/s)² = 50 kg⋅m²/s² = 50 J
Example 2: A car with a mass of 1000 kg is traveling at 20 m/s. What is its kinetic energy?
KE = ½ * (1000 kg) * (20 m/s)² = 200,000 J or 200 kJ
Common Misconceptions and Clarifications
Several common misconceptions exist regarding kinetic energy and its unit:
- Confusing kinetic energy with momentum: While both depend on mass and velocity, kinetic energy is proportional to the square of the velocity, while momentum is directly proportional to the velocity. They have different units and represent different physical quantities.
- Neglecting the direction of velocity: Kinetic energy is a scalar quantity; it only has magnitude and no direction. Unlike momentum, which is a vector quantity and has both magnitude and direction.
- Assuming constant velocity: The kinetic energy of an object changes when its velocity changes. Calculations should reflect this change.
Conclusion: Mastering the Unit of Kinetic Energy
The joule (J), or its equivalents in other unit systems, is the fundamental unit for measuring kinetic energy. A thorough understanding of this unit, its derivation from fundamental units, and its applications across various disciplines is critical for accurate calculations and effective problem-solving in numerous fields. By grasping the relationship between mass, velocity, and kinetic energy, and by understanding the nuances of different unit systems, engineers, scientists, and students can confidently tackle complex problems involving motion and energy. Remembering the fundamental equation, KE = ½mv², and the importance of using consistent units, is key to achieving accuracy and success in any kinetic energy calculation. Moreover, understanding the conceptual differences between kinetic energy and related concepts such as momentum is crucial for avoiding common misconceptions and building a robust understanding of the principles of mechanics and energy.
Latest Posts
Latest Posts
-
Which Statement Describes The Citric Acid Cycle
Mar 31, 2025
-
Why Do Plants Love Water In Bio Terms
Mar 31, 2025
-
Identifying The Important Intermolecular Forces In Pure Compounds
Mar 31, 2025
-
Why Does Km Increase In Competitive Inhibition
Mar 31, 2025
-
What Is The Electron Configuration Of Beryllium
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is The Unit Of Measure For Kinetic Energy . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
