What Is The Absolute Value Of -7
Muz Play
Mar 28, 2025 · 6 min read
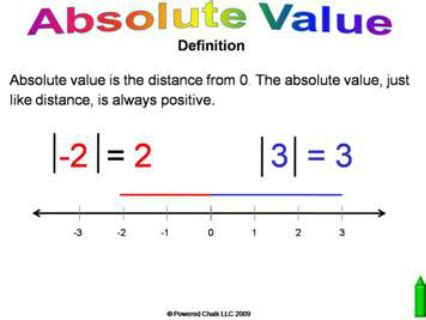
Table of Contents
What is the Absolute Value of -7? A Deep Dive into Absolute Value
The seemingly simple question, "What is the absolute value of -7?" opens the door to a fascinating exploration of a fundamental concept in mathematics: absolute value. While the answer itself is straightforward (7), understanding the why behind it unlocks a deeper appreciation for its significance in various mathematical fields and real-world applications. This comprehensive guide will not only answer the question but delve into the intricacies of absolute value, exploring its definition, properties, graphical representation, and applications.
Understanding Absolute Value: More Than Just Removing the Negative Sign
The absolute value of a number is its distance from zero on the number line. This definition is crucial because it emphasizes the non-directional nature of absolute value. Distance is always positive; you can't have a negative distance. Therefore, the absolute value of a number is always non-negative (positive or zero).
The absolute value of -7 is 7, because -7 is 7 units away from zero on the number line. Similarly, the absolute value of 7 is also 7, as it is also 7 units away from zero.
We denote the absolute value of a number x using vertical bars: |x|. So, |-7| = 7 and |7| = 7.
Defining Absolute Value Formally
Mathematically, we can define the absolute value function as follows:
|x| = { x, if x ≥ 0; -x, if x < 0 }
This piecewise function states that:
- If x is greater than or equal to zero (non-negative), the absolute value of x is simply x itself.
- If x is less than zero (negative), the absolute value of x is the negative of x (which makes it positive).
This definition perfectly captures the idea of distance from zero. When dealing with a negative number, negating it makes it positive, representing the distance from zero.
Properties of Absolute Value
Understanding the properties of absolute value is essential for effectively manipulating expressions and solving equations involving absolute value. These properties include:
- Non-negativity: |x| ≥ 0 for all real numbers x.
- Identity: |x| = 0 if and only if x = 0.
- Evenness: |-x| = |x| for all real numbers x. The absolute value function is an even function, symmetric about the y-axis.
- Multiplicative property: |xy| = |x||y| for all real numbers x and y. The absolute value of a product is the product of the absolute values.
- Triangle inequality: |x + y| ≤ |x| + |y| for all real numbers x and y. This inequality has significant implications in various mathematical areas.
Graphical Representation of the Absolute Value Function
The graph of the absolute value function, y = |x|, is a V-shaped graph with its vertex at the origin (0,0). The left branch of the V represents the portion of the function where x < 0, and the right branch represents the portion where x ≥ 0. The slope of the left branch is -1, and the slope of the right branch is 1.
Visualizing |-7| on the Graph
To visualize |-7| on the graph of y = |x|, we would find the point on the graph where x = -7. Since the function is defined as y = -x for x < 0, the y-coordinate at x = -7 is -(-7) = 7. This confirms that |-7| = 7.
Solving Equations and Inequalities Involving Absolute Value
Absolute value equations and inequalities require careful consideration of both positive and negative cases due to the nature of the absolute value function. Here's a brief overview:
Solving equations:
An equation of the form |x| = a (where a ≥ 0) has two solutions: x = a and x = -a. For example, if |x| = 5, then x = 5 or x = -5.
Solving inequalities:
Inequalities involving absolute value are solved differently depending on the inequality symbol:
- |x| < a: This inequality is equivalent to -a < x < a. The solution is an interval.
- |x| > a: This inequality is equivalent to x < -a or x > a. The solution consists of two disjoint intervals.
These techniques are extended to more complex equations and inequalities using algebraic manipulation and the properties of absolute value.
Real-World Applications of Absolute Value
Absolute value is not just a theoretical concept; it has numerous applications in various fields:
-
Distance calculations: The absolute value is crucial in calculating distances between points, whether in one dimension (on a number line) or higher dimensions (in coordinate systems). Distance is always a non-negative quantity.
-
Error analysis: In engineering and scientific fields, absolute value is used to represent the magnitude of errors or deviations from expected values. The absolute error is the absolute difference between the measured value and the true value.
-
Programming and computer science: Absolute value functions are built-in functions in most programming languages and are frequently used in algorithms and computations.
-
Physics and engineering: Absolute value finds use in various physics equations, particularly those involving vectors and magnitudes.
-
Economics and finance: Absolute value is applied in calculating deviations from expected values in financial modeling and forecasting.
-
Statistics: Absolute value plays a role in calculating measures of dispersion, such as mean absolute deviation, a measure of the average distance of data points from the mean.
Beyond the Basics: Advanced Concepts
For those seeking a deeper understanding, there are more advanced concepts related to absolute value:
-
Absolute value inequalities involving multiple variables: Solving inequalities with absolute values of expressions containing multiple variables requires careful consideration of various cases and systematic approaches.
-
Absolute value functions in calculus: Analyzing the derivative and integral of absolute value functions requires specialized techniques due to the non-differentiability at the vertex.
-
Absolute value in linear algebra: Absolute value concepts extend to vector spaces, where the absolute value generalizes to the concept of a norm (a measure of vector length).
-
Complex numbers and absolute value: The concept of absolute value (or modulus) is extended to complex numbers, representing the distance of a complex number from the origin in the complex plane.
Conclusion: The Significance of a Simple Concept
While the absolute value of -7 is simply 7, the underlying concept and its properties are far-reaching and profoundly impactful across numerous mathematical disciplines and real-world applications. Understanding absolute value is fundamental to mastering various mathematical techniques and interpreting results in diverse fields. This exploration goes beyond a simple answer; it highlights the significance of a seemingly basic concept in shaping our understanding of mathematics and its applications. The seemingly simple question has led us on a journey through various aspects of mathematics, demonstrating that even fundamental concepts hold rich complexities and far-reaching implications. This journey highlights the beauty of mathematics: the interconnectedness of its concepts and their surprising relevance to the world around us.
Latest Posts
Latest Posts
-
Why Do Plants Love Water In Bio Terms
Mar 31, 2025
-
Identifying The Important Intermolecular Forces In Pure Compounds
Mar 31, 2025
-
Why Does Km Increase In Competitive Inhibition
Mar 31, 2025
-
What Is The Electron Configuration Of Beryllium
Mar 31, 2025
-
When Do You Consider Log Diterminants Similar
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is The Absolute Value Of -7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
