What Is The Density Of The Gas
Muz Play
Mar 29, 2025 · 7 min read
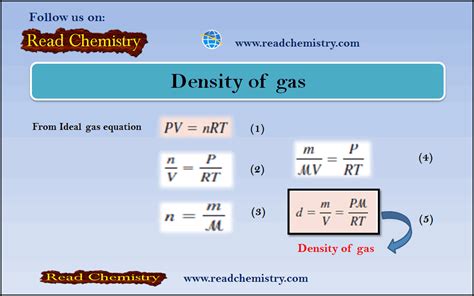
Table of Contents
What is the Density of a Gas? Understanding Gas Density and its Applications
Gas density, a fundamental concept in physics and chemistry, refers to the mass of a gas per unit volume. Unlike solids and liquids, gases are highly compressible, meaning their density can change significantly with variations in pressure and temperature. Understanding gas density is crucial across various scientific disciplines and industrial applications. This comprehensive guide will delve into the intricacies of gas density, exploring its definition, influencing factors, calculation methods, and real-world applications.
Defining Gas Density
Gas density is typically represented by the Greek letter ρ (rho) and is defined as:
ρ = m/V
where:
- ρ represents density (usually measured in kg/m³ or g/L)
- m represents mass (usually measured in kilograms or grams)
- V represents volume (usually measured in cubic meters or liters)
Unlike solids and liquids where density remains relatively constant under normal conditions, gas density is highly variable and depends heavily on the pressure and temperature of the gas. This dependence stems from the kinetic theory of gases, which describes gases as collections of particles in constant, random motion. Changes in pressure and temperature directly affect the average kinetic energy of these particles and, consequently, the volume they occupy.
Factors Affecting Gas Density
Several key factors influence the density of a gas:
1. Pressure
Increasing the pressure on a gas forces its molecules closer together, resulting in a higher density. This is because the same mass of gas now occupies a smaller volume. Conversely, decreasing the pressure allows the gas molecules to spread out, leading to a lower density. This relationship is often described by Boyle's Law, which states that at constant temperature, the pressure of a gas is inversely proportional to its volume (P ∝ 1/V).
2. Temperature
Increasing the temperature of a gas increases the kinetic energy of its molecules, causing them to move faster and collide more frequently. This leads to an expansion in volume, resulting in a lower density. Conversely, decreasing the temperature reduces molecular kinetic energy, causing the gas to contract and resulting in a higher density. This relationship is described by Charles's Law, which states that at constant pressure, the volume of a gas is directly proportional to its absolute temperature (V ∝ T).
3. Molecular Weight (Molar Mass)
The molecular weight or molar mass of a gas significantly influences its density. Heavier gas molecules (higher molar mass) will result in a higher density at the same temperature and pressure compared to lighter gas molecules (lower molar mass). This is because a given volume will contain fewer heavier molecules than lighter molecules, yet the total mass remains higher.
4. Humidity (for Moist Air)
In the case of moist air, the presence of water vapor affects the overall density. Since water vapor has a lower molar mass than dry air components (nitrogen and oxygen), the presence of water vapor generally decreases the density of moist air compared to dry air at the same temperature and pressure. This effect is especially noticeable at higher temperatures and humidities.
Calculating Gas Density
The density of a gas can be calculated using various methods, depending on the available information.
1. Using the Ideal Gas Law
The ideal gas law, PV = nRT, provides a powerful tool for calculating gas density. The ideal gas law relates pressure (P), volume (V), number of moles (n), gas constant (R), and temperature (T). We can rearrange this equation to express density:
ρ = (PM)/(RT)
Where:
- ρ is the density
- P is the pressure
- M is the molar mass of the gas
- R is the ideal gas constant (8.314 J/mol·K or 0.0821 L·atm/mol·K)
- T is the absolute temperature (in Kelvin)
This equation is particularly useful when dealing with ideal gases, which behave according to the ideal gas law. However, it's important to remember that real gases deviate from ideal behavior at high pressures and low temperatures.
2. Using the Density of Dry Air
The density of dry air at standard temperature and pressure (STP, 0°C and 1 atm) is approximately 1.29 kg/m³. This value can serve as a reference point for comparing the densities of other gases. However, it's crucial to remember that this density varies with changes in temperature and pressure, as discussed earlier.
3. Experimental Determination
Gas density can also be determined experimentally through careful measurement of the mass and volume of a gas sample. This involves techniques like using a gas collection apparatus and precise weighing scales. The accuracy of this method depends on the precision of the measurement instruments and the control of experimental conditions (temperature and pressure).
Applications of Gas Density
Understanding and calculating gas density is crucial across many fields:
1. Meteorology and Atmospheric Science
Gas density plays a vital role in understanding atmospheric phenomena. Variations in air density, influenced by temperature, pressure, and humidity, drive wind patterns, weather systems, and atmospheric stability. Density differences between air masses contribute to the formation of clouds, precipitation, and other weather events. Understanding atmospheric density is critical for weather forecasting and climate modeling.
2. Aviation and Aerospace Engineering
Aircraft design and flight performance are significantly influenced by air density. Higher air density at lower altitudes results in increased lift and drag on aircraft. Pilots and aerospace engineers use density altitude (the altitude in the standard atmosphere at which the air density is equal to the density at a given location) to adjust flight parameters and ensure safe and efficient operations.
3. Industrial Processes
Many industrial processes involve gases, and controlling their density is crucial for efficient operation and product quality. For example, the density of gases used in combustion processes affects the efficiency of energy production. Similarly, the density of gases in chemical reactors impacts reaction rates and product yields. Industries like food processing, pharmaceuticals, and petrochemicals all utilize density measurements for quality control and process optimization.
4. Environmental Monitoring
Measuring gas density is important for environmental monitoring. Monitoring the density of pollutants in the atmosphere helps determine the severity of air pollution and assess the effectiveness of pollution control measures. Measuring the density of greenhouse gases contributes to climate change research and policy development.
5. Medical Applications
Gas density plays a role in respiratory medicine. Measuring the density of lung tissue using techniques like computed tomography (CT) scans provides information about lung health and can help diagnose lung diseases.
6. Scientific Research
Gas density measurements are essential for various scientific research applications, including:
- Physical chemistry: Studying the behavior of gases under different conditions.
- Chemical engineering: Designing and optimizing chemical processes involving gases.
- Materials science: Investigating the properties of materials in gaseous environments.
- Environmental science: Monitoring the levels of atmospheric pollutants.
Understanding Real Gases vs. Ideal Gases
The ideal gas law provides a simplified model for gas behavior. However, real gases deviate from ideal behavior, particularly at high pressures and low temperatures. These deviations occur because the ideal gas law neglects intermolecular forces and the finite volume of gas molecules.
Real gas equations, such as the van der Waals equation, provide more accurate predictions of gas density by incorporating these factors. The van der Waals equation introduces two correction factors:
- a: Accounts for intermolecular attractive forces.
- b: Accounts for the finite volume of gas molecules.
While the ideal gas law is a useful approximation in many situations, understanding the limitations and using real gas equations when necessary is crucial for accurate calculations and interpretations, especially when dealing with gases under extreme conditions.
Conclusion
Gas density is a fundamental concept with far-reaching applications across various scientific and industrial fields. Its dependence on pressure, temperature, and molecular weight necessitates careful consideration when calculating and using it. While the ideal gas law offers a convenient approximation, understanding the deviations of real gases from ideal behavior is crucial for accurate and reliable results. Continued research and advancements in measurement techniques will further refine our understanding and application of gas density in diverse contexts. Further exploration into specific gas densities (e.g., the density of hydrogen, the density of helium, the density of carbon dioxide) can be beneficial to those in fields directly utilizing this knowledge. Understanding gas density is not just a theoretical exercise; it's a practical tool with significant implications for scientific advancement and technological progress.
Latest Posts
Latest Posts
-
Raising And Lowering Operators Angular Momentum
Mar 31, 2025
-
Unauthrized Data Rollbak Or Undo Cia Triad
Mar 31, 2025
-
Titration Curve Of Naoh And Hcl
Mar 31, 2025
-
If Keq Is Greater Than 1
Mar 31, 2025
-
Difference Between Density Dependent And Density Independent
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is The Density Of The Gas . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
